EasyElearn - Maths Tuition Singapore - Secondary 1 - HCF and LCM
Summary
TLDRIn this lesson, we explore prime factorization, the highest common factor (HCF), and the lowest common multiple (LCM). Prime factorization involves breaking down a number into its prime factors. The HCF is found by identifying the smallest powers of common prime factors, while the LCM is determined by selecting the largest powers. Through examples like 45, 12, and 18, the video demonstrates how to apply these concepts effectively. By using prime factorization, viewers can calculate the HCF and LCM with ease, helping them understand these key mathematical operations.
Takeaways
- 😀 Prime numbers have only two factors: 1 and the number itself.
- 😀 Prime factorization involves expressing a number as a product of prime numbers.
- 😀 To perform prime factorization of 45, divide by prime numbers starting from 3: 45 → 3 × 3 × 5.
- 😀 The highest common factor (HCF) is found by identifying the smallest powers of common prime factors.
- 😀 The lowest common multiple (LCM) is found by identifying the largest powers of all prime factors involved.
- 😀 For example, the HCF of 12 and 18 is 6 (2^1 × 3^1), while the LCM is 36 (2^2 × 3^2).
- 😀 To find the HCF, take the smallest powers of each common prime factor.
- 😀 To find the LCM, take the largest powers of each prime factor across the numbers involved.
- 😀 In the case of 12, its prime factorization is 2^2 × 3, and for 18, it's 2 × 3^2.
- 😀 The process of prime factorization helps in determining both the HCF and LCM accurately.
Q & A
What is a prime number?
-A prime number is a number that has only two factors: 1 and the number itself.
What does prime factorization mean?
-Prime factorization is the process of writing a number as a product of prime numbers.
How do you perform prime factorization on the number 45?
-To factorize 45, divide by prime numbers: 45 ÷ 3 = 15, 15 ÷ 3 = 5, and 5 ÷ 5 = 1. The prime factorization of 45 is 3^2 × 5.
What is the Highest Common Factor (HCF)?
-The HCF is the greatest number that divides two or more numbers exactly, which is found by taking the smallest powers of the common prime factors.
How do you find the HCF of 12 and 18?
-First, prime factorize both numbers: 12 = 2^2 × 3 and 18 = 2 × 3^2. The smallest powers of the common prime factors are 2^1 and 3^1. Therefore, the HCF of 12 and 18 is 6.
What is the Lowest Common Multiple (LCM)?
-The LCM is the smallest multiple that two or more numbers share, found by taking the largest powers of all the prime factors involved.
How do you find the LCM of 12 and 18?
-To find the LCM of 12 and 18, prime factorize both numbers: 12 = 2^2 × 3 and 18 = 2 × 3^2. The largest powers of the primes are 2^2 and 3^2. Therefore, the LCM of 12 and 18 is 36.
What is the method for finding the HCF using prime factorization?
-To find the HCF, list the prime factors of both numbers, then choose the smallest power of each common prime factor.
Why do we use prime factorization when finding the HCF and LCM?
-Prime factorization helps break down the numbers into their simplest prime components, making it easier to find the smallest or largest powers for calculating the HCF and LCM.
What is the significance of writing 3^2 × 5 instead of just 3 × 3 × 5?
-Writing 3^2 × 5 simplifies the expression and makes it clear that there are two 3's involved in the prime factorization of 45, which is more concise and easier to understand.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示
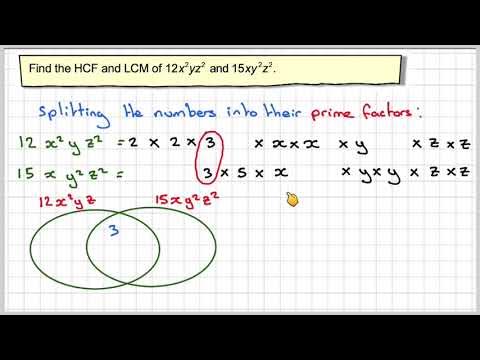
Finding the HCF and LCM of algebraic expressions

minimo comune multiplo (mcm) e Massimo Comun Divisore (MCD): come calcolarli senza confonderli
How to Find the LCM (2 Different Ways) | Least Common Multiple | Math with Mr. J
How to Find the LCM using Prime Factorization | Least Common Multiple | Math with Mr. J
How To Find The LCM of 3 Numbers - Plenty of Examples!

Discrete Math - 4.3.2 Greatest Common Divisors and Least Common Multiples
5.0 / 5 (0 votes)
