Résoudre un problème à l'aide du théorème de Pythagore - Quatrième
Summary
TLDRIn this video, the Pythagorean Theorem is applied to solve a practical problem involving the height of a roof in René's house. The base of the roof is 9 meters, and each slant height measures 5.6 meters. By breaking the roof into two right triangles, the height of the attic is calculated step-by-step using the theorem. The video explains how to apply the formula, identify the correct triangle, and solve the equation to find the unknown height, which turns out to be approximately 3.33 meters. The process is clear, concise, and easy to follow.
Takeaways
- 😀 The Pythagorean Theorem is used to solve problems involving right triangles.
- 😀 The script focuses on calculating the height of an attic in René's house using the Pythagorean Theorem.
- 😀 The base of the roof (AB) is 9 meters, and the length of each sloping side is 5.6 meters.
- 😀 The height of the attic (SH) needs to be calculated using the triangle formed by the roof.
- 😀 The triangle is identified as a right triangle due to the right angle at the foot of the height.
- 😀 The base of the roof (AB) is split into two equal parts, each measuring 4.5 meters.
- 😀 The Pythagorean Theorem formula used is: Hypotenuse² = Side1² + Side2².
- 😀 The Pythagorean Theorem is applied to the left triangle (ASH) to calculate the height.
- 😀 The calculation involves squaring the known values and solving for the unknown side SH.
- 😀 The final result for the height of the attic (SH) is approximately 3.33 meters, or 3 meters 33 centimeters.
Q & A
What is the problem described in the video?
-The video describes a problem where the goal is to calculate the height of René's attic using the Pythagorean theorem. The base of the roof (AB) is 9 meters, and each side of the roof (AS and BS) measures 5.6 meters.
What theorem is used to solve this problem?
-The Pythagorean theorem is used to solve this problem, which relates the sides of a right-angled triangle. Specifically, it states that the square of the hypotenuse is equal to the sum of the squares of the other two sides.
What does the Pythagorean theorem state?
-The Pythagorean theorem states that the square of the hypotenuse (the longest side of a right triangle) is equal to the sum of the squares of the other two sides. This relationship allows you to find one side if the other two are known.
How is the height of the attic calculated?
-To calculate the height of the attic, the Pythagorean theorem is applied to a right-angled triangle where the hypotenuse (AS) is known (5.6 meters) and one leg (AH) is half of AB (4.5 meters). By solving the equation, the height (SH) is found to be approximately 3.33 meters.
How is the length AH calculated?
-The length AH is calculated by dividing the length of AB by 2. Since AB is 9 meters, AH equals 9 / 2, which is 4.5 meters.
Why is the Pythagorean theorem applicable to this problem?
-The Pythagorean theorem is applicable because the problem involves a right-angled triangle (ASH), where the base (AH) and hypotenuse (AS) are known, and the height (SH) is the unknown value to be calculated.
What steps are involved in solving for SH using the Pythagorean theorem?
-To solve for SH, we start by using the Pythagorean theorem formula: AS² = AH² + SH². We then substitute the known values: 5.6² = 4.5² + SH². By calculating the squares and solving for SH, we find SH ≈ 3.33 meters.
What is the result of the calculation when applying the Pythagorean theorem?
-After applying the Pythagorean theorem and performing the necessary calculations, the result is SH² = 11.11, and taking the square root gives SH ≈ 3.33 meters, or 3 meters 33 centimeters.
How are the square values calculated during the solution process?
-The square values are calculated as follows: 5.6² = 31.36, 4.5² = 20.25. These values are substituted into the equation, and the difference between 31.36 and 20.25 is used to find SH², which is 11.11.
What is the final height of René's attic?
-The final height of René's attic is approximately 3.33 meters, or 3 meters and 33 centimeters.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Using math in the real world - Pythagorean theorem
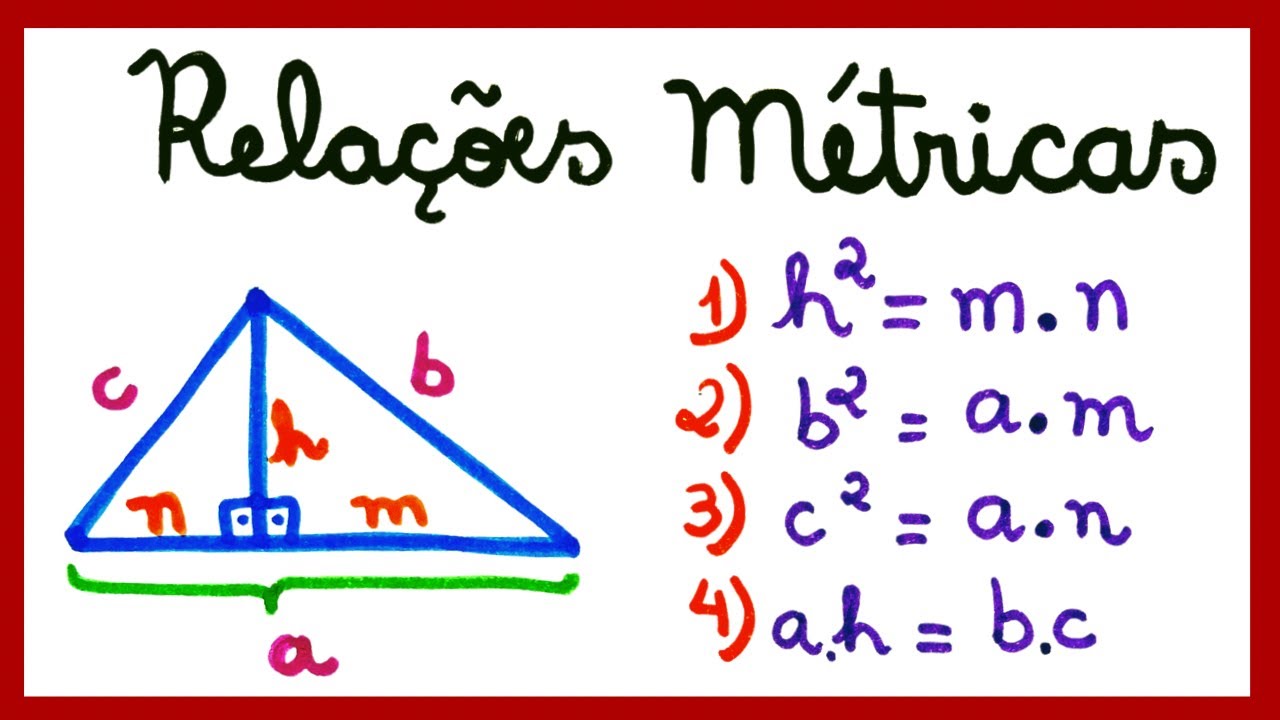
RELAÇÕES MÉTRICAS NO TRIÂNGULO RETÂNGULO!!
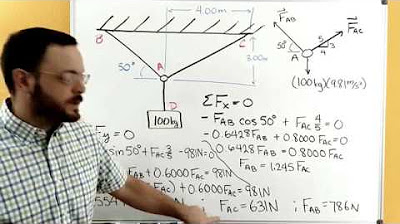
Statics - 2D equilibrium - particle problems - example

Pythagoras (1) - Pengenalan Teorema Pythagoras, Pythagoras Theorem - Matematika SMP
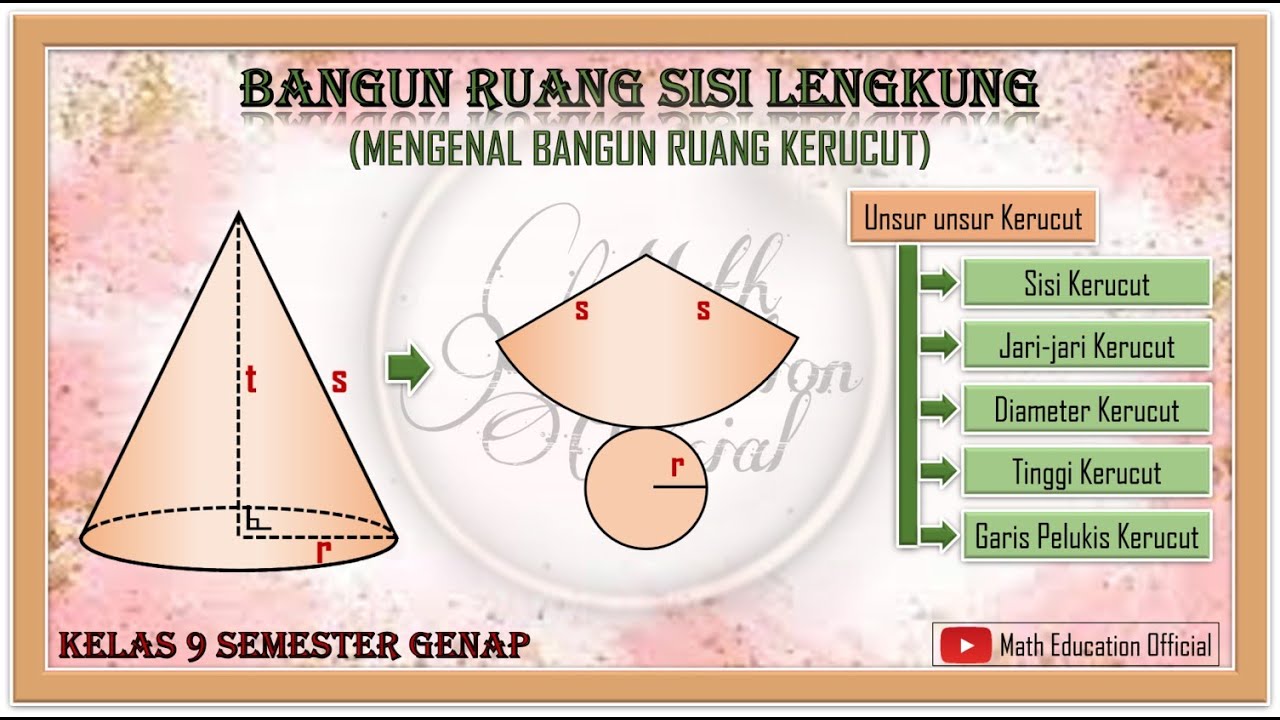
Mengenal Bangun Ruang Kerucut
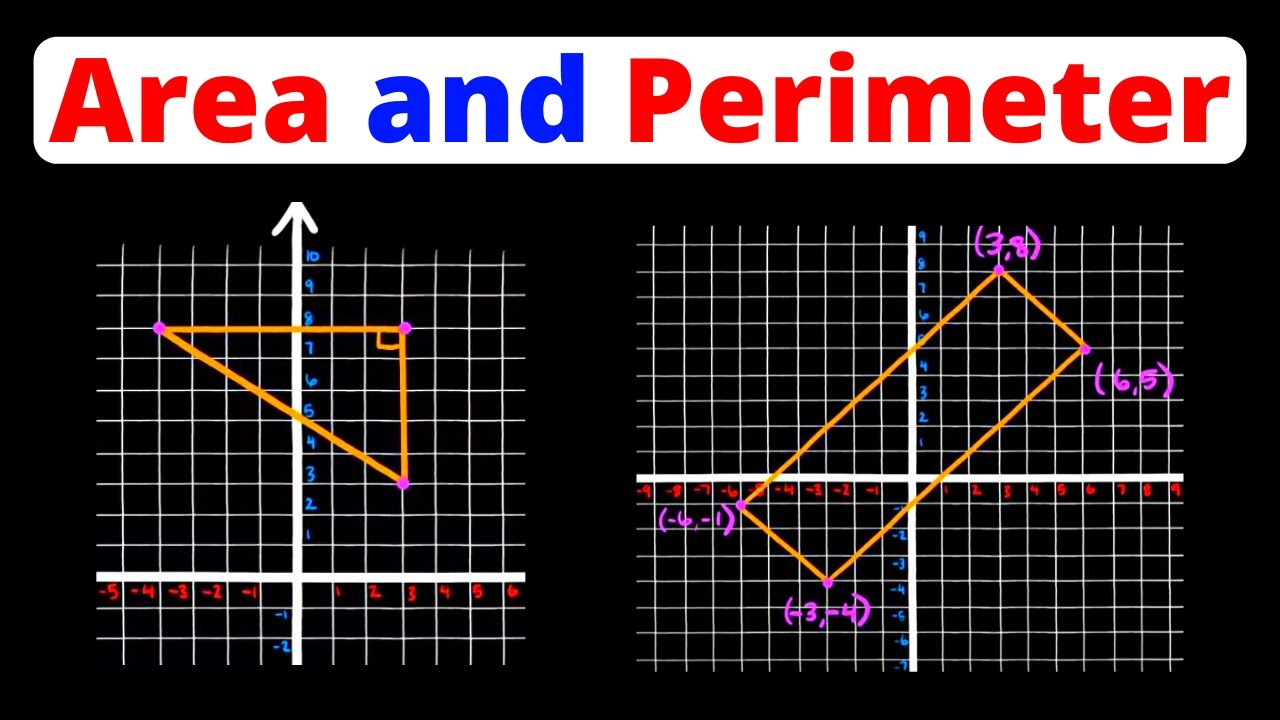
Area & Perimeter in the Coordinate Plane | Geometry | Eat Pi
5.0 / 5 (0 votes)
