calc 3 vector fields

Summary

This video explains key concepts in vector calculus, focusing on vector fields, gradient fields, and conservative fields. It covers how to interpret vector fields in both 2D and 3D, highlighting the relationship between a field and its components. The video explores how to determine if a vector field is conservative by checking the equality of mixed partials. Using practical examples, it demonstrates the process of verifying if a field has a potential function, illustrating the importance of equal mixed partials for conservativeness. The video concludes by emphasizing the significance of these concepts in understanding vector field behavior.

Takeaways

• 😀 Vector fields are functions that assign vectors to points in a domain, often in two or three dimensions (R² or R³).
• 😀 A vector field's output vectors can either be scalars or vectors, depending on the component functions.
• 😀 An example of a simple vector field is one where the x-component is 1 and the y-component is 0, resulting in a constant vector at each point.
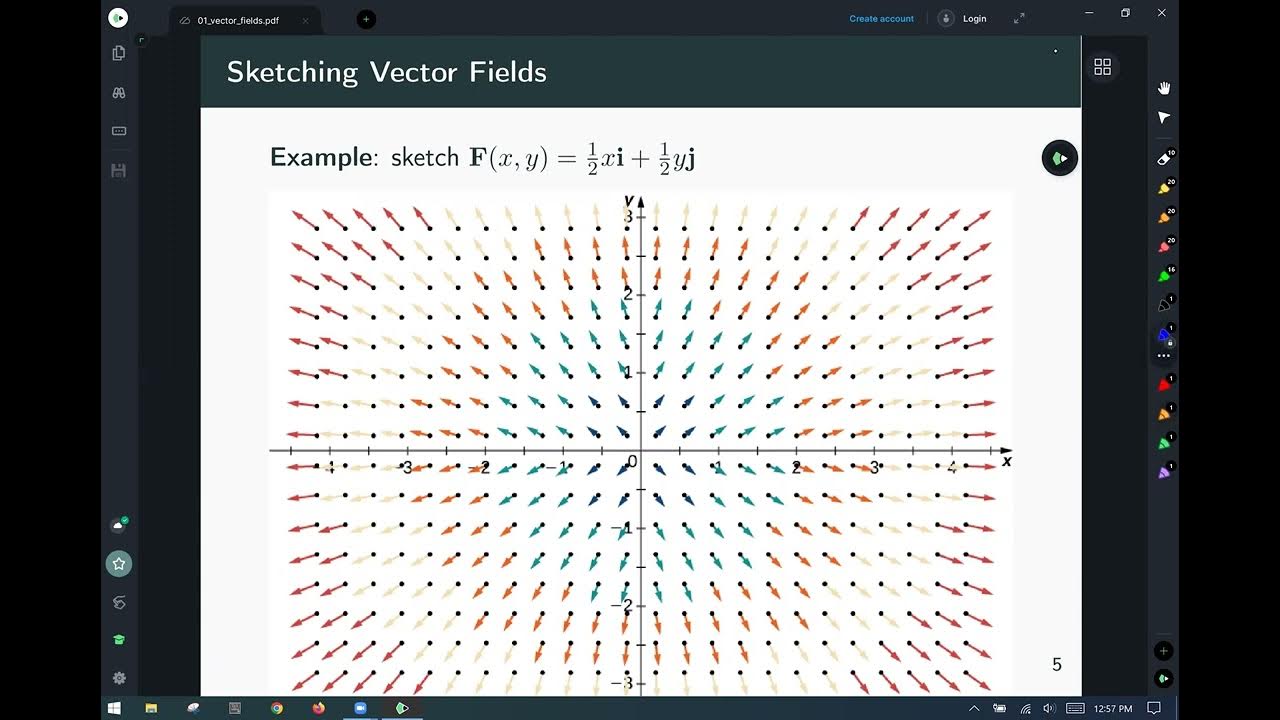
• 😀 For more complex vector fields, the vectors can depend on their location, like the field x*i + y*j, which outputs vectors proportional to the point's coordinates.
• 😀 Vector field visualization often involves scaling down the vectors to avoid clutter on the graph, especially for large or complex fields.
• 😀 The magnitude and direction of a vector field can change across the domain, such as in fields where vectors grow longer as they move away from the origin.
• 😀 A unit vector field has vectors with a constant magnitude of 1, achieved by normalizing the field (scaling each vector to unit length).
• 😀 A gradient field is a specific type of vector field that represents the gradient of a scalar function, indicating the direction of maximum increase of the function.
• 😀 Potential functions are scalar functions whose gradient produces the given vector field, and these fields are called conservative.
• 😀 To verify if a function is a potential function for a vector field, calculate the gradient of the scalar function and compare it to the vector field's components.
• 😀 A conservative vector field has equal mixed partials of its component functions. If the mixed partials are not equal, the field is not conservative.

Q & A

What is a vector field, and how is it represented in the plane?

A vector field is a function that takes an input point, typically an ordered pair (x, y) in the plane (R2), and assigns a vector to each point in its domain. The output vector can be represented by a pair of scalar functions for the x and y components.

How does the notation differ between scalar functions and vector functions in a vector field?

Scalar functions are represented by regular variables (e.g., p, q) without bold notation, while vector functions are denoted with bold or underlined symbols (e.g., F) to indicate that the output is a vector.

What is the significance of scaling vectors when plotting a vector field?

Scaling vectors helps to avoid cluttering the plot when the vectors have varying magnitudes. It allows for a clearer visualization of the direction and pattern of the vector field without the vectors becoming too long or overlapping.

How does the output of a vector field change when the components depend on the position?

When the components of a vector field depend on the position, the output vectors vary at different points in the domain. For example, a vector field like x i + y j would have vectors that change in magnitude and direction depending on the location in the plane.

What is a continuous vector field, and how does it extend to higher dimensions?

A vector field is continuous if its component functions are continuous. This concept extends to higher dimensions, where a vector field can take input triples (x, y, z) in three-dimensional space or higher, and output vectors accordingly.

How is a gradient field related to a scalar function?

A gradient field is the vector field obtained from the gradient of a scalar function. The gradient of a scalar function gives the direction of the greatest increase of the function, and its magnitude corresponds to the largest directional derivative at that point.

What is the process of finding a potential function for a vector field?

To find a potential function for a vector field, one must find a scalar function whose gradient gives the given vector field. If such a function exists, it is called a potential function, and the vector field is conservative.

What is a conservative vector field, and how can we verify its existence?

A conservative vector field is one that can be expressed as the gradient of a scalar potential function. To verify that a vector field is conservative, we check if its component functions satisfy the condition of equal mixed partials of second-order derivatives.

What does it mean for the mixed partials of a vector field to be equal?

For a vector field to be conservative, its mixed partials must be equal. This means that if the components of the vector field are differentiable, the mixed second-order partial derivatives of the components with respect to different variables should be identical.

How can you determine if a vector field is not conservative?

A vector field is not conservative if the mixed partials of its component functions are not equal. By calculating and comparing these partials, we can determine whether the field satisfies the conditions of a conservative vector field.