Übungsblatt6 – LGS mit 2 Unbekannten lösen und geometrische Interpretation Aufg.1c, e und f
Summary
TLDRThis video tutorial explains the process of solving systems of linear equations using different methods: substitution, elimination (addition), and graphical interpretation. It covers key concepts such as finding unique solutions where lines intersect, determining when systems have no solution due to parallel lines, and identifying infinite solutions when lines are identical. The tutorial also delves into vector analysis and linear combinations, helping viewers understand how to visualize and solve systems both algebraically and geometrically. Key takeaway: the solution can be interpreted through intersection points, parallelism, or identical lines, each representing different types of solutions.
Takeaways
- 😀 The video focuses on solving systems of linear equations using various methods such as substitution, elimination (addition), and graphical interpretation.
- 😀 A system with two equations and two unknowns (x1 and x2) is solved using substitution, where one variable is isolated and substituted into the other equation.
- 😀 The graphical interpretation of solutions involves plotting the corresponding linear equations, where the solution is the point of intersection of the two lines.
- 😀 When two linear equations have a single point of intersection, this point represents the unique solution to the system of equations.
- 😀 The concept of parallel lines is explained, where no solution exists when the lines are parallel, as they do not intersect.
- 😀 In the case of no solution, the two equations are inconsistent, and no common point exists between the two lines.
- 😀 The method of vector-based interpretation is introduced, where the solution can be understood as a linear combination of the corresponding vectors.
- 😀 The video explores the situation where a system of equations has infinitely many solutions, which occurs when the system is consistent and the two equations represent the same line.
- 😀 When there are infinitely many solutions, one variable is set as a free parameter, and the other variable is expressed in terms of this parameter.
- 😀 A variety of graphical techniques are shown, including how to plot lines and understand their behavior in the coordinate plane for different types of solutions (unique, no solution, infinite solutions).
Q & A
What is the main method used to solve the system of equations in this video?
-The main method used to solve the system of equations is substitution, elimination (addition), or the equalization method, where one equation is solved for one variable and substituted into the other equation.
What is the interpretation of the solution in terms of graphical representation?
-Graphically, the solution is interpreted as the intersection point of two lines, each representing a linear equation in the system. If the system has a unique solution, the lines intersect at one point.
How is the system of equations solved when there is no solution?
-When there is no solution, the system is shown graphically as two parallel lines, which do not intersect. This indicates that there are no common points or solutions to the system.
What does it mean when the system of equations has infinitely many solutions?
-Infinitely many solutions occur when the two equations are essentially the same line, leading to an infinite set of intersection points. In this case, the system is dependent, and the equations represent the same geometric line.
How is the parameterized solution presented when there are infinitely many solutions?
-In the case of infinitely many solutions, one variable is treated as a free parameter, and the other variable is expressed in terms of this parameter. For example, setting x2 as a parameter allows x1 to be expressed as a linear function of x2.
What happens in the case of a system with no solution when using the addition method?
-In the addition method, when no solution exists, adding the two equations results in a contradiction, such as 0 = 14, which implies that the system is inconsistent and does not have a solution.
What role do the slopes of the lines play in determining the solution?
-The slopes of the lines are crucial in determining the solution. If the slopes are different, the lines intersect at a single point, providing a unique solution. If the slopes are identical, the lines are parallel and do not intersect, meaning there is no solution.
Can the solution be visualized using vector notation in the column-wise method?
-Yes, the solution can be visualized using vectors in the column-wise method. The vectors corresponding to the equations are linearly combined to reach the solution vector. If the vectors are parallel, no combination will give the solution, indicating no solution.
What does it mean if two vectors are linearly dependent in this context?
-If two vectors are linearly dependent, it means that one vector can be expressed as a scalar multiple of the other, which implies the system has infinitely many solutions, as the equations represent the same line in the vector space.
How do you determine if two equations represent the same line geometrically?
-Two equations represent the same line geometrically if, after manipulation (such as solving for one variable), the equations are identical, meaning they have the same slope and y-intercept. This indicates infinitely many solutions, as the lines coincide.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Linear equations Gaussian Methods - English
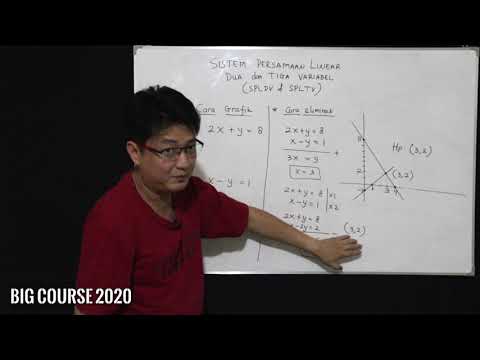
Matematika kelas X - Sistem Persamaan Linear part 1 - Sistem Persamaan Linear Dua Variabel (SPLDV)

Kurikulum Merdeka Matematika Kelas 9 Bab 1 Sistem Persamaan Linear Dua Variabel
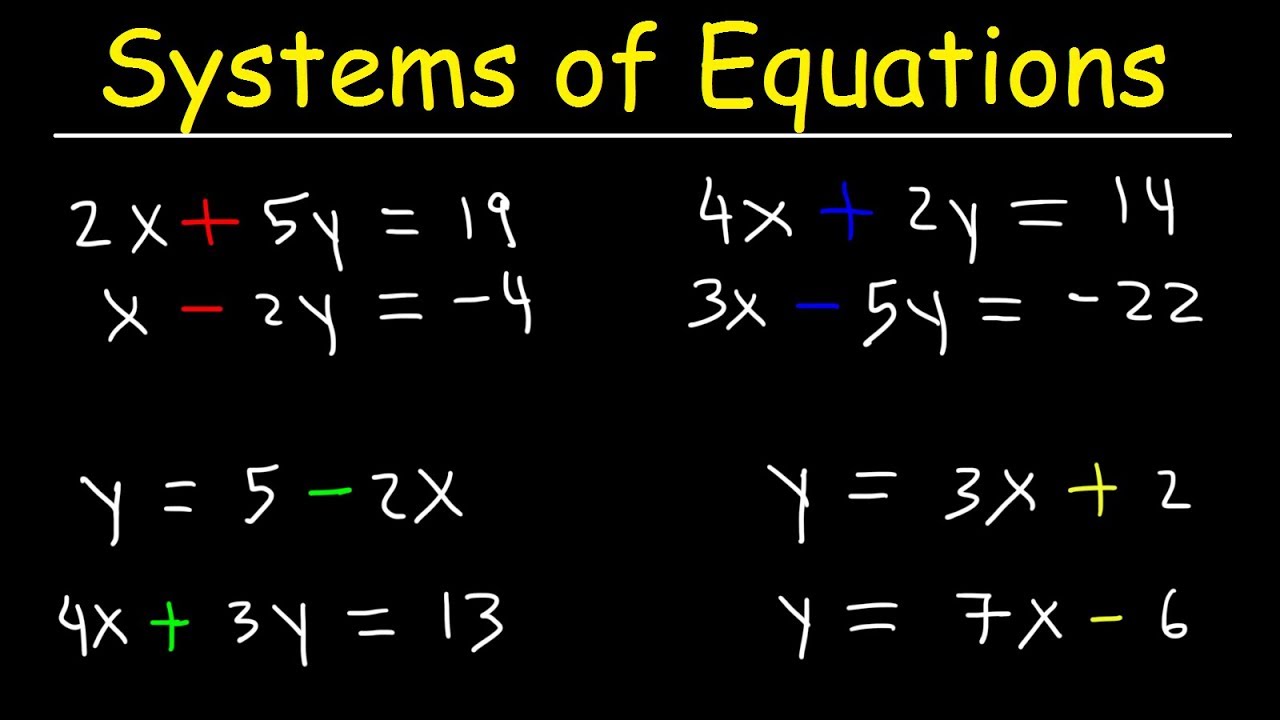
Solving Systems of Equations By Elimination & Substitution With 2 Variables
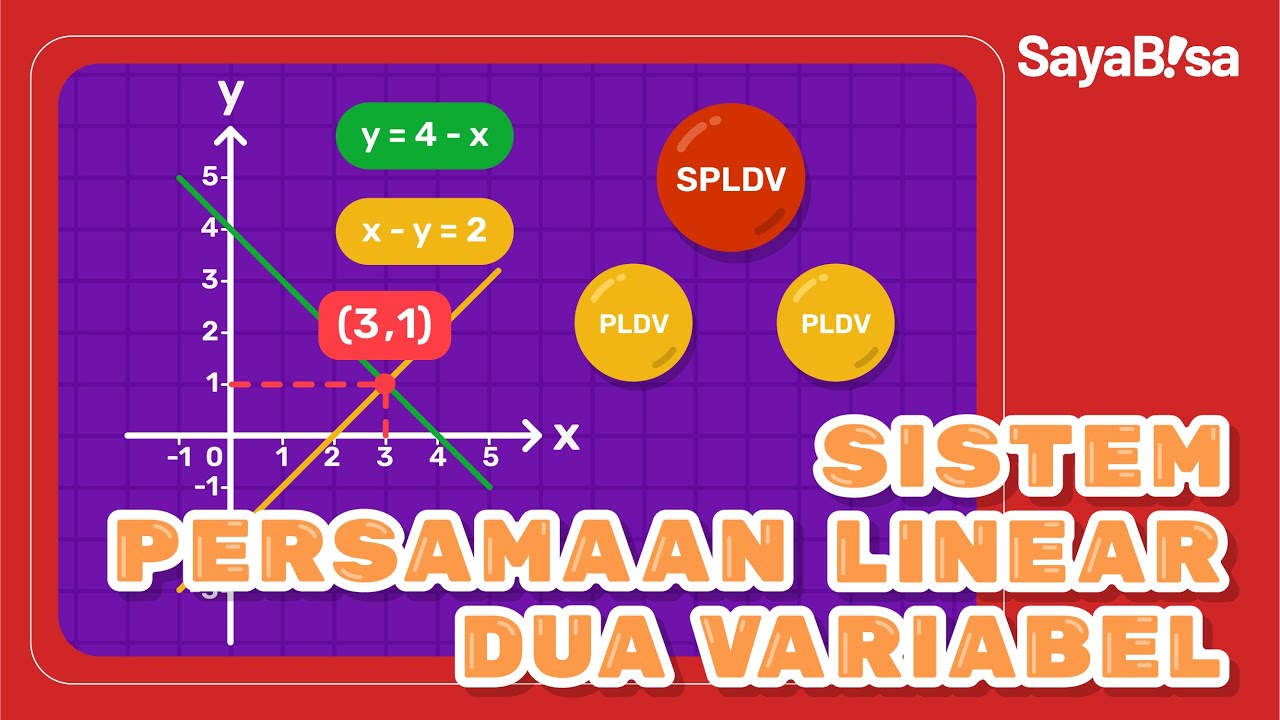
Sistem Persamaan Linear Dua Variabel | Matematika | SayaBisa

SPLDV - Metode Eliminasi
5.0 / 5 (0 votes)
