Spherical harmonics for Schrodinger equation
Summary
TLDRThis video explores spherical harmonics, vital mathematical functions in quantum mechanics, particularly in the context of the hydrogen atom. It details how these functions arise from the angular symmetry of the Schrödinger equation, emphasizing their separation into radial and angular components. The video explains the derivation of spherical harmonics through the associated Legendre functions and demonstrates their visualization in three dimensions. It highlights the significance of these functions, particularly in identifying the shapes of atomic orbitals such as S, P, and D orbitals, setting the stage for further discussions on the hydrogen atom.
Takeaways
- 😀 Spherical harmonics are mathematical functions defined on angular coordinates (phi and theta).
- 😀 They are important in quantum mechanics, particularly in the context of the electron's wave functions in hydrogen atoms.
- 😀 The spherical harmonics arise from the rotational symmetry of the hydrogen atom's Schrödinger equation.
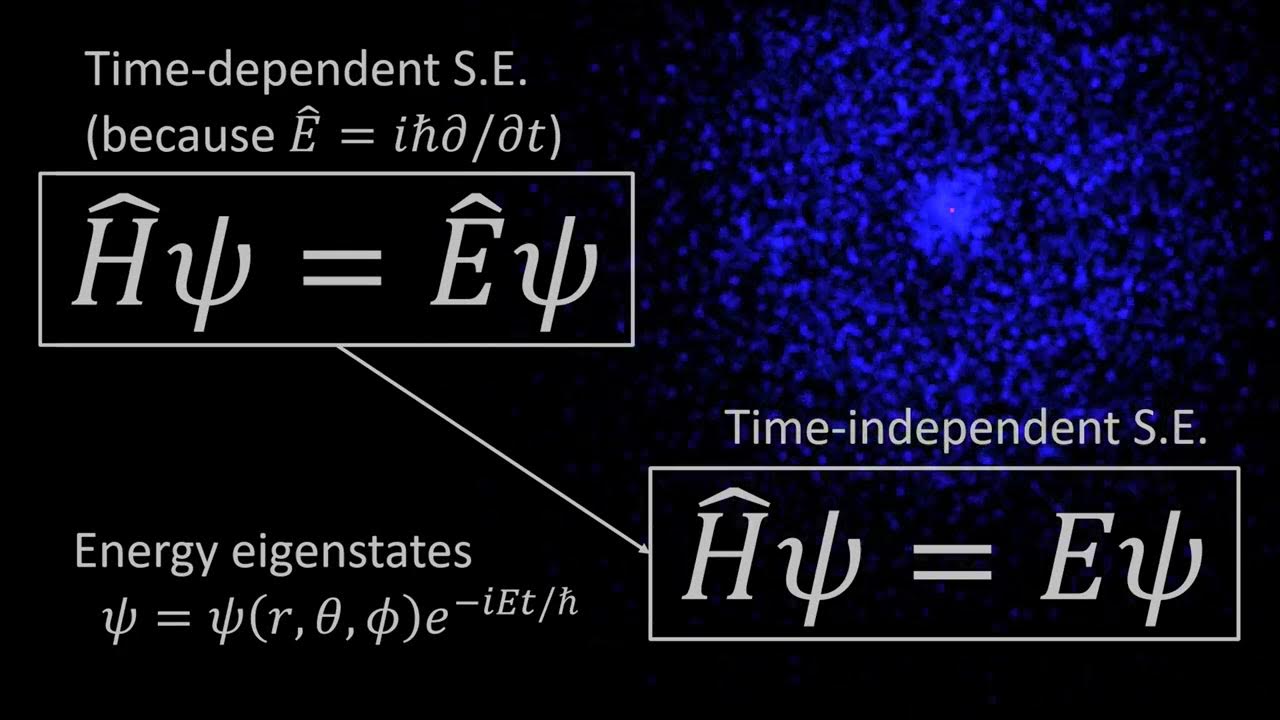
- 😀 The Schrödinger equation in three dimensions incorporates the Hamiltonian operator, including kinetic and potential energy terms.
- 😀 The problem of the central potential is best approached using spherical coordinates (r, theta, phi).
- 😀 The wave function can be expressed as a product of radial and angular functions, leading to the separation of variables.
- 😀 The angular equation, which yields spherical harmonics, was originally solved by Laplace in relation to gravitation.
- 😀 The azimuthal equation's solutions are complex exponentials, and M must be an integer for periodicity.
- 😀 The polar solutions are represented by associated Legendre functions, defined in terms of Legendre polynomials.
- 😀 The spherical harmonics are complex functions that can be transformed into real spherical harmonics using combinations of the complex forms.
Q & A
What are spherical harmonics?
-Spherical harmonics are special mathematical functions defined on angular coordinates, specifically the azimuthal and polar angles, Phi and Theta. They play a crucial role in quantum mechanics, particularly in describing the wave functions of electrons in a hydrogen atom.
Why are spherical harmonics important in quantum mechanics?
-Spherical harmonics are important because they emerge from the angular symmetry of problems like the hydrogen atom, contributing to the solutions of the Schrödinger equation and helping describe the spatial distribution of electrons.
How does the Schrödinger equation relate to spherical harmonics?
-The Schrödinger equation for a central potential can be separated into radial and angular components. The angular part leads to the angular equation, whose solutions are the spherical harmonics.
What is the role of the Hamiltonian operator in the Schrödinger equation?
-The Hamiltonian operator includes kinetic and potential energy terms, and it is used to derive the Schrödinger equation, which describes how the quantum state of a physical system changes over time.
What are the spherical coordinates used in this context?
-In spherical coordinates, a point in three-dimensional space is defined by three parameters: the radial distance (R), the polar angle (Theta), and the azimuthal angle (Phi).
What does the separation of variables method entail?
-The separation of variables method involves expressing the wave function as a product of functions, where one depends only on the radial coordinate and the other only on the angular coordinates, facilitating the solution of the equation.
What are associated Legendre functions?
-Associated Legendre functions are polynomial functions that arise in the solutions of the angular equation. They are defined in terms of Legendre polynomials and are crucial for determining the polar part of the spherical harmonics.
How are the spherical harmonics normalized?
-Spherical harmonics are normalized using a condition based on the volume element in spherical coordinates, ensuring that their total probability density integrates to one.
Can you describe the real spherical harmonics?
-Real spherical harmonics are obtained by combining complex spherical harmonics through linear combinations of their complex exponentials, typically using Euler's identity to express them in terms of sine and cosine functions.
What shapes do the spherical harmonics take for different values of l and m?
-For l=0, there is one spherical harmonic (a constant). For l=1, three shapes appear (p orbitals), and for l=2, five shapes arise (d orbitals), each with distinct lobular patterns corresponding to their m values.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード5.0 / 5 (0 votes)