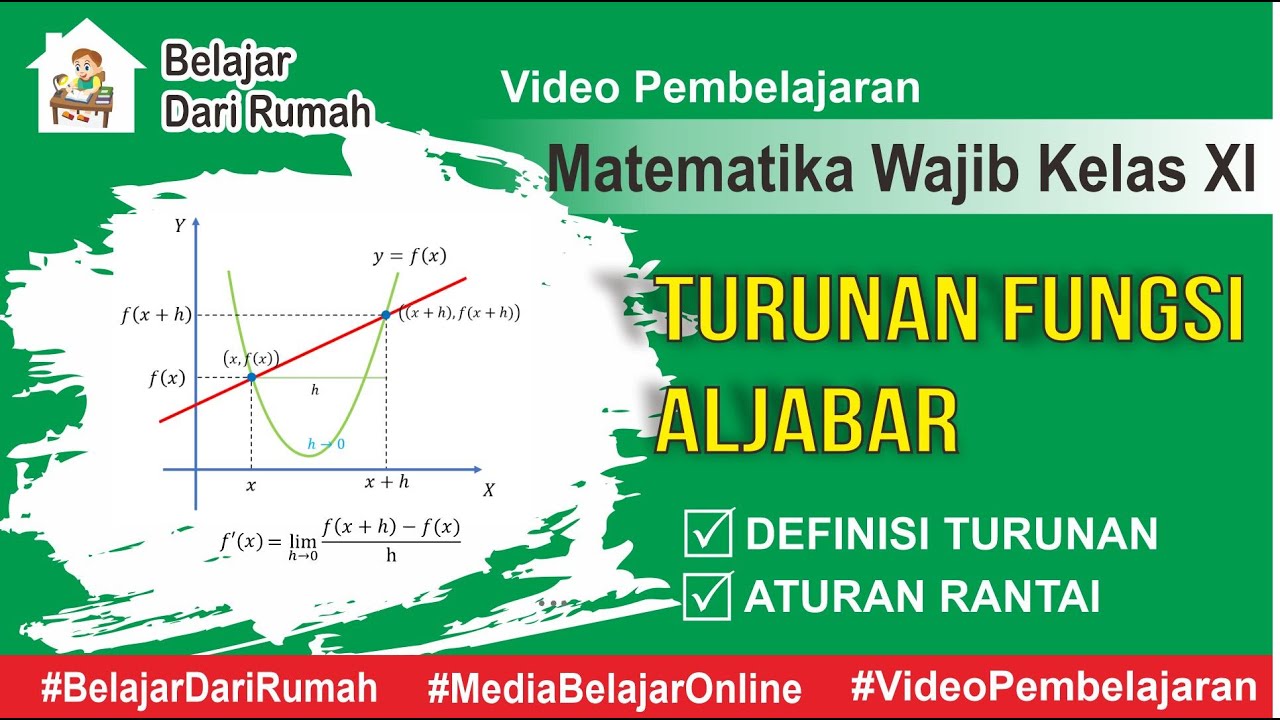
TURUNAN FUNGSI ALJABAR
Summary
TLDRIn this educational video, Iva introduces the fundamental concepts of algebraic derivatives, guiding students through the learning objectives of understanding derivatives, determining them for single functions, and applying differentiation rules. The lesson includes clear explanations of key rules such as the sum, product, and quotient rules, accompanied by practical examples. Students learn how to calculate derivatives for various functions, including constants and power functions, while also receiving quick differentiation techniques. The video concludes with motivational encouragement for students to trust in their abilities and apply what they've learned.
Takeaways
- 😀 The lesson focuses on understanding the concept of derivatives in algebraic functions.
- 📚 Students will learn to calculate the derivative of single-variable functions.
- 🔍 The derivative of a constant function, such as f(x) = a, is always 0.
- ✏️ For power functions, the derivative reduces the exponent by one and multiplies by the original exponent.
- 🔄 The power rule is exemplified with f(x) = 2x^3, where the derivative is 6x^2.
- 📈 When dealing with a function in the form of u^n, apply the chain rule to find its derivative.
- ➗ The script explains the rules for derivatives of addition and subtraction of functions.
- 🔢 The product rule for derivatives is outlined, indicating how to handle the multiplication of two functions.
- ⚖️ The quotient rule is introduced for finding the derivative of a division of two functions.
- 🌟 Quick derivative methods for common forms help simplify calculations efficiently.
Q & A
What is the main focus of the lesson presented in the transcript?
-The lesson focuses on understanding the concept of derivatives in algebraic functions, including how to determine and operate with derivatives.
What are the learning objectives outlined in the lesson?
-The learning objectives include understanding the concept of derivatives, determining derivatives of single functions, performing derivative operations, and solving related problems.
How is the derivative of a constant function defined?
-The derivative of a constant function, such as f(x) = a, is defined as zero, meaning f'(x) = 0.
What is the Power Rule for derivatives?
-The Power Rule states that if f(x) = x^n, then the derivative f'(x) = nx^{n-1}, where n is the exponent.
Can you explain the Chain Rule with an example?
-The Chain Rule is used for composite functions. For example, if f(x) = (2x + 5)^4, the derivative is found by applying the Power Rule to the outer function and multiplying by the derivative of the inner function: f'(x) = 4(2x + 5)^3 * 2.
What are the rules for performing operations on derivatives?
-The operations on derivatives include the sum/difference rule, product rule, and quotient rule, allowing for the combination and division of functions when finding their derivatives.
How do you apply the Product Rule in derivatives?
-The Product Rule states that if y = u * v, then the derivative y' = u'v + uv', where u and v are functions. You differentiate each function and apply the rule accordingly.
What is the Quotient Rule, and how is it applied?
-The Quotient Rule states that if y = u/v, then the derivative y' = (u'v - uv')/v^2. This involves differentiating the numerator and the denominator separately.
What method does the instructor suggest for quickly finding derivatives of quotients?
-The instructor suggests a quick method for derivatives of quotients using a formula that involves the products of the numerator and denominator derivatives, aiming to simplify calculations.
What motivational message does the instructor conclude the lesson with?
-The instructor concludes with a motivational message emphasizing the importance of believing in oneself when facing challenges.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード5.0 / 5 (0 votes)