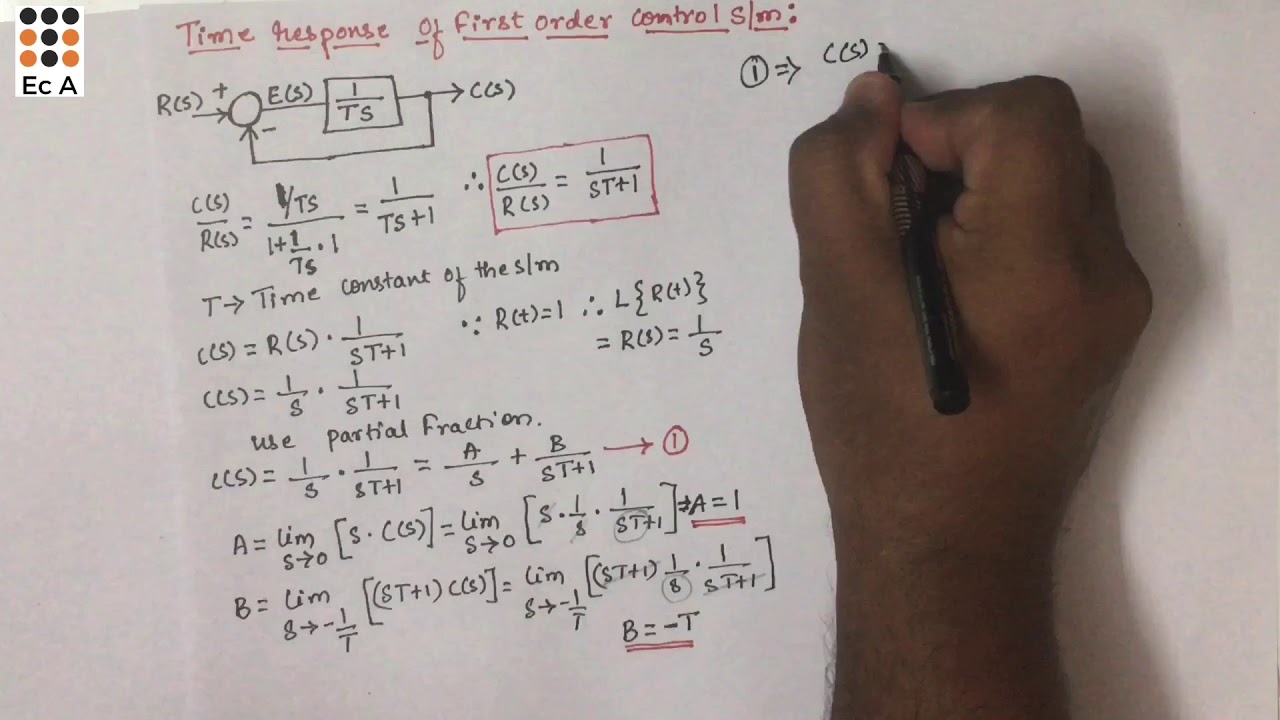
#174 Time response of second order control systems || EC Academy
Summary
TLDRIn this lecture, Ajay introduces the latest Indian Standard Time response of second-order control systems. The session explores key concepts, including transfer functions, natural frequency, and damping ratio, while addressing questions about system responses and characteristics. It emphasizes the importance of understanding system behavior under various conditions, with practical examples and calculations. Ajay also highlights the implications of complex roots and the conditions leading to negative responses. This engaging overview is designed to enhance comprehension of control systems and their applications.
Takeaways
- 😀 The lecture focuses on the latest Indian Standard Time response of second-order control systems.
- 😀 The system can be divided into a simple transfer function, facilitating easier analysis.
- 😀 Natural frequency and damping ratio are important parameters for understanding system responses.
- 😀 The behavior of the system is characterized by its response to disturbances and input signals.
- 😀 It is crucial to understand the characteristics of poles and zeros in the system's transfer function.
- 😀 Complex poles indicate potential oscillatory behavior, which can affect stability.
- 😀 Various conditions are examined to determine the system's response and performance.
- 😀 The importance of calculating the distance from roots to denominators is emphasized for system stability.
- 😀 The lecture also covers how to handle equations involving imaginary roots, relevant for system analysis.
- 😀 Understanding system responses is essential for designing effective control systems.
Q & A
What is the main topic of the lecture?
-The lecture discusses the latest Indian standard time response of second-order control systems.
Who conducted the survey mentioned in the lecture?
-The survey was conducted by the Leaf GIA officer.
How can the transfer function of a system be divided?
-The transfer function can be divided into a square plus two transfer functions.
What is the significance of natural frequency in control systems?
-Natural frequency is crucial for understanding the dynamic behavior of the system, including its response to disturbances.
What happens when the system reaches a positive traffic jam condition?
-In a positive traffic jam condition, the system gradually increases its power, impacting the overall system response.
What are poles and zeros in the context of control systems?
-Poles are the values that cause the system's transfer function to go to infinity, while zeros are the values that cause it to become zero.
What is the damping ratio mentioned in the lecture?
-The damping ratio is a measure of how oscillations in a system decay after a disturbance, affecting system stability and response.
What is the importance of calculating the distance from the system roots?
-Calculating the distance from system roots helps determine system stability and performance characteristics.
How does the system's response change with variations in input?
-The system's response will vary based on input conditions, such as changes in gain or frequency, impacting stability and performance.
What does the term 'imaginary roots' refer to in the context of this lecture?
-Imaginary roots refer to system characteristics where the response does not return to equilibrium, indicating potential instability.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード5.0 / 5 (0 votes)