Lingkaran dan Busur Lingkaran | #PekanBuktiKarya
Summary
TLDRIn this educational video for 11th-grade students, the topic of circles and circular arcs is explored. The lesson aims to help students apply theorems related to circles and calculate arc lengths and areas of sectors. The presenter engages viewers by discussing the significance of circular shapes in daily life, such as in bicycle wheels and manhole covers. Key concepts include the relationship between central angles and inscribed angles, along with practical examples and exercises to reinforce learning. Students will gain a solid understanding of circular geometry, equipping them for real-world problem-solving.
Takeaways
- 😀 The lesson focuses on circles and arcs, specifically for 11th-grade students in phase F.
- 📏 Students will learn to apply theorems related to circles to determine arc lengths and the area of sectors.
- 🌍 The concepts will help solve real-world problems, including positioning on Earth and measuring distances between locations.
- 🔄 Understanding the relationship between central angles and inscribed angles facing the same arc is a key learning objective.
- 🚴 The circular shape of bicycle wheels is highlighted as essential for smooth riding on flat surfaces.
- 🕳️ The importance of circular manhole covers is discussed for safety, compared to square or rectangular shapes that can pose risks.
- 🔵 The transcript explains that a circular region can be formed by connecting points that are equidistant from a center point.
- 📐 The definition of an arc is provided, detailing its formation from the intersection of two radii of a circle.
- 🔍 Key terms such as 'minor arc' and 'major arc' are introduced to describe parts of the circle's circumference.
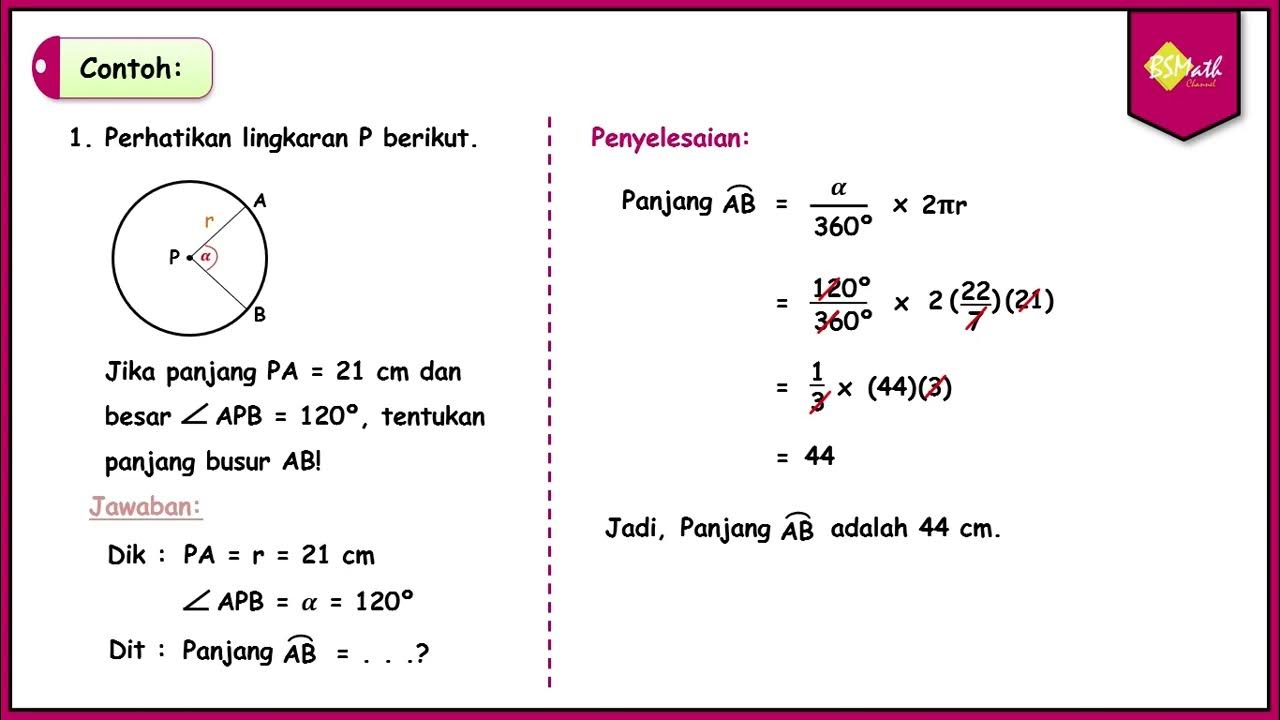
- 📊 Various example problems are solved, illustrating how to calculate angles and lengths related to circles using theorems.
Q & A
What is the primary focus of the lesson on circles and arcs?
-The primary focus is to understand theorems related to circles, calculate the lengths of arcs, and determine the areas of circular sectors.
How does the lesson plan to relate circular shapes to real-life applications?
-The lesson discusses practical implications of circular shapes, such as their use in bicycle wheels and manhole covers, emphasizing safety and functionality.
What is a central angle, and how is it defined?
-A central angle is defined as an angle whose vertex is located at the center of the circle, with its sides extending to the circumference.
What is the relationship between inscribed angles and central angles in a circle?
-The measure of an inscribed angle is always half that of the central angle that subtends the same arc.
What are the two types of arcs mentioned in the lesson?
-The two types of arcs mentioned are the minor arc, which is the shorter arc connecting two points, and the major arc, which is the longer arc between the same two points.
What is the significance of Thales' theorem in relation to circles?
-Thales' theorem states that an angle inscribed in a semicircle is a right angle (90°), establishing a crucial relationship between diameters and inscribed angles.
If angle POQ is 90°, what is the measure of angle BDC?
-If angle POQ is 90°, then angle BDC is calculated as half of that, making it 45°.
In a circle with a radius of 2 units, if BC = 2, what is the measure of angle BDC?
-Given that triangle ABC is equilateral, angle BAC is 60°, thus angle BDC is half of that, which is 30°.
How can you find the length of a diameter if the radius is 8.5 cm?
-The diameter can be found by doubling the radius: diameter AB = 2 * 8.5 cm = 17 cm.
What mathematical theorem can be used to find the length of BC in the triangle mentioned?
-The Pythagorean theorem can be used to find the length of BC in the triangle formed, resulting in BC being 15 cm.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示
Lingkaran dan Busur Lingkaran - Matematika SMA Kelas XI Kurikulum Merdeka

Materi Lingkaran, Unsur Lingkaran dan Hubungan Sudut Pusat Sudut Keliling Kelas XI Kur Merdeka

MATERI PAI KELAS XI | BAB 6 | TOLERANSI DAN MEMELIHARA KEHIDUPAN MANUSIA

Bahasa Arab ISTIMA' kelas XI MA Bab 5

CHORDS, ARCS AND ANGLES || GRADE 10 MATHEMATICS Q2

Materi Cerpen kelas 11 SMA/SMK
5.0 / 5 (0 votes)
