How To Factor Polynomials The Easy Way!
Summary
TLDRThis video tutorial offers a comprehensive guide on factoring polynomials, starting with basic examples like extracting the greatest common factor (GCF) from expressions like 6x - 12. It then advances to factoring trinomials with a leading coefficient of one and proceeds to more complex scenarios, including factoring by grouping and handling expressions with no initial GCF. The video also covers factoring perfect square differences and concludes with a method for factoring polynomials with four terms by recognizing patterns in coefficients, providing a step-by-step approach to mastering polynomial factoring.
Takeaways
- 📚 Start with simple polynomial factoring by identifying and extracting the greatest common factor (GCF).
- 🔍 For expressions like 3x³ - 9x² and 4x² - 12x, factor out the GCF to simplify the expression to x(x² - 3x) and x(4x - 12) respectively.
- 📉 Factoring trinomials involves finding two numbers that multiply to the product of the squared term and add up to the linear coefficient.
- 🎯 Example: x² + 2x - 15 factors to (x + 5)(x - 3) by using the numbers 5 and -3 that meet the above criteria.
- 📝 When the leading coefficient is not one, factor out the GCF first, then proceed to factor the resulting trinomial.
- ⚖️ For a trinomial like 2x² - 6x - 56, factor out the GCF of 2, resulting in x² - 3x - 28, which then factors further to (2)(x - 7)(x + 4).
- 🛑 Difference of perfect squares can be factored by taking the square root of each term and using the identity (a² - b²) = (a + b)(a - b).
- 📐 Examples of perfect square differences include x² - 16, x² - 64, 4x² - 25, and 9x² - 49, which factor to (x + 4)(x - 4), (x + 8)(x - 8), (2x + 5)(2x - 5), and (3x + 7)(3x - 7) respectively.
- 🔑 Factoring by grouping is used when no GCF is present and involves multiplying the leading coefficient by the constant term to find two numbers that meet specific criteria.
- 🔄 For expressions like 2x² - 5x - 3, replace the middle term with two terms that add up to the original middle term and have a product equal to the product of the leading and constant terms, then factor by grouping.
- 📈 Practice is key to mastering polynomial factoring, as demonstrated by the various examples provided in the script.
Q & A
What is the first step in factoring the polynomial 6x - 12?
-The first step is to take out the greatest common factor (GCF), which in this case is 6.
How is the expression 3x^3 - 9x^2 factored?
-The GCF, which is 3x^2, is factored out, leaving x - 3 as the remaining terms.
What is the GCF of the polynomial 4x^2 - 12x?
-The GCF of this polynomial is 4x, which can be factored out to leave x - 3 as the remaining terms.
How do you factor a trinomial when the leading coefficient is one?
-You find two numbers that multiply to the constant term and add up to the coefficient of the linear term.
What two numbers multiply to 12 and add to 7 for the trinomial x^2 + 7x + 12?
-The two numbers are 3 and 4, as 3 * 4 = 12 and 3 + 4 = 7.
How do you factor the trinomial x^2 + 2x - 15?
-You find two numbers that multiply to -15 and add to 2, which are 5 and -3, resulting in (x + 5)(x - 3).
What is the first step in factoring the polynomial 2x^2 - 6x - 56?
-The first step is to factor out the GCF, which is 2, from all terms of the polynomial.
What is the GCF of the polynomial 3x^2 - 18x + 24?
-The GCF of this polynomial is 3, which can be factored out to leave x^2 - 6x + 8.
How do you factor a difference of perfect squares like x^2 - 16?
-You take the square root of each term, resulting in (x - 4)(x + 4) since √x^2 is x and √16 is 4.
What is the process of factoring by grouping used for?
-Factoring by grouping is used when there is no common factor to extract and the polynomial has more than two terms, allowing you to group terms and factor out common binomials.
How do you factor the polynomial 2x^2 - 5x - 3 when there is no GCF?
-You multiply the leading coefficient by the constant term to find a pair of numbers that multiply to -6 (the result of 2 * -3) and add to the middle coefficient, -5. Then you replace the middle term with the sum of these two numbers and factor by grouping.
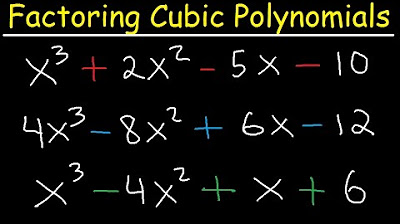
What is the final factored form of 3x^3 - 2x^2 - 12x + 8?
-After factoring out the GCFs and using factoring by grouping, the final factored form is (3x + 2)(x - 4)(x + 1).
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
5.0 / 5 (0 votes)