Factor Polynomials with GCMF Video
Summary
TLDRThis video script offers a comprehensive guide to factoring polynomials by identifying the greatest common monomial factor (GCMF). It explains the process of finding the GCMF by examining both numerical coefficients and variable exponents, using examples like 6x + 4x^2 and 9x^3y^7 - 18xy^2 - 36y. The script demonstrates how to simplify polynomials by dividing each term by the GCMF and combining the results, resulting in factored forms that are easier to understand and solve.
Takeaways
- 🔍 The target of the script is to teach how to factor polynomials with the greatest common monomial factor (GCM).
- 📚 The GCM is defined as the highest possible value of a number or a combination of numbers and variables that is common in each term of a polynomial.
- 📝 The process of factoring polynomials involves three main steps: identifying the GCM, dividing each term by the GCM, and combining the results.
- 📉 For the polynomial 6x + 4x^2, the GCM of the numerical coefficients (6 and 4) is 2, and the GCM of the variables (x and x^2) is x to the least exponent, which is x.
- 📈 After finding the GCM, the script demonstrates dividing each term of the polynomial by the GCM, resulting in 3 and 2x, respectively.
- 📚 The script then combines the results to show the factored form of the polynomial as 2x(3 + 2x).
- 🔢 In the second example, the polynomial 9x^3y^7 - 18xy^2 - 36y, the GCM of the numerical parts (9, -18, -36) is 9, and the GCM of the variables is y squared.
- 📉 The script illustrates dividing each term of the polynomial by the GCM, 9y^2, to simplify it to x^3y^8 - 2x - 45.
- 📝 The final factored form of the second polynomial is 9y^2(x^3y^5 - 2x - 45).
- 👉 The script emphasizes the importance of finding the least exponent for the variable part of the GCM when variables have different powers.
- 📌 The examples provided in the script serve to illustrate the step-by-step process of factoring polynomials using the GCM method.
Q & A
What is the greatest common monomial factor (GCMF)?
-The GCMF is the highest possible value of a number or a combination of numbers and variables which is common in each term of a polynomial.
What are the steps involved in factoring polynomials using the greatest common factor?
-The steps are: 1) Find the greatest common monomial factor, 2) Divide each term in the polynomial by the GCMF, and 3) Combine the answers from steps one and two.
How do you determine the greatest common factor (GCF) of numerical coefficients in a polynomial?
-You determine the GCF of the numerical coefficients by finding the largest number that divides evenly into all the coefficients of the terms in the polynomial.
What is the GCF of the variables in the polynomial 6x + 4x^2?
-The GCF of the variables in 6x + 4x^2 is x, since x is the common variable with the least exponent.
What is the greatest common monomial factor for the polynomial 6x + 4x^2?
-The GCMF for the polynomial 6x + 4x^2 is 2x, which is the product of the GCF of the numerical coefficients (2) and the GCF of the variables (x).
How do you simplify the polynomial 6x + 4x^2 after finding the GCMF?
-After finding the GCMF of 2x, you divide each term by 2x, resulting in 3 and 2x, respectively, and then write the simplified polynomial as 2x(3 + 2x).
What is the GCF of the numerical parts of the polynomial 9x^3y^7 - 18xy^2 - 36y?
-The GCF of the numerical parts (9, -18, -36) is 9.
How do you determine the GCF of the variables in the polynomial 9x^3y^7 - 18xy^2 - 36y?
-The GCF of the variables is determined by the least exponent of the common variable present in all terms, which in this case is y squared (y^2).
What is the greatest common monomial factor for the polynomial 9x^3y^7 - 18xy^2 - 36y?
-The GCMF for the polynomial 9x^3y^7 - 18xy^2 - 36y is 9y^2, considering both the GCF of the numerical parts and the GCF of the variables.
How do you simplify the polynomial 9x^3y^7 - 18xy^2 - 36y after finding the GCMF?
-After finding the GCMF of 9y^2, you divide each term by 9y^2, resulting in x^3y^5, -2x, and -4, and then write the simplified polynomial as 9y^2(x^3y^5 - 2x - 4).
Why is it important to factor polynomials?
-Factoring polynomials is important as it simplifies the expression, making it easier to solve equations, understand the roots, and perform various algebraic manipulations.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

Factoring Polynomials using Greatest Common Monomial Factor

Factoring Greatest Common Monomial Factor

Grade 8 Math Q1 Ep1: Factoring Polynomials
How To Factor Polynomials The Easy Way!

Common Monomial Factoring - Polynomial Factoring - Grade 8 Math
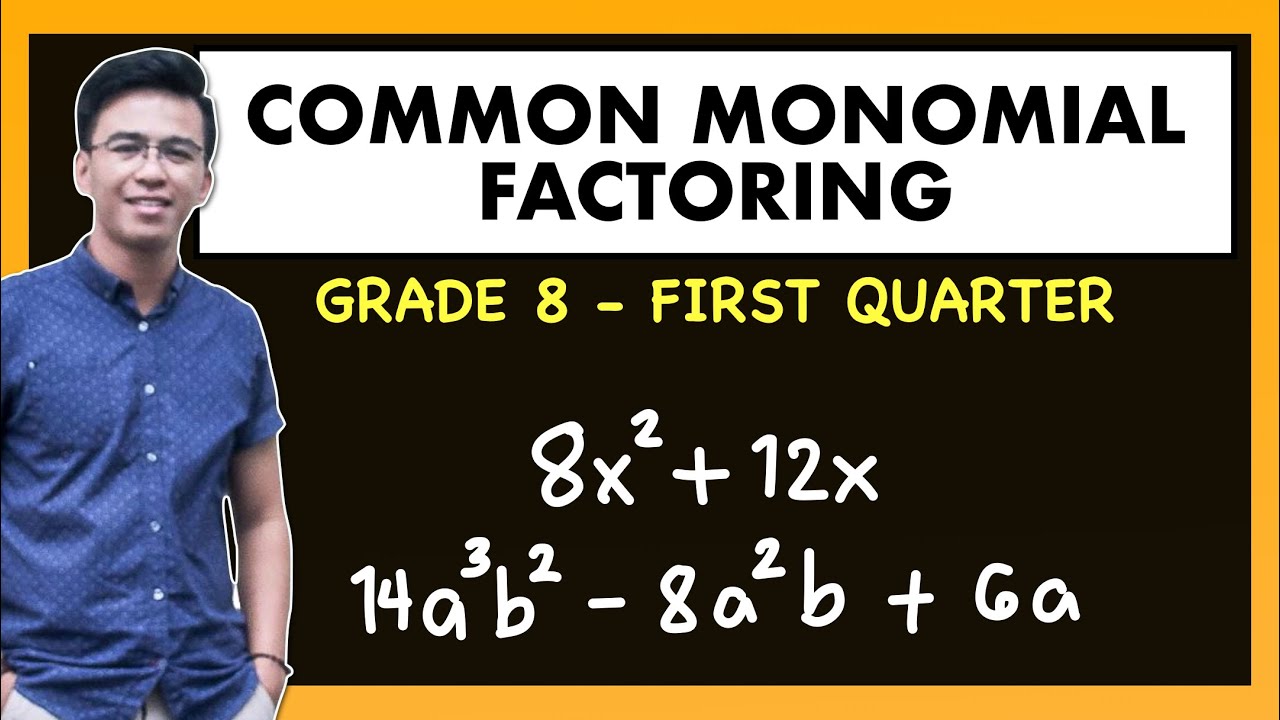
Factoring Part 1 - Common Monomial Factoring | Grade 8 Q1 @MathTeacherGon
5.0 / 5 (0 votes)
