KONSEP, SOAL, DAN PEMBAHASAN MATERI DISTRIBUSI BINOMIAL![MATEMATIKA PEMINATAN KELAS 12]
Summary
TLDRThis video explains the concept of Bernoulli trials and the binomial distribution, with a focus on the calculation of probabilities in different scenarios. It covers how to determine the probability of success and failure in repeated trials, using formulas such as the binomial distribution formula. Examples are provided, including coin tosses, egg selection, and penalty kick scenarios, with step-by-step demonstrations of how to apply the binomial distribution to solve for the probability of specific outcomes. The video emphasizes the importance of understanding combination formulas and applying them correctly in various problems.
Takeaways
- 😀 A Bernoulli trial (binomial experiment) involves repeating an experiment with two possible outcomes: success or failure.
- 😀 In a binomial experiment, the probability of success (P) and failure (Q) must remain constant throughout all trials, with P + Q = 1.
- 😀 The binomial distribution formula is P(X = x) = C(n, x) * p^x * (1-p)^(n-x), where C(n, x) is the binomial coefficient.
- 😀 The binomial coefficient, C(n, x), represents the number of ways to choose 'x' successes from 'n' trials, calculated as n! / (x!(n-x)!).
- 😀 The probability of success (P) and failure (Q) depend on the specific problem, like getting heads or tails in a coin toss.
- 😀 The formula for binomial probability requires knowing the number of trials (n), the number of successes (x), and the probabilities (p and q).
- 😀 A successful binomial trial must only have two outcomes: success or failure. For example, in a coin toss, heads is success, and tails is failure.
- 😀 In binomial distribution, each trial is independent, meaning the outcome of one trial doesn't affect the others.
- 😀 The binomial distribution is widely used in scenarios like coin tossing, quality control (defective products), and sports performance (e.g., goals scored).
- 😀 The solution for binomial problems often involves calculating probabilities for specific numbers of successes and summing them when necessary (e.g., P(X > 2) in multiple trials).
Q & A
What is a Bernoulli experiment?
-A Bernoulli experiment is a trial or experiment that can have only two possible outcomes: success or failure. The probability of success remains constant across repeated trials.
What is the relationship between P and Q in a Bernoulli experiment?
-In a Bernoulli experiment, P is the probability of success, and Q is the probability of failure. They are related by the equation P + Q = 1, meaning that the sum of the probabilities of success and failure must equal 1.
How is the binomial distribution formula derived?
-The binomial distribution formula is given by: CX from n * P^x * Q^(n-x), where C is the combination formula, n is the number of trials, P is the probability of success, Q is the probability of failure, and x is the number of successes.
What role does the combination formula play in binomial distribution?
-The combination formula calculates the number of ways a specific number of successes (x) can occur in a set of trials (n). This is essential in determining the likelihood of various outcomes in a binomial distribution.
What are the key properties of a binomial distribution?
-The key properties of a binomial distribution are: (1) The experiment is repeated multiple times, (2) There are two possible outcomes for each trial (success or failure), (3) The probability of success is constant across trials, and (4) The trials are independent of each other.
How would you calculate the probability of getting 3 heads when tossing a coin 8 times?
-To calculate this, you would use the binomial distribution formula with n = 8, x = 3, P = 0.5 (probability of heads), and Q = 0.5 (probability of tails). The result is the combination of 3 successes from 8 trials, multiplied by the probabilities raised to the appropriate powers.
In the example of 25% of eggs being bad, how would you calculate the probability of picking 3 bad eggs from 4?
-In this case, P (probability of a bad egg) is 0.25, Q (probability of a good egg) is 0.75, n is 4 (total eggs), and x is 3 (bad eggs). Using the binomial distribution formula, you would calculate the combination of 3 bad eggs from 4, multiplied by the probabilities for each outcome.
What does the term 'combination' refer to in binomial distribution?
-In binomial distribution, the 'combination' refers to the number of different ways you can select x successes from n trials. It is calculated using the combination formula: C(n, x) = n! / (x!(n-x)!)
What does the notation P^x and Q^(n-x) represent in the binomial distribution formula?
-In the binomial distribution formula, P^x represents the probability of success raised to the power of the number of successes (x), and Q^(n-x) represents the probability of failure raised to the power of the number of failures (n - x).
How do you calculate the probability of a player scoring more than 2 goals out of 4 penalty kicks, with a success rate of 0.8 per kick?
-To calculate this, you would find the probabilities of the player scoring 3 and 4 goals (using the binomial distribution formula for x = 3 and x = 4), and then sum these probabilities to get the total probability of scoring more than 2 goals.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

Binomial distribution # Explanation with notes# Properties of binomial distribution.

Bien rédiger un exercice contenant la loi binomiale. Bernoulli. Probabilité.Terminale.

Metode Statistika | Sebaran Peluang Diskrit | Bernoulli | Binomial | Poisson
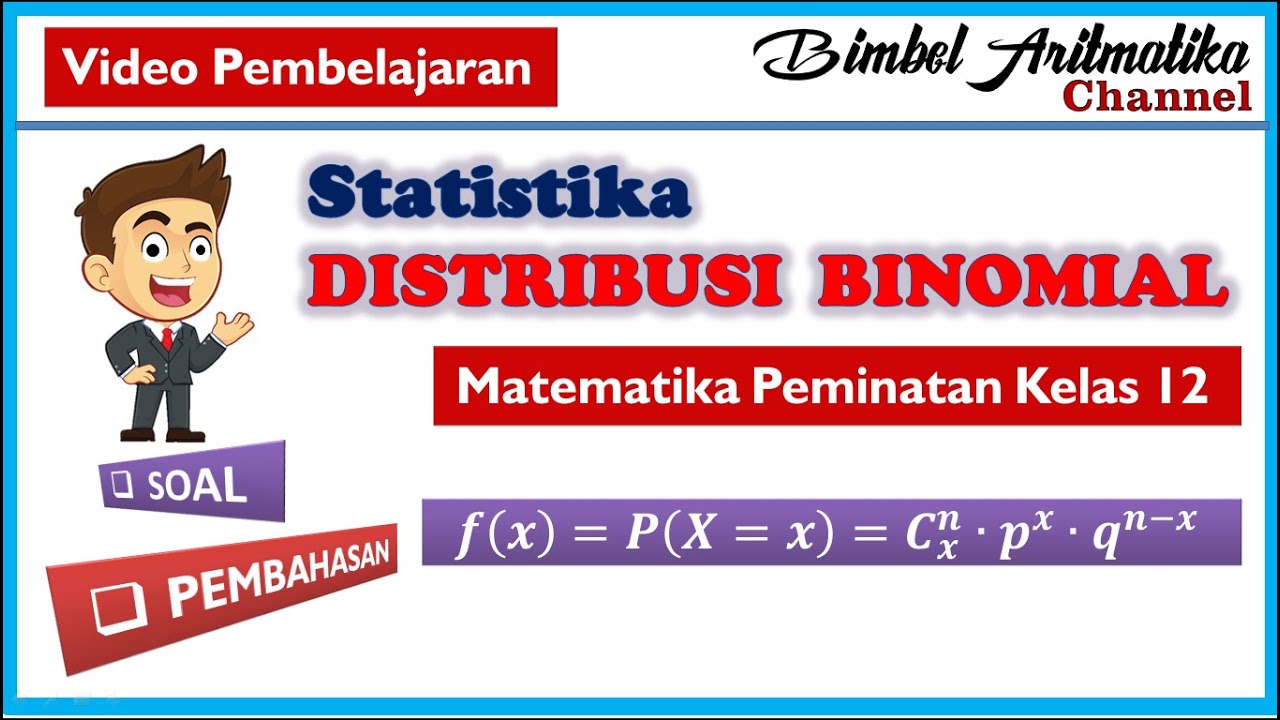
Distribusi Probabilitas Binomial | Matematika Peminatan Kelas 12

Distribusi Binomial • Part 12: Contoh Soal Distribusi Peluang Binomial (3)

Sesi 5 3 Distribusi Probabilitas Bagian 3
5.0 / 5 (0 votes)
