TRIGONOMETRI - Rumus Penjumlahan ke Perkalian Sin dan Cos
Summary
TLDRIn this tutorial, the speaker explains the transformation of trigonometric expressions using sum-to-product formulas for sine and cosine. The video covers the key formulas that simplify sums and differences of trigonometric functions into products, providing clear step-by-step examples. The speaker guides viewers through solving practical exercises, ensuring a deep understanding of how to apply these identities. The lesson emphasizes memorization and practice of these formulas, with additional exercises for reinforcement. Overall, this tutorial is designed to help students master trigonometric simplifications efficiently.
Takeaways
- 😀 The video discusses the conversion of trigonometric formulas from product-to-sum and sum-to-product identities for sine and cosine.
- 😀 It emphasizes the importance of understanding product-to-sum identities before moving on to sum-to-product identities.
- 😀 The formulas for sine and cosine are presented as: 2 * sin(α) * cos(β), 2 * cos(α) * cos(β), and others.
- 😀 The video explains how to manipulate angles to match sum-to-product identities, such as the transformations of α + β and α - β.
- 😀 Key formulas include: sin(α) + sin(β) = 2 * sin((α + β) / 2) * cos((α - β) / 2), and similar ones for other combinations of sine and cosine.
- 😀 The tutorial involves solving specific examples where sum-to-product identities are applied to trigonometric expressions like sin(75°) + sin(15°) and cos(165°) - cos(105°).
- 😀 The lesson also addresses complex cases, such as combining sin and cos in the same equation and transforming them into similar forms.
- 😀 The importance of memorizing the core formulas and knowing when to apply them is stressed throughout the video.
- 😀 The video shows how simplifying expressions and using standard values for sine and cosine (like sin(45°) or cos(30°)) makes solving easier.
- 😀 The video concludes by offering practice problems to reinforce understanding and ensure viewers can apply the discussed formulas in various scenarios.
Q & A
What is the main topic of the video?
-The main topic of the video is the mathematical concept of converting trigonometric identities from product to sum and vice versa, specifically focusing on sine and cosine functions.
What is the first identity discussed in the video?
-The first identity discussed is the product-to-sum identity for sine and cosine, where the product of sin(α)cos(β) is expressed as a sum involving trigonometric functions.
How does the product-to-sum identity for sin(α)cos(β) look like?
-The identity for sin(α)cos(β) is expressed as: sin(α)cos(β) = 1/2 [sin(α + β) + sin(α - β)].
What happens when both angles in the identity are the same?
-When both angles are the same, such as in sin(α)cos(α), the resulting expression simplifies to 1/2[sin(2α)].
What formula is used to convert a sum of sines into a product?
-To convert a sum of sines (sin(α) + sin(β)) into a product, the formula used is: sin(α) + sin(β) = 2 sin[(α + β)/2] cos[(α - β)/2].
Can you explain the formula for converting a sum of cosines into a product?
-The formula for converting a sum of cosines (cos(α) + cos(β)) into a product is: cos(α) + cos(β) = 2 cos[(α + β)/2] cos[(α - β)/2].
What is the significance of the four identities discussed in the video?
-The four identities provide essential tools for simplifying and manipulating trigonometric expressions. They are useful for solving problems in both mathematics and physics, particularly in Fourier series and wave analysis.
What strategy does the presenter suggest to simplify trigonometric expressions?
-The presenter suggests memorizing the basic product-to-sum and sum-to-product identities, and then applying these identities to simplify trigonometric expressions by converting them between sum and product forms.
How are angles manipulated when using these identities?
-Angles are manipulated by adding or subtracting them as per the required identity. For example, in the identity sin(α + β), you add the angles α and β. Similarly, for other identities, the angles are adjusted accordingly to fit the form of the identity.
What examples does the presenter provide to demonstrate the use of these identities?
-The presenter demonstrates the use of the identities by solving examples such as sin(75°) + sin(15°), cos(165°) - cos(105°), and other trigonometric expressions using the sum-to-product and product-to-sum identities.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
TRIGONOMETRÍA: Cos (a+b) Demostración de la fórmula Academia DIEGO
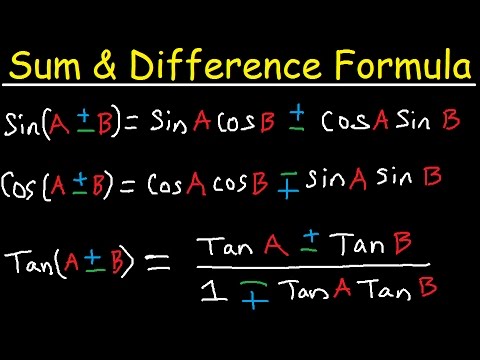
Sum and Difference Identities & Formulas - Sine, Cosine, Tangent - Degrees & Radians, Trigonometry

Identidades trigonométricas, Identidades de simetría e identidades de suma y resta.

10 класс. Алгебра. Тригонометрические уравнения, решаемые разложением левой части на множители

Part 2-Rumus Cosinus Jumlah dan Selisih Dua Sudut

Jumlah dan selisih dua sudut trigonometri matematika kelas XI
5.0 / 5 (0 votes)
