How To Find The LCM of 3 Numbers - Plenty of Examples!
Summary
TLDRThis video teaches how to find the Least Common Multiple (LCM) using two methods: listing multiples and prime factorization. It explains the process step-by-step with examples, such as finding the LCM of 3 and 4, 6 and 9, 24 and 32, and others. The video emphasizes the importance of identifying common multiples and using prime factors to calculate the LCM. Viewers also learn how to check their results by dividing the LCM by the original numbers. This educational video aims to simplify the LCM concept, making it easy to understand for learners of all levels.
Takeaways
- 😀 Listing multiples is a simple method to find the least common multiple (LCM) by comparing common values in each list.
- 😀 The LCM is the smallest number that appears in both lists of multiples.
- 😀 Prime factorization breaks down numbers into their prime components, which helps find the LCM by using the highest powers of each prime factor.
- 😀 For LCM using prime factorization, include the highest number of each prime factor found in any one of the numbers involved.
- 😀 The most common prime factor of a number is used in the LCM calculation based on its highest power.
- 😀 Multiples of smaller numbers like 3 and 4 include values like 12, which can help identify the LCM quickly.
- 😀 By using prime factorization, the LCM of numbers like 6 and 9 can be calculated using 2 threes (3 × 3 = 9).
- 😀 The prime factorization of 24 and 32 leads to an LCM of 96, verified by division or listing the multiples.
- 😀 In cases with multiple numbers, like 12, 15, and 20, prime factorization helps find the LCM by selecting the highest powers of each prime factor.
- 😀 Using prime factorization, the LCM of 8, 10, 12, and 15 can be found by multiplying the highest powers of primes (e.g., 2^3 × 3 × 5 = 120).
- 😀 The LCM can be checked by dividing the candidate LCM by each original number; if all results are whole numbers, the LCM is correct.
Q & A
What is the least common multiple (LCM) of 3 and 4, and how do we find it using the list method?
-The LCM of 3 and 4 is 12. Using the list method, we list the multiples of both numbers: 3 (3, 6, 9, 12, 15, ...) and 4 (4, 8, 12, 16, 20, ...). The common multiples are 12 and 24, with 12 being the least common multiple.
How do you find the LCM of 6 and 9 using prime factorization?
-To find the LCM of 6 and 9, we first find their prime factorizations: 6 = 2 × 3, and 9 = 3 × 3. The LCM requires the highest powers of all primes found in both factorizations. The LCM is 18 (2 × 3 × 3 = 18).
What role does prime factorization play in finding the LCM?
-Prime factorization helps identify the prime factors of each number, and to find the LCM, we select the highest powers of all primes that appear in any of the numbers. This ensures the LCM is divisible by all original numbers.
What is the LCM of 24 and 32, and how is it calculated using prime factorization?
-The LCM of 24 and 32 is 96. The prime factorizations are: 24 = 2 × 2 × 2 × 3 and 32 = 2 × 2 × 2 × 2 × 2. The LCM is found by taking the highest power of 2 (2^5) and multiplying it by the highest power of 3 (3^1), resulting in 96 (2^5 × 3 = 96).
How can we verify that 96 is the LCM of 24 and 32?
-To verify, we divide 96 by both 24 and 32. If the result is a whole number, then 96 is divisible by both numbers and is indeed the LCM. 96 ÷ 24 = 4 and 96 ÷ 32 = 3, both of which are whole numbers.
What is the LCM of 12, 15, and 20, and how is it calculated?
-The LCM of 12, 15, and 20 is 60. The prime factorizations are: 12 = 2 × 2 × 3, 15 = 3 × 5, and 20 = 2 × 2 × 5. The LCM is found by selecting the highest powers of each prime: two 2's, one 3, and one 5. Therefore, 2 × 2 × 3 × 5 = 60.
Why do we choose the highest powers of prime factors when calculating the LCM?
-We choose the highest powers of prime factors to ensure that the LCM is divisible by all the numbers involved. This guarantees the least common multiple is the smallest number that can be evenly divided by all the original numbers.
How do we check if the LCM of 12, 15, and 20 (which is 60) is correct?
-We check by dividing 60 by each of the original numbers: 60 ÷ 12 = 5, 60 ÷ 15 = 4, and 60 ÷ 20 = 3. Since all divisions result in whole numbers, 60 is the correct LCM.
What is the LCM of 8, 10, 12, and 15, and how is it found using prime factorization?
-The LCM of 8, 10, 12, and 15 is 120. The prime factorizations are: 8 = 2 × 2 × 2, 10 = 2 × 5, 12 = 2 × 2 × 3, and 15 = 3 × 5. The LCM is found by taking the highest powers of each prime: three 2's, one 3, and one 5. Therefore, 2 × 2 × 2 × 3 × 5 = 120.
How can we verify that 120 is the LCM of 8, 10, 12, and 15?
-We verify by dividing 120 by each number: 120 ÷ 8 = 15, 120 ÷ 10 = 12, 120 ÷ 12 = 10, and 120 ÷ 15 = 8. Since each division results in a whole number, 120 is confirmed as the LCM.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
How to Find the LCM (2 Different Ways) | Least Common Multiple | Math with Mr. J

MMC - MÍNIMO MÚLTIPLO COMUM
How to Find the LCM using Prime Factorization | Least Common Multiple | Math with Mr. J

minimo comune multiplo (mcm) e Massimo Comun Divisore (MCD): come calcolarli senza confonderli

SOAL CERITA KPK DAN FPB KELAS 5
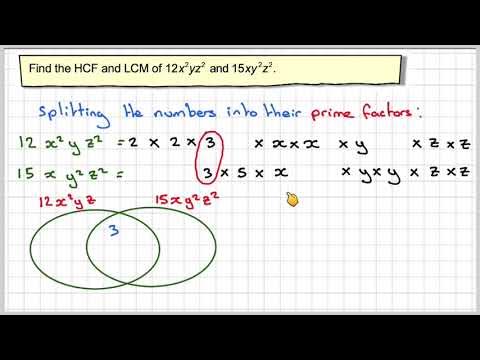
Finding the HCF and LCM of algebraic expressions
5.0 / 5 (0 votes)
