Lecture 1: Basics of Mathematical Modeling
Summary
TLDRThis video introduces the essential principles of mathematical modeling, emphasizing its role in understanding real-world phenomena through abstraction. The process starts by defining the problem and building mathematical models, followed by solving equations and interpreting results. Key steps include validation, which is done through experimentation or intuitive checks against physical laws. The video also discusses the iterative nature of modeling, highlighting the importance of refinement based on feedback and validation. Applications in various fields like epidemiology, biology, and economics are explored to showcase how mathematical modeling enables accurate predictions and optimizations.
Takeaways
- 😀 Mathematical modeling is the process of representing real-world phenomena through mathematical language, such as equations and formulas.
- 😀 Models are used to analyze, predict, and gain insights into real-world systems and behaviors, especially when direct experimentation is impractical.
- 😀 Mathematical models can be physical (e.g., model airplanes) or schematic (e.g., maps) and are commonly applied in fields like biology, engineering, and economics.
- 😀 The purpose of modeling is to understand complex behaviors, predict future outcomes, provide insights into phenomena, and optimize real-world processes.
- 😀 The modeling cycle involves observation of real-world phenomena, conceptualizing a model, mathematical representation, analysis, and validation.
- 😀 Validation is an essential step in ensuring a model’s accuracy by comparing its outcomes with real-world data or checking its logical consistency.
- 😀 If a model's predictions are valid, it is accepted; if not, the model is refined by revisiting the assumptions or parameters.
- 😀 Mathematical models are crucial in various applications, such as predicting disease spread (e.g., COVID-19), optimizing business strategies, and modeling biological systems.
- 😀 The modeling process follows a set of principles: identifying the system, constructing the model, solving the equations, and validating the results.
- 😀 An invalid model may require modifications in its assumptions, parameters, or purpose to improve its alignment with real-world observations.
- 😀 Mathematical modeling is a continuous and iterative process, where models are continuously improved based on feedback and new data.
Q & A
What is a mathematical model?
-A mathematical model is an abstract representation of a real-world phenomenon, expressed in mathematical terms (such as equations, algorithms, or graphs), used to analyze, predict, and understand the behavior of the system or process.
Why are mathematical models important?
-Mathematical models are essential because they help quantify real-world phenomena, providing a more efficient, cost-effective way of studying and predicting behaviors, especially when direct experimentation is challenging or impossible.
Can you list some fields where mathematical models are used?
-Mathematical models are widely used in fields such as epidemiology (disease modeling), biological transport, traffic simulations, economics, engineering (structural modeling), and software development.
What is the general process of mathematical modeling?
-The process of mathematical modeling typically follows these steps: identifying the real-world problem, formulating the model, solving the model mathematically, interpreting the results, and validating the model with real-world data or through intuitive checks.
What is meant by the validation step in mathematical modeling?
-Validation is the process of verifying that the model's predictions match real-world data or expected outcomes. This can be done by comparing the model's results to experimental data or by ensuring the model adheres to known physical laws.
What happens if the model fails validation?
-If the model fails validation, it indicates that the assumptions, parameters, or structure of the model need to be reconsidered. The modeler may need to go back to earlier steps, such as redefining the assumptions or revisiting the purpose of the model.
What are the key assumptions made during mathematical modeling?
-Assumptions in mathematical modeling often involve simplifying the complexities of real-world systems. For example, certain factors may be ignored, or specific relationships might be approximated to make the model solvable and practical.
How can mathematical models be used to predict disease spread?
-Mathematical models can predict the spread of diseases like COVID-19 by using variables such as infection rates, recovery rates, and population density. These models help estimate future outbreaks and assess the impact of different interventions.
What does it mean to interpret the results of a mathematical model?
-Interpreting the results of a mathematical model involves analyzing the outcomes to understand the behavior of the system being modeled. This interpretation helps in making informed decisions or predictions about real-world phenomena.
What is the role of simplifications and assumptions in mathematical modeling?
-Simplifications and assumptions are made to make the problem more manageable. Since real-world systems are often too complex to model in full detail, assumptions help in creating a model that is solvable while still capturing the key features of the system.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

Overview of Object-oriented programming in C# | C# object-oriented programming | C# oops
#01 Fisika Komputasi: Pendahuluan
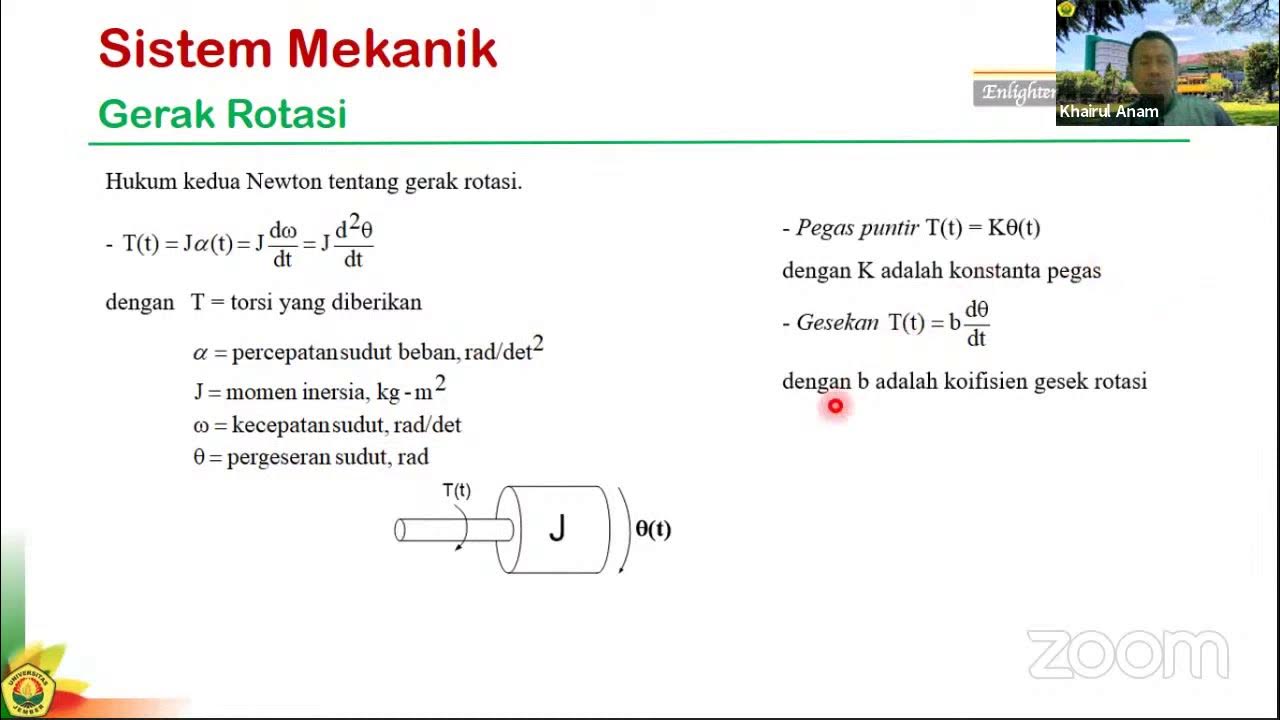
SK#2c: Pemodelan Sistem dengan Persamaan Differensial

5.1 Introduction to Climate Modeling

Bancos de Dados - Aula 01 - Visão geral sobre banco de dados e motivação

OOPS CONCEPTS - JAVA PROGRAMMING
5.0 / 5 (0 votes)
