Moment of Inertia Explained
Summary
TLDRThis engaging discussion on moment of inertia explains its significance in rotational dynamics. It illustrates the concept using simple examples, highlighting how mass distribution affects rotational resistance. By comparing a hoop and a solid disc, the speaker demonstrates that the hoop, with all its mass at a distance from the rotation axis, exhibits a higher moment of inertia. The presentation further explores how the axis of rotation influences stability, revealing that different rotational axes result in varying moments of inertia, ultimately affecting an object's ability to resist changes in motion.
Takeaways
- 🌀 Moment of inertia is defined as the mass times the distance squared from the rotation axis.
- 🌀 Holding an object at its center makes it easier to rotate than holding it at the end due to distribution of mass.
- 🌀 The greater the distance of mass from the rotation axis, the higher the moment of inertia.
- 🌀 A hoop has a larger moment of inertia compared to a solid disc because all its mass is at the maximum distance from the axis.
- 🌀 The moment of inertia influences an object's resistance to rotational acceleration.
- 🌀 Objects with a smaller moment of inertia will accelerate more easily in rotation than those with a larger moment.
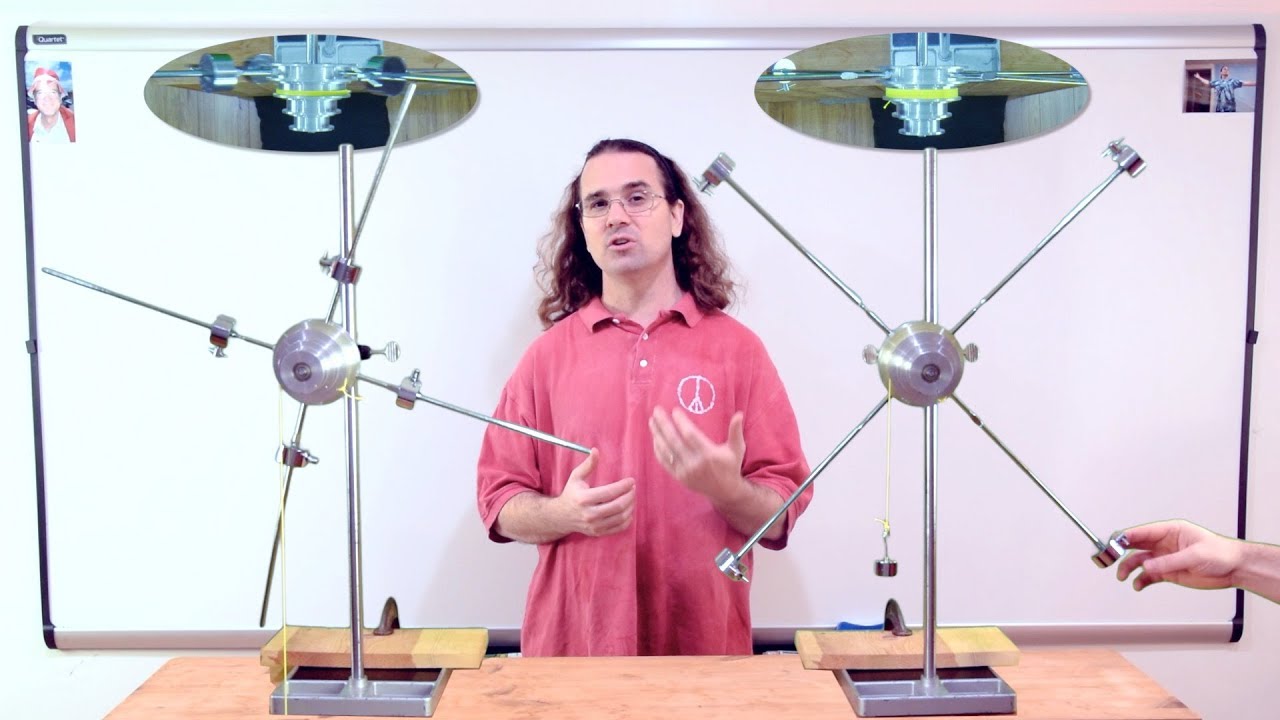
- 🌀 Different axes of rotation can lead to varying moments of inertia for the same object.
- 🌀 An object rotated about an axis with mass distributed diagonally has a larger moment of inertia.
- 🌀 Stability in rotation can vary significantly based on the moment of inertia; objects can be stable or unstable depending on their rotational axis.
- 🌀 Understanding moment of inertia can lead to deeper insights in physics, particularly in dynamics and stability.
Q & A
What is the formula for calculating the moment of inertia?
-The moment of inertia is calculated using the formula I = m * r², where I is the moment of inertia, m is the mass, and r is the distance from the rotation axis.
What does moment of inertia represent?
-Moment of inertia represents an object's resistance to rotational acceleration about a specific axis.
How does the distance from the rotation axis affect moment of inertia?
-The moment of inertia increases as the distance from the rotation axis increases, due to the squared term in the formula, making it significantly more challenging to rotate objects with mass located farther from the axis.
Why is it easier to rotate an object held at its center compared to the end?
-When held at the center, the mass is closer to the rotation axis, resulting in a lower moment of inertia and thus requiring less torque to rotate. When held at the end, the mass is farther away, increasing the moment of inertia and making rotation more difficult.
What is the difference in moment of inertia between a hoop and a solid disc?
-A hoop has all its mass located at a distance R from the rotation axis, resulting in a higher moment of inertia compared to a solid disc, where the mass is distributed evenly and thus has a lower moment of inertia.
What effect does the distribution of mass have on an object's moment of inertia?
-The distribution of mass affects the moment of inertia; mass distributed further from the rotation axis results in a higher moment of inertia, while mass closer to the axis results in a lower moment of inertia.
How can an object be rotated to demonstrate different moments of inertia?
-An object can be rotated along different axes. The moment of inertia will vary based on how the mass is distributed relative to the axis of rotation, with different configurations leading to different levels of stability and resistance to rotation.
What happens when an object is rotated along its axis with the largest moment of inertia?
-When rotated along its axis with the largest moment of inertia, the object is very stable, resisting perturbations and maintaining its rotational motion effectively.
Why is an object less stable when rotated along the axis with the smallest moment of inertia?
-An object rotated along the axis with the smallest moment of inertia is less stable because even small perturbations can easily throw it off its axis of rotation.
What conclusion can be drawn about the moment of inertia and stability?
-The moment of inertia is directly related to an object's stability; higher moments of inertia correlate with greater resistance to changes in rotational motion, while lower moments lead to decreased stability.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

FISIKA KELAS XI || Momen Gaya dan Momen Inersia || DINAMIKA ROTASI DAN KESETIMBANGAN BENDA TEGAR

Dinamika Rotasi • Part 2: Momen Inersia

Mekanika Bahan - Momen Inersia

FISIKA KELAS XI || Momen Gaya dan Momen Inersia || DINAMIKA ROTASI DAN KESETIMBANGAN BENDA TEGAR
Demonstrating Rotational Inertia (or Moment of Inertia)

Tensor Inersia
5.0 / 5 (0 votes)
