Segment Addition Postulate explained with examples
Summary
TLDRThe video provides a clear and concise explanation of the Segment Addition Postulate, a fundamental concept in geometry. The instructor discusses how it applies when a point is between two others on a line, explaining that the sum of the lengths of the segments equals the total distance. Through several examples, the video demonstrates solving equations based on this postulate, emphasizing the importance of setting up part-plus-part-equals-whole equations and plugging in values to find unknown lengths. The lesson also highlights avoiding assumptions based on visual cues and relying on provided information.
Takeaways
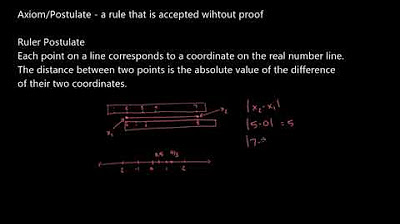
- 📏 The Segment Addition Postulate involves having two points with another point between them, defining the concept of 'betweenness' in geometry.
- 🔗 The postulate states that if a point is between two other points, the sum of the segments formed by these points equals the total length of the segment.
- 🛑 It’s important not to confuse the point between two others as the midpoint unless specified. The point is usually random and not the midpoint.
- ➕ Segment Addition Postulate is expressed as part + part = whole, which can be used to set up equations for problem-solving.
- ✏️ In a practical problem, if one part of the segment is given as a number and another as a variable, you can write an equation and solve for the unknown variable.
- 📝 Always ensure you're solving for the correct quantity, whether it's a variable or the actual segment length, after solving an equation.
- 📐 More complex problems involve algebraic expressions for segment lengths, which can also be solved using the same part + part = whole concept.
- 🧮 Once you find the value of the variable, remember to substitute it back into the expressions to find the actual lengths of the segments.
- 🔍 Be careful not to assume relative sizes based on how the segment is drawn; use the provided information to guide your understanding.
- ✅ The concept of 'part plus part equals the whole' can be extended to problems with more than two segments, as long as all parts sum up to the whole.
Q & A
What is the Segment Addition Postulate?
-The Segment Addition Postulate states that if a point is between two other points on a line segment, the sum of the distances from one point to the middle point and from the middle point to the other point equals the total distance of the segment.
How is 'betweenness' defined in geometry according to the script?
-'Betweenness' in geometry refers to a point being between two other points on the same line segment. A point is only considered 'between' if it lies on the segment connecting the two other points.
Why does the instructor emphasize not placing the middle point directly in the center of the segment?
-The instructor avoids placing the middle point in the center to prevent students from mistakenly thinking it is the midpoint. The point between can be anywhere along the segment and is not necessarily equidistant from the endpoints.
What is the mathematical expression that summarizes the Segment Addition Postulate?
-The mathematical expression for the Segment Addition Postulate can be summarized as 'part + part = whole,' meaning the sum of the two segment parts equals the total length of the segment.
How would you solve for a missing length using the Segment Addition Postulate?
-To solve for a missing length, set up an equation where the known lengths of the parts are added together to equal the total segment length. For example, if one part is 10 and another is x, and the total length is 17, the equation would be 10 + x = 17, then solve for x.
In the context of the segment AB + BC = AC, what does the notation AB or AC mean?
-The notation AB or AC without any symbol over it refers to the length or distance between points A and B or A and C, respectively.
How would you approach a problem with algebraic expressions for segment lengths?
-For algebraic expressions, apply the Segment Addition Postulate by writing an equation where the sum of the algebraic parts equals the whole. For example, if one part is 2x + 1, another is 5x, and the total length is 29, the equation would be (2x + 1) + 5x = 29. Combine like terms and solve for x.
What is the process after solving for the variable x in an algebraic problem?
-After solving for x, substitute the value of x back into the expressions for the segment lengths to find the actual lengths of the individual parts. Always check if the problem requires the value of x or the actual segment length.
What should you remember about segment lengths in geometry when interpreting diagrams?
-In geometry, you cannot assume one segment is longer or shorter based solely on appearance. Rely on given information, such as measurements or expressions, rather than visual assumptions.
How can you check your solution in a segment addition problem?
-You can check your solution by adding the calculated lengths of the segment parts and verifying that their sum matches the total length given in the problem.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenant5.0 / 5 (0 votes)