Trinomials (M2 2.3 Lesson)

Summary

The script is a tutorial on factoring polynomials, emphasizing the importance of understanding the underlying principles before using shortcuts. It introduces a method for factoring trinomials by finding two numbers that multiply to the product of the leading coefficient and constant term, and add up to the linear coefficient. The process involves splitting the middle term and using factoring by grouping. The script also covers factoring when the leading and constant terms are perfect squares and extends the technique to expressions with higher powers. It concludes with a problem-solving example, illustrating how to apply the method to solve equations.

Takeaways

• 📚 The video script is a tutorial on factoring polynomials, emphasizing the importance of understanding the underlying principles before using shortcuts.
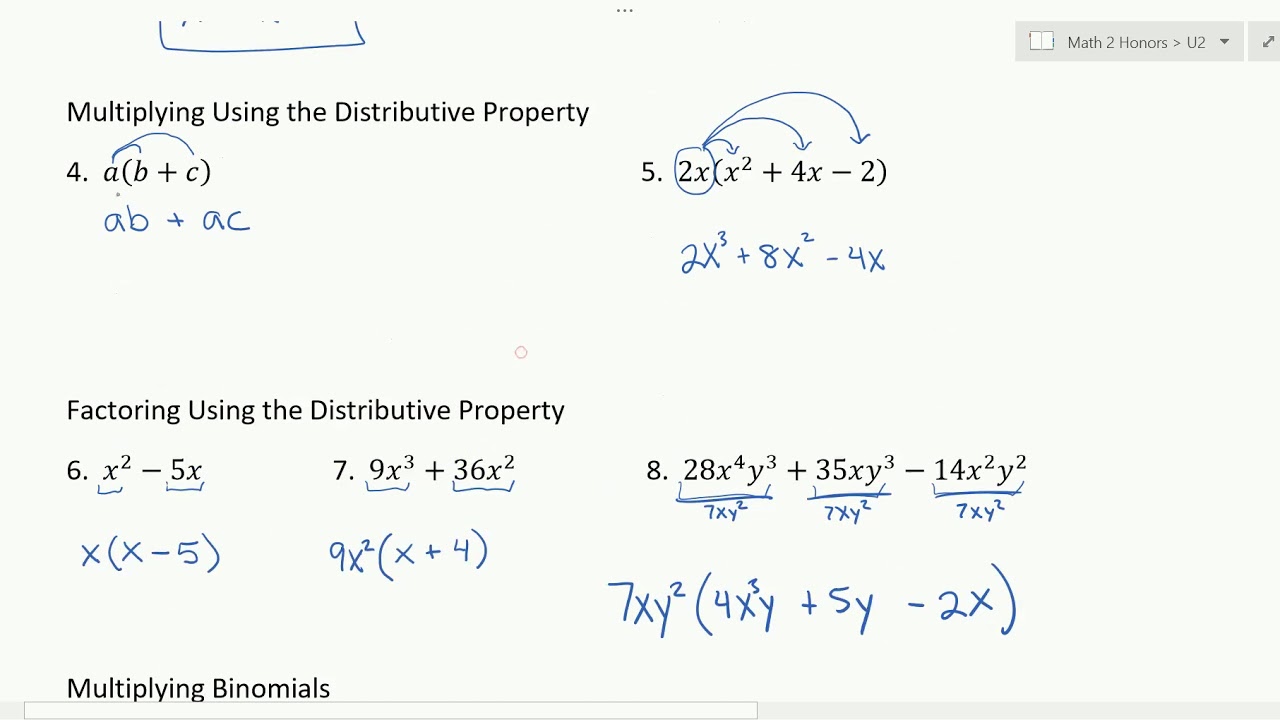
• 🔑 The first method discussed is factoring out the Greatest Common Factor (GCF), which involves identifying a common factor in all terms of a polynomial.
• 🔍 The second method involves a strategic approach to factoring trinomials with three terms, where the script introduces a game-like method to find two numbers that meet specific multiplication and addition criteria.
• 📈 The tutorial explains how to use the coefficients of the polynomial to guide the factoring process, particularly focusing on the lead coefficient, constant term, and linear coefficient.
• 🎯 The script provides a step-by-step guide on how to split the middle term of a trinomial based on the two numbers identified, which is crucial for the factoring process.
• 🔄 The concept of 'factoring by grouping' is introduced as a method to simplify polynomials once they are expanded to four terms through strategic splitting.
• 📝 The tutorial emphasizes the importance of checking work by multiplying the factored form back to its original polynomial form to ensure accuracy.
• 🧩 The script discusses strategies for dealing with larger numbers and perfect squares in polynomials, suggesting tricks like prime factorization and recognizing patterns.
• 🔢 The video also touches on factoring polynomials that are not quadratic, such as those with terms raised to the sixth power, showing adaptability in the factoring techniques.
• 📉 The tutorial concludes with a problem-solving example, demonstrating how to apply the learned factoring methods to solve equations using the zero product property.

Q & A

What is the first type of factoring mentioned in the script?

The first type of factoring mentioned is factoring out the Greatest Common Factor (GCF).

Why is it important to understand why shortcuts work before using them?

It's important to understand why shortcuts work to ensure you're using them correctly and to apply them effectively in more complex problems.

What is the second type of factoring discussed in the script?

The second type of factoring discussed is factoring by grouping, which is typically applied when there are an even number of terms.

Why can't the method of factoring by grouping be used for three terms?

Factoring by grouping cannot be used for three terms because it requires an even number of terms to be split into pairs.

How does the script suggest finding two numbers for factoring when the terms are not easily divisible?

The script suggests using prime factorization and grouping factors in sets of two to find two numbers that multiply to a given product and add to a given sum.

What is the significance of the numbers 18 and 2 in the script?

The numbers 18 and 2 are found to be the two numbers whose product is 36 (3 times 12) and whose sum is 20, which allows for the successful factoring of the given expression.

How does the script recommend checking the factoring work?

The script recommends checking the factoring work by multiplying the factored terms and comparing them to the original expression.

What is the strategy for factoring when the numbers are large or not easily factorable?

The strategy is to look for patterns, such as perfect squares, and use prime factorization to break down the numbers into manageable parts.

Why is it important to match the terms when splitting up the middle term during factoring?

It's important to match the terms to ensure that the factoring process is mathematically correct and that the terms can be grouped properly for further factoring.

What does the script suggest for factoring expressions that are not quadratic?

The script suggests that the same factoring techniques can be applied to non-quadratic expressions by making necessary adjustments, such as ensuring the terms match when splitting.

How does the script demonstrate solving a problem using factoring?

The script demonstrates solving a problem by first setting the equation to zero, then factoring, and finally using the zero product property to find the solutions.