Identitas Trigonometri | Matematika Wajib Kelas X
Summary
TLDRIn this video, Deni Handayani discusses basic trigonometric identities, covering reciprocal identities, ratio identities, and Pythagorean identities. He explains how these identities relate to the six main trigonometric functions: sine, cosine, tangent, cosecant, secant, and cotangent. Through examples, he demonstrates how to simplify trigonometric expressions using these identities, such as simplifying fractions and applying the Pythagorean theorem. The video provides viewers with a clear and structured approach to understanding foundational trigonometric principles.
Takeaways
- 😀 The video introduces basic trigonometric identities, focusing on three main types: reciprocal identities, ratio identities, and Pythagorean identities.
- 😀 The reciprocal identities describe relationships such as sin being the inverse of cosecant, cos being the inverse of secant, and tan being the inverse of cotan.
- 😀 Ratio identities show relationships between different trigonometric functions, like tan being equal to sin divided by cos, and cotan being the inverse of tan.
- 😀 The Pythagorean identity is based on the Pythagorean theorem, with sin²(α) + cos²(α) = 1.
- 😀 By dividing the Pythagorean identity by cos²(α), you can derive the identity tan²(α) + 1 = sec²(α).
- 😀 Dividing the Pythagorean identity by sin²(α) leads to the identity cot²(α) + 1 = cosec²(α).
- 😀 The video uses various examples to demonstrate how these identities simplify trigonometric expressions.
- 😀 It also covers the step-by-step process of simplifying trigonometric expressions using these identities, such as converting sin, cos, tan, and sec functions.
- 😀 The video emphasizes the importance of understanding the relationships between different trigonometric functions to simplify problems.
- 😀 At the end, the video provides a summary of key trigonometric identities and encourages viewers to practice solving related problems for a better grasp of the material.
Q & A
What are the three main trigonometric identities discussed in this video?
-The three main trigonometric identities discussed in the video are the reciprocal identities, the ratio identities, and the Pythagorean identities.
Can you explain the reciprocal identities mentioned in the video?
-The reciprocal identities are: sine (sin) is the reciprocal of cosecant (csc), cosine (cos) is the reciprocal of secant (sec), and tangent (tan) is the reciprocal of cotangent (cot). For example, sin(α) = 1/csc(α), cos(α) = 1/sec(α), and tan(α) = 1/cot(α).
What are the ratio identities, and how do they relate to sine and cosine?
-The ratio identities express tangent (tan) as the ratio of sine to cosine. Specifically, tan(α) = sin(α)/cos(α), and cotangent (cot) is the inverse of tangent, which is cot(α) = cos(α)/sin(α).
What is the Pythagorean identity in trigonometry?
-The Pythagorean identity states that sin²(α) + cos²(α) = 1, which is derived from the Pythagorean theorem applied to a right triangle. This identity is fundamental in simplifying trigonometric expressions.
How can we derive other identities from the Pythagorean identity?
-By dividing both sides of the Pythagorean identity by sin²(α), cos²(α), or another trigonometric function, we can derive other useful identities, such as tan²(α) + 1 = sec²(α) and 1 + cot²(α) = csc²(α).
What is the significance of the identity sin²(α) + cos²(α) = 1?
-This identity is crucial in trigonometry because it allows simplification of expressions and helps in transforming one trigonometric function into another. It also serves as the basis for deriving other trigonometric identities.
In the example problems, how is sin(x) * cos(x) simplified?
-In the example, sin(x) * cos(x) * tan(x) / csc(x) is simplified by recognizing that tan(x) = sin(x)/cos(x) and csc(x) = 1/sin(x), which leads to the simplification of the expression to sin(x) * cos(x).
What is the result when sin²(x) + cos²(x) is divided by tan²(x) - sec²(x)?
-The result of sin²(x) + cos²(x) divided by tan²(x) - sec²(x) is 1, as sin²(x) + cos²(x) equals 1, and tan²(x) - sec²(x) simplifies based on trigonometric identities.
How is the expression cos(x) + cot(x) * (1 - cos(x)) simplified?
-The expression cos(x) + cot(x) * (1 - cos(x)) simplifies by expanding the terms and applying trigonometric identities, eventually simplifying to a fraction with sin(x) and cos(x) terms.
What identity is used to simplify the expression tan²(x) - sec²(x) / -sec²(x)?
-The expression tan²(x) - sec²(x) / -sec²(x) is simplified using the identity tan²(x) = sec²(x) - 1, resulting in sec²(x) as the final answer.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

Simplifying Trigonometric Expressions
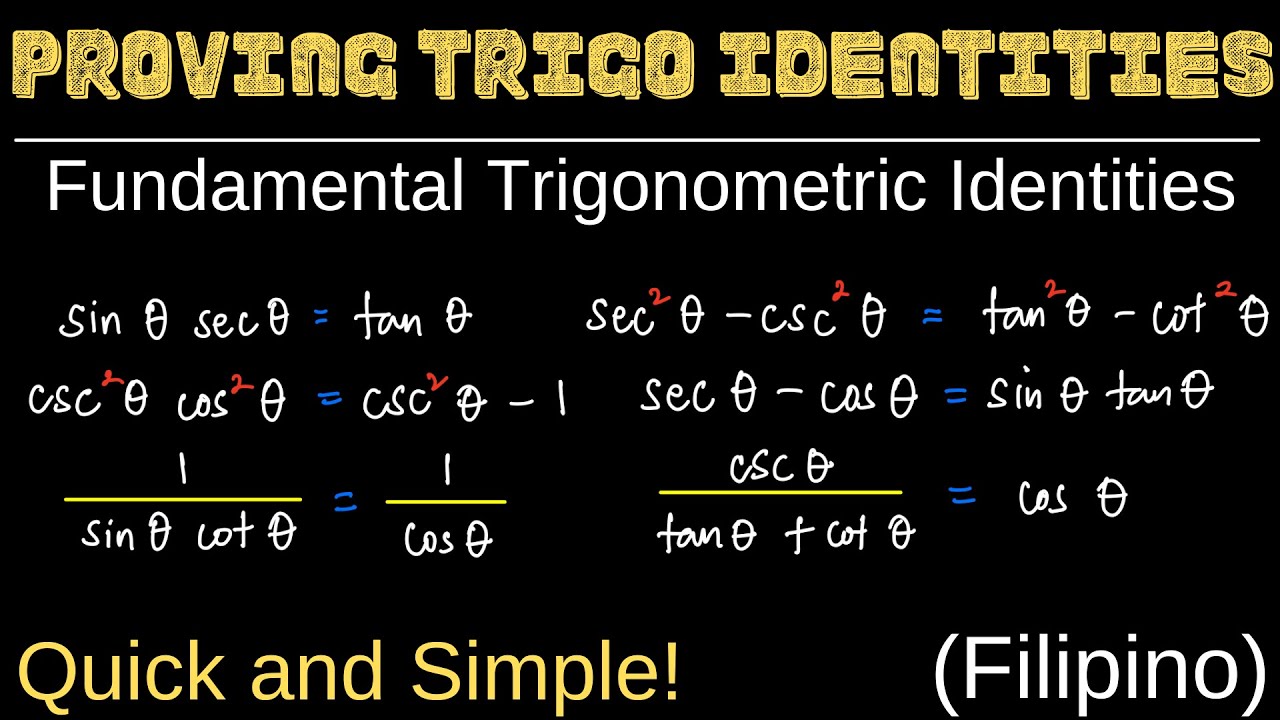
Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Sample Problems
Proving Trigonometric Identities (Tagalog/Flipino Math)

[Math 20] Lec 3.4 Fundamental Identities (1 of 2)

Identitas Trigonometri: Identitas Kebalikan, Perbandingan dan Pythagoras - SMA Kelas 10
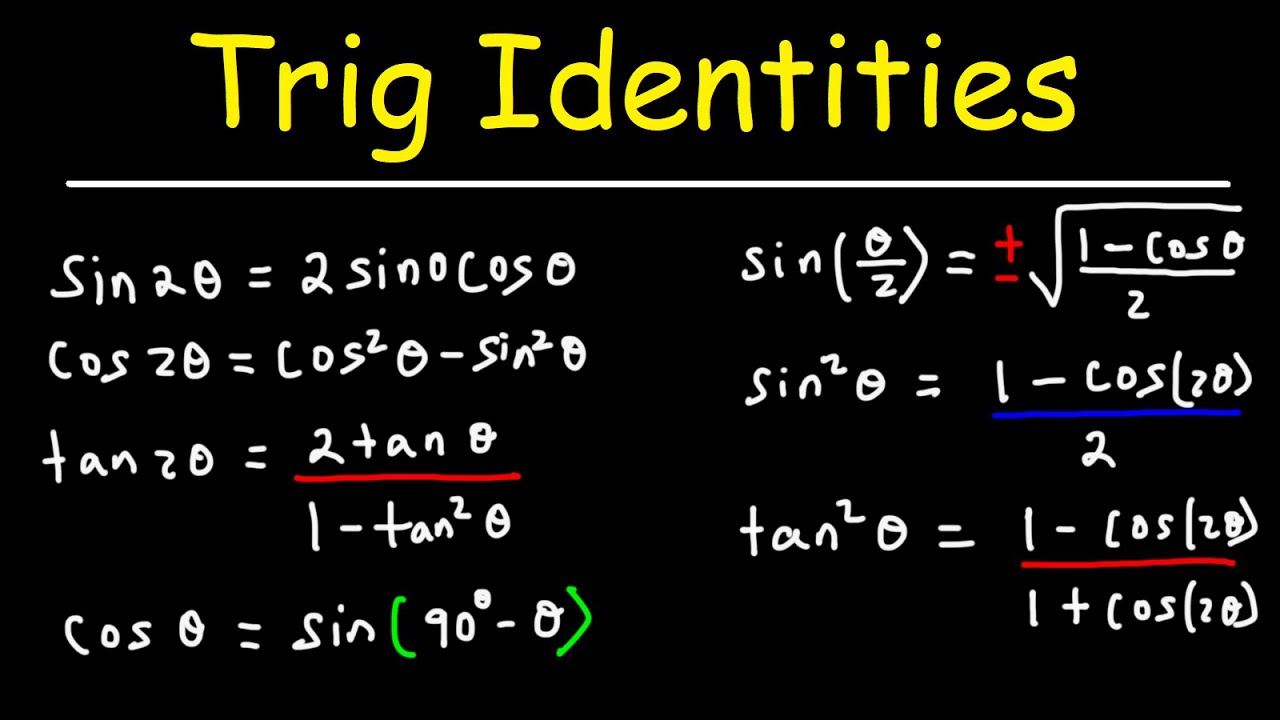
Trig Identities
5.0 / 5 (0 votes)
