A2 Focus and Directrix
Summary
TLDRThis video tutorial explores the concept of parabolas, focusing on the relationship between the focus, directrix, and vertex. It introduces the distance formula as a key tool for deriving the equations of vertical and horizontal parabolas. The instructor provides clear examples, illustrating how to determine the p-value, which indicates the distance from the vertex to the focus or directrix. By visually representing parabolas and their components, the lesson helps viewers understand how to graph these curves and formulate their equations effectively.
Takeaways
- 😀 The focus and directrix are fundamental components in understanding the shape and properties of a parabola.
- 📐 The distance formula is essential for deriving the vertex form of a quadratic equation and determining the parabola's characteristics.
- 🔍 The value of 'p' represents the distance from the vertex to the focus (positive) or from the vertex to the directrix (negative).
- ⬆️ For vertical parabolas, the equation is generally in the form of 'y = (1/4p)x²', while horizontal parabolas use 'x = (1/4p)y²'.
- 📈 The vertex of a parabola is located at the midpoint between the focus and the directrix.
- 👁️ The parabola forms an 'umbrella' shape around the focus, opening away from the directrix.
- ✏️ When given a parabola's focus and directrix, you can calculate the vertex and formulate the parabola's equation.
- 🔄 The axis of symmetry is a vertical or horizontal line that divides the parabola into two equal halves.
- 📝 When converting a given equation to the standard form, you can derive the vertex and p-value to analyze the parabola's properties.
- 💡 Identifying whether a parabola opens upwards, downwards, left, or right is crucial for correctly applying the distance formula.
Q & A
What is the primary formula used for understanding parabolas?
-The primary formula is the distance formula, which is essentially the vertex form of a quadratic equation.
What does the variable 'p' represent in the context of a parabola?
-'p' represents the distance from the vertex to the focus, or the negative distance from the vertex to the directrix.
How can you find the p-value for a parabola with a vertex at (0, 0) and a focus at (0, 2)?
-The p-value can be found by measuring the distance from the vertex to the focus, which in this case is 2 units up, so p = 2.
What is the relationship between the vertex, focus, and directrix of a parabola?
-The vertex is always the halfway point between the focus and the directrix.
How do you identify the direction in which a parabola opens?
-If the p-value is positive, the parabola opens upward (vertical), and if it is negative, it opens downward. For horizontal parabolas, the direction depends on the sign of the p-value relative to the x-axis.
What is the equation of a vertical parabola with a vertex at (1, 1) and a focus at (1, -1)?
-The equation is y = -1/8(x - 1)² + 1, with a p-value of -2.
In the context of horizontal parabolas, how does the equation differ?
-For horizontal parabolas, the formula changes to x = (1/4p)y², as opposed to the vertical form y = (1/4p)x².
How do you determine the directrix of a parabola given its vertex and focus?
-The directrix can be found by measuring the same distance as p from the vertex, but in the opposite direction of the focus.
What steps should be taken when given an equation to find the vertex and p-value?
-Rearrange the equation to isolate either x or y, identify the vertex, and match the equation to the distance formula to solve for p.
Why is it important to understand the umbrella-like shape of a parabola?
-The umbrella shape illustrates how the parabola forms around the focus and moves away from the directrix, helping visualize the geometric properties of parabolas.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados
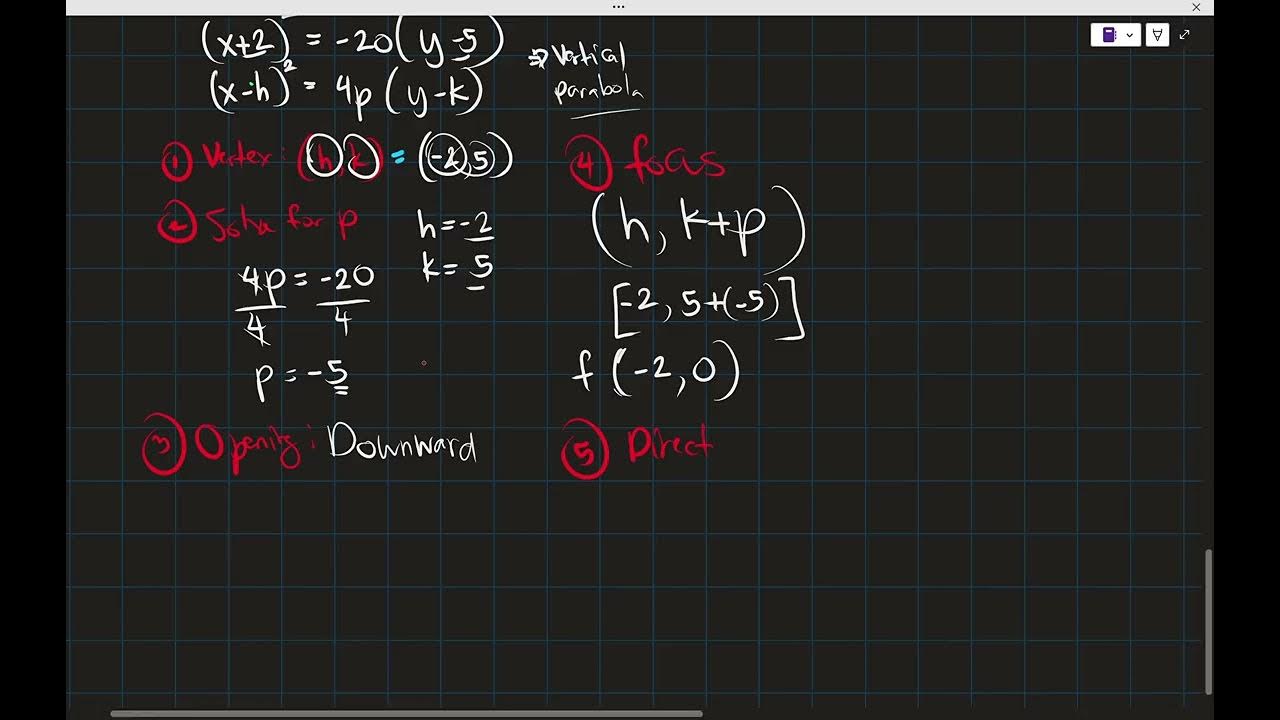
Review on Parabola

Pre-Calculus - Parabola : If the Vertex is (0,0) find the Focus, Directrix, Axis of Symmetry, Latus
Conic Sections - Circles, Semicircles, Ellipses, Hyperbolas, and Parabolas
part 1 materi irisan kerucut parabola kelas 12 materi kelas 12 kurikulum merdeka geometri analitik

Parábolas con albanene.wmv
Pre Calculus - Graph of Parabola | Finding Vertex, Focus, Directrix and Axis of Symmetry of Parabola
5.0 / 5 (0 votes)
