HOW TO SOLVE GOLDEN RATIO (MADE EASY)

Summary

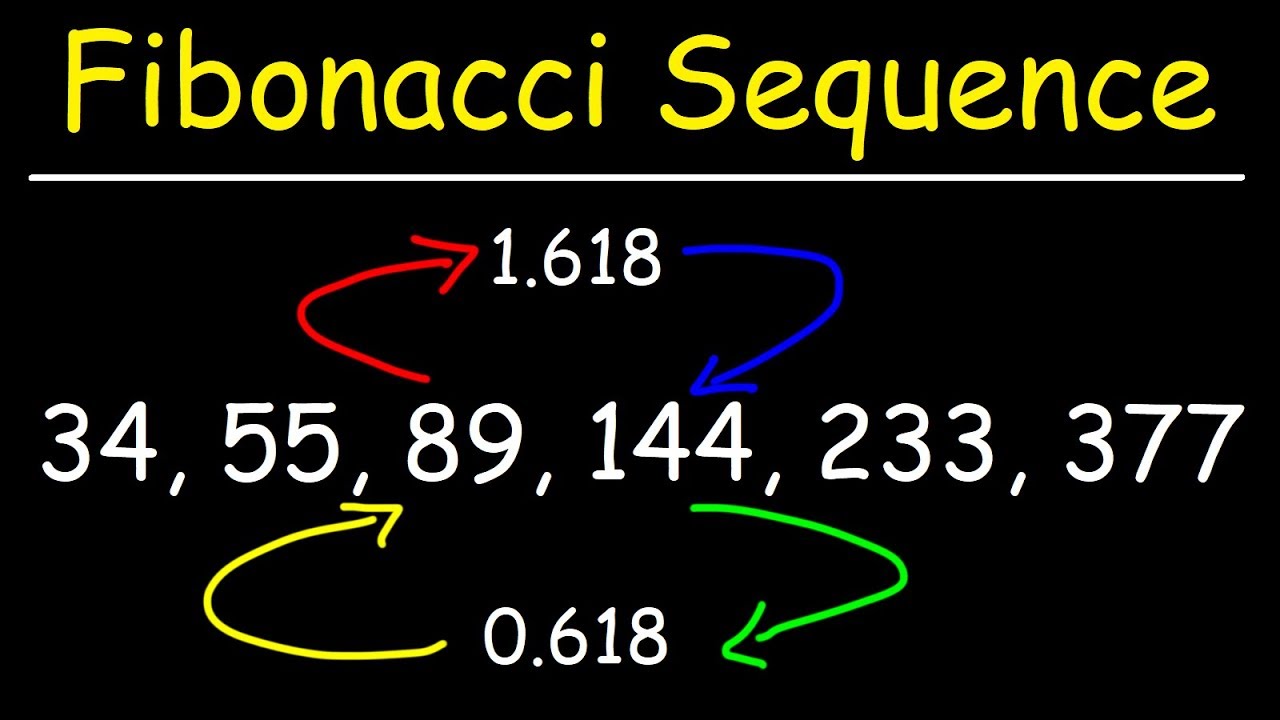
The video explains the concept of the golden ratio by showing how two numbers are in golden ratio if they satisfy the equation a/b = (a + b)/a, where a is greater than b. The examples 55 and 34, as well as 377 and 233, are used to demonstrate this principle. Calculations confirm that these pairs yield the same result (1.62) on both sides of the equation, indicating they are in golden ratio. If the results differ, such as 1.8, the numbers would not be in golden ratio.

Takeaways

• 🔢 The golden ratio is defined by the equation a/b = (a + b)/a, where a is greater than b and both are positive.
• 🔍 The golden ratio formula states that a is always greater than b.
• 📊 Example 1: Given 34 and 55, a is 55 and b is 34 because a must be larger.
• 🧮 Using the golden ratio formula, 55/34 = (55 + 34)/55, which simplifies to 1.62 = 1.62, confirming the golden ratio.
• 📏 The pair 55 and 34 satisfy the golden ratio condition.
• 📝 Example 2: Given 233 and 377, a is 377 and b is 233 because a is larger.
• 🔢 In this example, 377/233 = (377 + 233)/377, which again simplifies to 1.62 = 1.62, confirming the golden ratio.
• 🔗 Both 377 and 233 are in the golden ratio.
• ⚖️ If the two sides of the equation are not equal, such as 1.62 ≠ 1.8, then the numbers are not in the golden ratio.
• ✅ The golden ratio condition is satisfied when the values on both sides of the equation are the same.

Q & A

What is the definition of the golden ratio?

The golden ratio is a special number approximately equal to 1.618, and it is found by dividing a line into two parts so that the whole length divided by the long part is equal to the long part divided by the short part.

What are the conditions for two numbers to be in the golden ratio?

For two numbers to be in the golden ratio, the larger number divided by the smaller number must be equal to the sum of the two numbers divided by the larger number, with the larger number being greater than the smaller number and both numbers being positive.

In the example given, what are the values of a and b for the numbers 34 and 55?

In the example, a is 55 and b is 34 because a is always greater than b.

How do you verify if 55 and 34 are in the golden ratio?

You verify by checking if 55/34 is approximately equal to (55 + 34)/55. Both calculations yield approximately 1.618, confirming that 55 and 34 are in the golden ratio.

What are the values of a and b for the numbers 233 and 377?

In the example, a is 377 and b is 233 because a is always greater than b.

How do you verify if 233 and 377 are in the golden ratio?

You verify by checking if 377/233 is approximately equal to (377 + 233)/377. Both calculations yield approximately 1.618, confirming that 233 and 377 are in the golden ratio.

If the result of the calculation on one side is 1.8, what does it indicate about the numbers being in the golden ratio?

If the result of the calculation on one side is 1.8, it indicates that the numbers are not in the golden ratio because 1.8 is not approximately equal to 1.618.

What is the approximate value of the golden ratio?

The approximate value of the golden ratio is 1.618.

Can you provide another example of two numbers in the golden ratio?

Yes, another example of two numbers in the golden ratio could be 89 and 55, as 89/55 is approximately equal to (89 + 55)/89, both yielding approximately 1.618.

What is the significance of the golden ratio in mathematics and art?

The golden ratio is significant in mathematics and art because it is aesthetically pleasing and appears frequently in nature, art, and architecture. It is often used to create proportions that are considered beautiful and harmonious.