FISIKA KUANTUM : KENAPA FUNGSI GELOMBANG HARUS DI NORMALISASI?
Summary
TLDRThis video delves into the concept of wave function normalization in quantum physics. The creator explains why wave functions need to be normalized and their significance in making Schrodinger's equation solutions physically meaningful. By discussing Max Born's statistical interpretation, the video illustrates how the integral of the squared wave function determines the probability of locating a particle in a given region. If the integral over all space equals one, the wave function is considered normalized. Future videos will cover the detailed process of wave function normalization.
Takeaways
- 🔔 Make sure to subscribe and share the video to get more content on quantum physics.
- 🔍 The video explains the concept of wave function normalization, essential in quantum mechanics.
- 📚 Understanding wave function normalization helps clarify why only one solution of the Schrödinger equation represents a physical reality.
- 🧠 Max Born's statistical interpretation states that the square of the wave function gives the probability of finding a particle in a specific region.
- 📈 Mathematically, this probability is obtained by integrating the square of the wave function over a given range.
- 🌍 For a realistic physical interpretation, the probability of finding a particle in the entire space must equal one.
- 📝 Not all solutions to the Schrödinger equation meet the physical criteria, which is why normalization is crucial.
- 📊 Normalization involves multiplying the wave function by a constant to ensure it meets Max Born's statistical interpretation.
- ❌ Without normalization, the wave function lacks physical meaning and doesn't accurately represent a particle.
- 🔜 The video promises a detailed explanation on how to normalize a wave function in a future video.
Q & A
What is the purpose of normalizing a wave function in quantum mechanics?
-Normalizing a wave function ensures that the total probability of finding a particle in all possible positions is equal to one, in line with the statistical interpretation of quantum mechanics.
What does the square of the wave function represent?
-The square of the wave function, when integrated over a given range, represents the probability of finding a particle in that range.
Why is it necessary to normalize the solutions to Schrödinger's equation?
-Schrödinger's equation can have many mathematical solutions, but not all of them provide a physically meaningful interpretation of particle probability. Normalization helps identify the correct solution by ensuring the probability interpretation is satisfied.
What does the process of integrating the wave function squared represent in physical terms?
-Integrating the wave function squared over a specific range gives the probability of finding a particle within that range, corresponding to the area under the curve between two points.
What is the physical significance of a wave function that is not normalized?
-A non-normalized wave function doesn't accurately represent the probability of finding a particle, making it a purely mathematical solution without physical meaning.
What is Max Born’s statistical interpretation of the wave function?
-Max Born's statistical interpretation suggests that the wave function squared gives the probability density of finding a particle in a specific region of space.
What is the integral of the wave function squared over all space supposed to equal?
-The integral of the wave function squared over all space must equal one, representing the certainty that the particle exists somewhere in space.
What does it mean if the integral of the wave function squared is not equal to one?
-If the integral is not equal to one, it means that the wave function does not properly represent the total probability of finding a particle, and therefore it needs to be normalized.
How can a wave function be normalized?
-A wave function can be normalized by multiplying it by a constant, such that the integral of its square over all space equals one.
What happens if a wave function is not normalized in quantum mechanics?
-If a wave function is not normalized, it cannot correctly represent the physical reality of a particle's position probability, making it only a mathematical solution with no physical interpretation.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

Quantum Entanglement: Spooky Action at a Distance

Why We Need the Normalization Condition in Quantum Mechanics

Statistical Interpretation of Quantum Mechanics
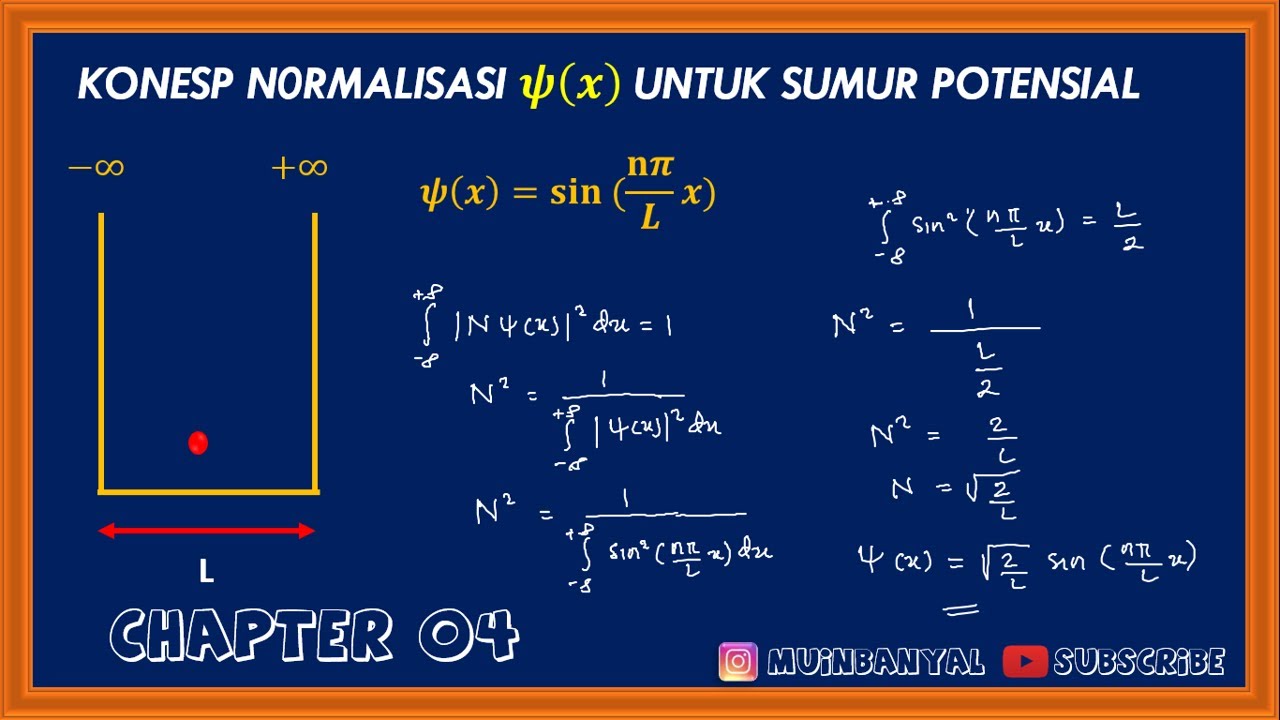
FISIKA KUANTUM : KONSEP NORMALISASI UNTUK SUMUR POTENSIAL
Física quântica - Efeito fotoelétrico Parte 2 de 2

The Many Worlds of the Quantum Multiverse
5.0 / 5 (0 votes)
