Trigonometry made easy
Summary
TLDRThis Tech Math Channel video introduces trigonometry, focusing on its application to right-angle triangles. The host explains the fundamental relationships between triangle sides and angles, using the example of an angle labeled Theta. Key concepts include labeling sides as opposite, adjacent, and hypotenuse, and using trigonometric functions like sine, cosine, and tangent to solve for unknown angles or side lengths. The video simplifies complex concepts with mnemonics and practical examples, demonstrating how to calculate and use inverse functions on a calculator to find angles from side lengths.
Takeaways
- 📚 Trigonometry is a branch of mathematics that studies the relationships between the sides and angles of a right-angle triangle.
- 🔢 The three primary trigonometric functions are sine (sin), cosine (cos), and tangent (tan), which relate the angles and side lengths of a right-angle triangle.
- 📐 The sides of a right-angle triangle are labeled as the hypotenuse (opposite the right angle), the opposite side (opposite the angle in question), and the adjacent side (next to the angle in question).
- 💡 The sine function is defined as the ratio of the opposite side to the hypotenuse, cosine as the adjacent side to the hypotenuse, and tangent as the opposite side to the adjacent side.
- 🧠 Memorizing the acronym SOHCAHTOA can help recall the trigonometric functions: Sine = Opposite/Hypotenuse, Cosine = Adjacent/Hypotenuse, Tangent = Opposite/Adjacent.
- 🔄 To solve for an unknown side length, one can use the appropriate trigonometric function based on the given angle and known side lengths.
- 🔢 Using a calculator, one can find the value of the trigonometric functions for specific angles and then solve for the unknown side lengths.
- 🔄 To find an unknown angle given two side lengths, use the inverse trigonometric functions (e.g., sin^-1, cos^-1) available on most calculators.
- 📝 The process involves labeling the sides, selecting the correct trigonometric function, substituting the known values, and then solving for the unknown using basic algebraic manipulations.
- 👨🏫 The video script provides practical examples demonstrating how to apply trigonometry to solve for unknown sides and angles in right-angle triangles.
Q & A
What is the main focus of the video?
-The main focus of the video is to explain trigonometry, specifically its application to right-angle triangles, and how it can be used to find unknown angles or side lengths.
What are the three sides of a right-angle triangle called?
-The three sides of a right-angle triangle are called the hypotenuse, the opposite side, and the adjacent side.
What are the three primary trigonometric functions?
-The three primary trigonometric functions are sine (sin), cosine (cos), and tangent (tan).
How are the trigonometric functions related to the sides of a right-angle triangle?
-The trigonometric functions relate to the sides of a right-angle triangle as follows: sine is the ratio of the opposite side to the hypotenuse, cosine is the ratio of the adjacent side to the hypotenuse, and tangent is the ratio of the opposite side to the adjacent side.
What is the mnemonic provided in the video to remember the trigonometric functions?
-The mnemonic provided in the video to remember the trigonometric functions is 'SOHCAHTOA', which stands for Sine equals Opposite over Hypotenuse, Cosine equals Adjacent over Hypotenuse, and Tangent equals Opposite over Adjacent.
How does the video suggest solving for an unknown side length using trigonometry?
-The video suggests first labeling the sides as opposite, adjacent, or hypotenuse, then choosing the appropriate trigonometric function based on the given angle and known side, and finally substituting the values into the function to solve for the unknown side length.
What is the process for finding an unknown angle when two side lengths are known?
-To find an unknown angle when two side lengths are known, one must first label the sides, then use the appropriate trigonometric function (sine or cosine), calculate the ratio, and finally use the inverse function on a calculator to find the angle.
How does the video demonstrate solving for an unknown side length using the sine function?
-The video demonstrates solving for an unknown side length using the sine function by providing an example with a 35° angle and a hypotenuse of 12 units, then using the sine function to calculate the opposite side length as 6.88 units.
What is the role of the inverse trigonometric functions in solving for angles?
-The inverse trigonometric functions, such as sin^-1 or cos^-1, are used to find the angle when the ratio of the sides is known. They are essential for converting back from the ratio to the actual angle measure.
How does the video recommend practicing and mastering trigonometry?
-The video recommends practicing and mastering trigonometry by solving various problems, understanding the relationships between the sides and angles, and getting familiar with the functions and how to use them on a calculator.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

TRIGONOMETRI - Ukuran Sudut dan Perbandingan Trigonometri

SOHCAHTOA using the TI-84 Plus
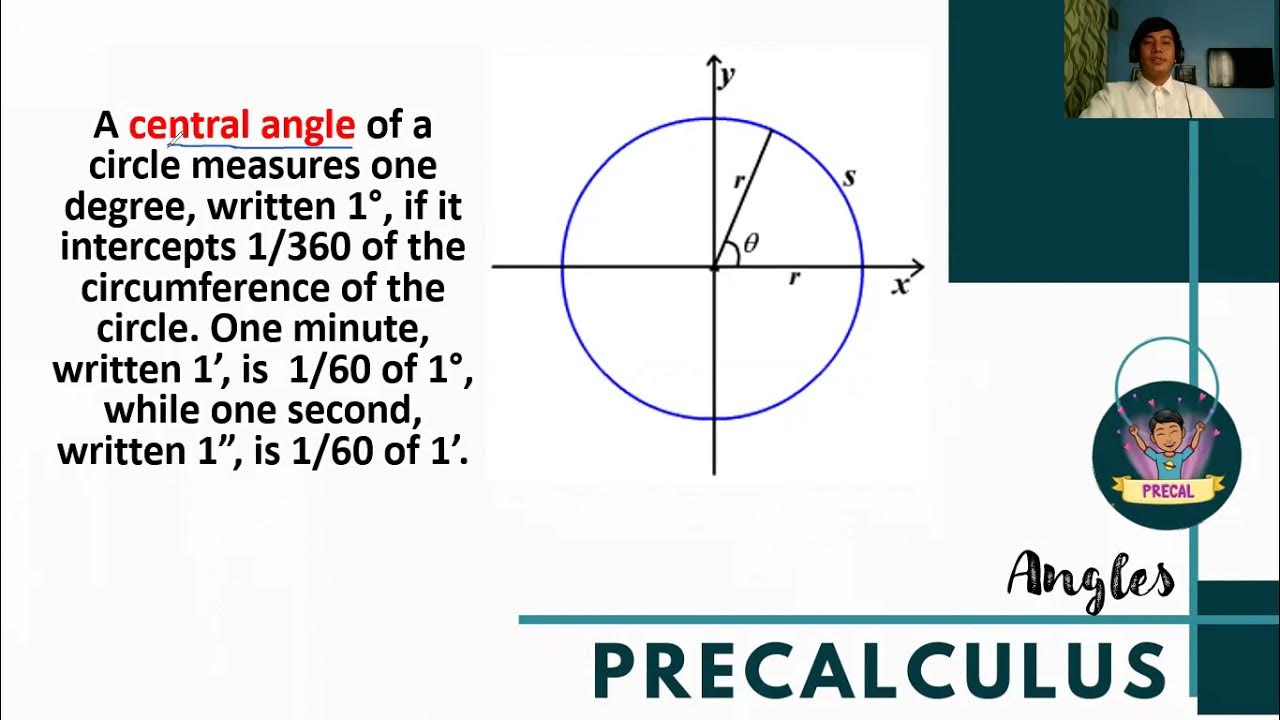
Angle Measure (Precalculus)

Perbandingan Trigonometri Pada Segitiga Siku-siku - Matematika Wajib Kelas X

Matematika SMA - Trigonometri (7) - Trigonometri Aturan Sinus dan Cosinus (A)
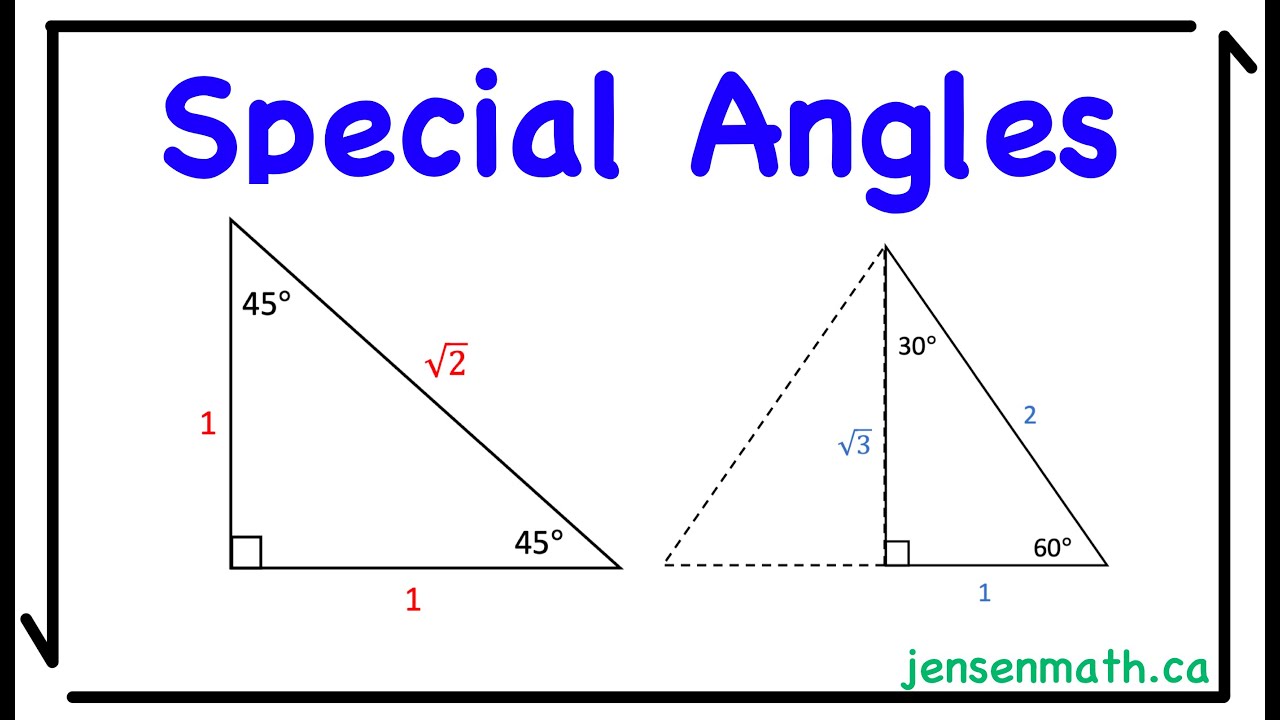
Special Triangles (full lesson) | jensenmath.ca
5.0 / 5 (0 votes)
