What is the Heisenberg Uncertainty Principle? - Chad Orzel

Summary

The Heisenberg Uncertainty Principle, a cornerstone of quantum mechanics, posits that it's impossible to know both the exact position and momentum of a particle simultaneously. This principle arises from the dual particle-wave nature of matter, where particles exhibit wave-like properties with wavelength related to momentum. The more precisely the position is measured, the less certain the momentum becomes, and vice versa. This isn't a measurement issue but a fundamental aspect of quantum physics, illustrating the inherent uncertainty in the properties of particles at the subatomic level.

Takeaways

• 🌌 The Heisenberg Uncertainty Principle is a fundamental concept in quantum physics that has permeated popular culture, symbolizing inherent uncertainty.
• 🔍 It asserts that it's impossible to know both the exact position and exact speed (momentum) of a particle simultaneously.
• 🌊 The principle arises because quantum objects exhibit wave-particle duality, behaving as both a particle and a wave.
• 📊 In quantum mechanics, the exact position and momentum of an object are not well-defined until measured.
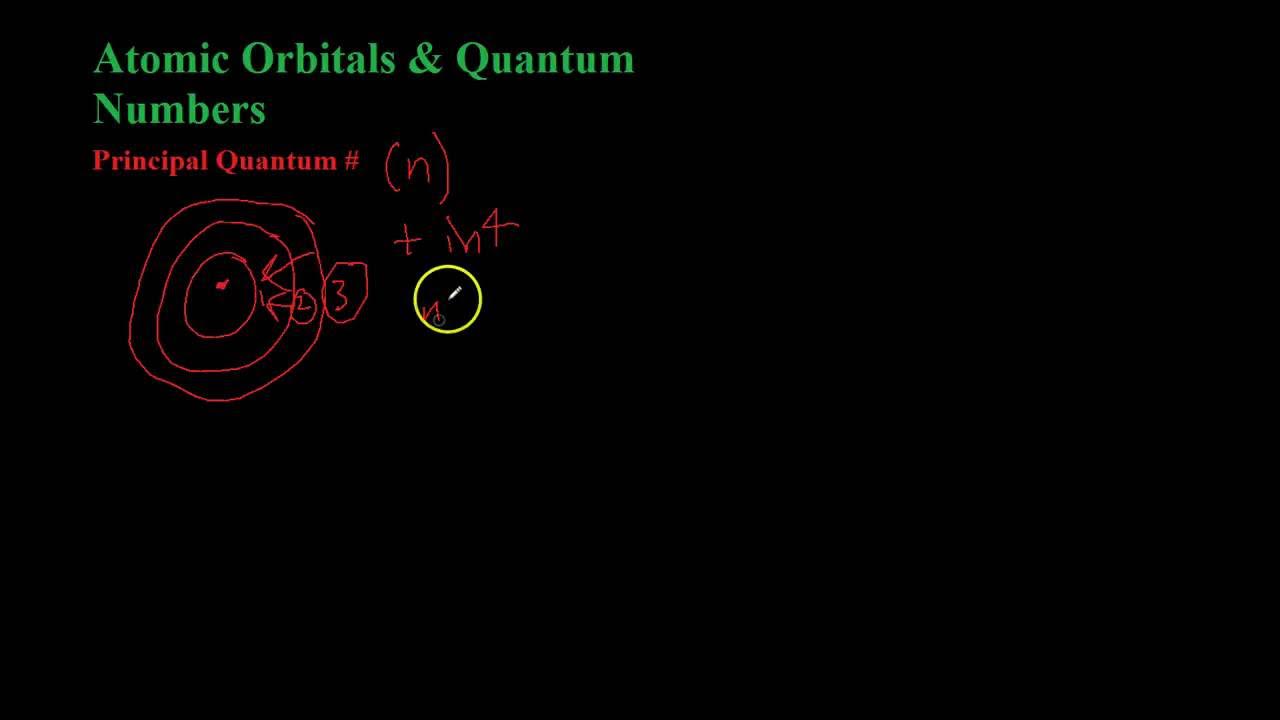
• 🏓 Particles are defined as existing in a single place at any instant, represented by a probability spike at one position.
• 🌀 Waves are spread out in space, with features like wavelength that can't be assigned a single position but have probabilities across many.
• 🔗 The wavelength of an object relates to its momentum, with faster or heavier objects having shorter wavelengths.
• 🏞 Everyday objects don't exhibit wave nature due to their minuscule wavelengths, but atomic or subatomic particles can.
• 🤔 To measure both position and momentum, a wave packet is created by combining waves of different wavelengths, introducing uncertainty.
• 🔄 The act of reducing position uncertainty by creating a smaller wave packet increases momentum uncertainty, and vice versa.
• 📖 Heisenberg's principle isn't about measurement limitations but reflects a fundamental property of the universe, inherent in its structure.

Q & A

What is the Heisenberg Uncertainty Principle?

The Heisenberg Uncertainty Principle states that it is impossible to simultaneously know the exact position and the exact speed (momentum) of an object, reflecting the inherent uncertainty in the properties of particles at the quantum level.

Why is the Heisenberg Uncertainty Principle significant in popular culture?

The Heisenberg Uncertainty Principle has become a metaphor for uncertainty in various fields, from literary criticism to sports commentary, because it captures the idea that complete certainty is unattainable, which resonates in many contexts.

How is the uncertainty principle related to the act of measurement?

The uncertainty principle is often misconceived as a result of measurement. However, the principle is more fundamentally about the nature of quantum objects behaving as both particles and waves, which inherently limits the precision with which their position and momentum can be known.

What does it mean for an object to behave like a particle?

An object behaving like a particle exists in a single place at any instant in time, which can be represented by a probability graph showing a spike at one specific position and zero elsewhere.

How is the concept of a wave different from that of a particle?

Waves are disturbances spread out in space, like ripples on a pond, and do not have a single position. They can be characterized by their wavelength, which is the distance between two neighboring peaks or valleys.

Why is wavelength important in quantum physics?

Wavelength is crucial in quantum physics because it is related to an object's momentum (mass times velocity). A fast-moving or heavy object has a short wavelength, which is significant for understanding the behavior of quantum objects.

Why don't we notice the wave nature of everyday objects?

The wave nature of everyday objects like a baseball is not noticeable because their wavelengths are extremely small, far beyond detection, due to their mass and speed.

How can small objects like atoms or electrons exhibit measurable wave properties?

Small objects such as atoms or electrons can have wavelengths that are large enough to be measured in physics experiments, making their wave nature observable.

How does combining waves with different wavelengths affect the position and momentum of a quantum object?

Combining waves with different wavelengths allows for the creation of a wave packet with a defined position but uncertain momentum, reflecting the trade-off between knowing an object's position and momentum due to the Heisenberg Uncertainty Principle.

What is the relationship between the size of a wave packet and the uncertainty of position and momentum?

A smaller wave packet, which would localize the position of a quantum object, inherently increases the uncertainty in its momentum, and vice versa. This relationship is a direct consequence of the Heisenberg Uncertainty Principle.

Who first stated the Heisenberg Uncertainty Principle, and when was it stated?

The Heisenberg Uncertainty Principle was first stated by German physicist Werner Heisenberg in 1927.

Is the uncertainty principle a practical limit on measurement or a fundamental property of the universe?

The uncertainty principle is not just a practical limit on measurement but a fundamental property of the universe, reflecting the inherent limitations in the properties that quantum objects can possess.

Outlines

00:00

🌌 The Heisenberg Uncertainty Principle Explained

The Heisenberg Uncertainty Principle is a quantum physics concept that has permeated popular culture, suggesting an inherent limit to our knowledge of an object's exact position and velocity simultaneously. This principle arises from the dual nature of quantum objects behaving as both particles and waves. Particles are defined by their precise location, represented by a spike in a probability graph, while waves are spread out, characterized by a wavelength. The principle is not due to measurement limitations but is fundamental to quantum mechanics. To reconcile the particle and wave nature, a wave packet is formed by combining waves of different wavelengths, which introduces uncertainty in both position and momentum. The more certain we want to be about an object's position, the less certain we can be about its momentum, and vice versa, encapsulating the essence of the Heisenberg Uncertainty Principle.

Keywords

💡Heisenberg Uncertainty Principle

The Heisenberg Uncertainty Principle is a fundamental concept in quantum mechanics that states it is impossible to simultaneously know both the exact position and exact speed (momentum) of an object. This principle is central to the video's theme, illustrating the inherent limits of our ability to measure and know the properties of quantum objects. The script explains that this principle is not just a measurement limitation but a fundamental aspect of the universe's structure.

💡Quantum Physics

Quantum physics is a branch of physics that deals with the behavior of particles at the atomic and subatomic level. The video uses quantum physics as the backdrop to discuss the Heisenberg Uncertainty Principle, emphasizing how it challenges classical physics' deterministic view of the universe. The script mentions that in quantum mechanics, the exact position and speed of an object have no meaning, highlighting the probabilistic nature of quantum states.

💡Wave-Particle Duality

Wave-particle duality is the concept that every quantum object can exhibit both wave-like and particle-like properties. This concept is crucial in the video as it explains why the Uncertainty Principle exists. The script describes how particles exist in a single place at a time, while waves are spread out, and how the combination of these properties leads to the uncertainty in position and momentum.

💡Momentum

Momentum, defined as mass times velocity, is a key property of objects in physics. In the context of the video, momentum is directly related to the wavelength of an object's wave function. The script uses momentum to explain how fast-moving or heavy objects have shorter wavelengths, which is why their wave nature is not noticeable in everyday life but becomes significant in quantum scales.

💡Wavelength

Wavelength is the distance between two consecutive peaks or troughs of a wave. It is essential in quantum physics as it is related to an object's momentum. The video explains that while a pure wave can have a well-defined wavelength, it does not have a specific position, illustrating the trade-off between knowing an object's position and its momentum.

💡Probability

In quantum mechanics, probability plays a significant role in determining where a particle might be found or what its momentum might be. The video script uses the concept of probability to explain how the Uncertainty Principle arises from the need to describe particles with both wave and particle characteristics, resulting in a range of possible positions and momenta.

💡Wave Packet

A wave packet is a localized region in space where the wave-like properties of a quantum object are concentrated. The video script describes how wave packets are created by combining waves of different wavelengths, which allows for the coexistence of particle and wave properties. However, this concentration of wave properties within a small region leads to uncertainty in both position and momentum.

💡Position

Position, in the context of the video, refers to the exact location of a quantum object. It is highlighted that in quantum mechanics, the exact position of a particle cannot be known with certainty if its momentum is also known. The script uses the concept of position to illustrate the limitations imposed by the Uncertainty Principle.

💡Measurement

Measurement, as discussed in the video, is not just an act of observation but a fundamental interaction that affects the properties of quantum objects. The script clarifies that the Uncertainty Principle is not a result of poor measurement techniques but an inherent feature of the quantum world, where the act of measurement influences the object's state.

💡Fundamental Structure of the Universe

The video script emphasizes that the Heisenberg Uncertainty Principle is not a mere practical limit but a reflection of the universe's fundamental structure. It suggests that the principle is a deep-seated aspect of reality, indicating that the universe operates on probabilities rather than certainties at the quantum level.

💡Wave Function

The wave function is a mathematical description of the quantum state of a system. In the video, the wave function is used to explain how particles can exhibit wave-like behavior. The script mentions how the wave function's properties, such as wavelength, are related to an object's momentum and how the wave function's shape influences the probabilities of position and momentum.

Highlights

The Heisenberg Uncertainty Principle is a fundamental concept in quantum physics that has permeated popular culture.

It asserts that one cannot know both the exact position and exact speed of an object simultaneously.

The principle is often misunderstood as a measurement issue, but it's a deeper quantum mechanical property.

Everything in the universe exhibits both particle and wave characteristics.

In quantum mechanics, the exact position and speed of an object are not well-defined.

Particles are defined as existing in a single place at any given time, represented by a probability spike.

Waves are disturbances spread out in space, without a single position, characterized by their wavelength.

Wavelength is crucial in quantum physics as it relates to an object's momentum, which is mass times velocity.

Fast-moving or heavy objects have short wavelengths, making their wave nature difficult to observe.

Small objects like atoms or electrons can have wavelengths significant enough to be measured in experiments.

A pure wave can have its wavelength measured, but it lacks a definite position.

A particle can have a known position, but without a wavelength, its momentum remains uncertain.

Combining waves with different wavelengths allows for the creation of a quantum object with both wave and particle properties.

Creating a wave packet with a clear wavelength in a small region requires a compromise on certainty for position and momentum.

The Heisenberg Uncertainty Principle dictates that reducing position uncertainty increases momentum uncertainty, and vice versa.

This principle was first stated by Werner Heisenberg in 1927 and is not just a measurement limitation but a fundamental property of the universe.

Transcripts

The Heisenberg Uncertainty Principle is one of a handful of ideas

from quantum physics to expand into general pop culture.

It says that you can never simultaneously know the exact position

and the exact speed of an object and shows up as a metaphor in everything

from literary criticism to sports commentary.

Uncertainty is often explained as a result of measurement,

that the act of measuring an object's position changes its speed, or vice versa.

The real origin is much deeper and more amazing.

The Uncertainty Principle exists because everything in the universe

behaves like both a particle and a wave at the same time.

In quantum mechanics, the exact position and exact speed of an object

have no meaning.

To understand this,

we need to think about what it means to behave like a particle or a wave.

Particles, by definition, exist in a single place at any instant in time.

We can represent this by a graph showing the probability of finding

the object at a particular place, which looks like a spike,

100% at one specific position, and zero everywhere else.

Waves, on the other hand, are disturbances spread out in space,

like ripples covering the surface of a pond.

We can clearly identify features of the wave pattern as a whole,

most importantly, its wavelength,

which is the distance between two neighboring peaks,

or two neighboring valleys.

But we can't assign it a single position.

It has a good probability of being in lots of different places.

Wavelength is essential for quantum physics

because an object's wavelength is related to its momentum,

mass times velocity.

A fast-moving object has lots of momentum,

which corresponds to a very short wavelength.

A heavy object has lots of momentum even if it's not moving very fast,

which again means a very short wavelength.

This is why we don't notice the wave nature of everyday objects.

If you toss a baseball up in the air,

its wavelength is a billionth of a trillionth of a trillionth of a meter,

far too tiny to ever detect.

Small things, like atoms or electrons though,

can have wavelengths big enough to measure in physics experiments.

So, if we have a pure wave, we can measure its wavelength,

and thus its momentum, but it has no position.

We can know a particles position very well,

but it doesn't have a wavelength, so we don't know its momentum.

To get a particle with both position and momentum,

we need to mix the two pictures

to make a graph that has waves, but only in a small area.

How can we do this?

By combining waves with different wavelengths,

which means giving our quantum object some possibility of having different momenta.

When we add two waves, we find that there are places

where the peaks line up, making a bigger wave,

and other places where the peaks of one fill in the valleys of the other.

The result has regions where we see waves

separated by regions of nothing at all.

If we add a third wave,

the regions where the waves cancel out get bigger,

a fourth and they get bigger still, with the wavier regions becoming narrower.

If we keep adding waves, we can make a wave packet

with a clear wavelength in one small region.

That's a quantum object with both wave and particle nature,

but to accomplish this, we had to lose certainty

about both position and momentum.

The positions isn't restricted to a single point.

There's a good probability of finding it within some range

of the center of the wave packet,

and we made the wave packet by adding lots of waves,

which means there's some probability of finding it

with the momentum corresponding to any one of those.

Both position and momentum are now uncertain,

and the uncertainties are connected.

If you want to reduce the position uncertainty

by making a smaller wave packet, you need to add more waves,

which means a bigger momentum uncertainty.

If you want to know the momentum better, you need a bigger wave packet,

which means a bigger position uncertainty.

That's the Heisenberg Uncertainty Principle,

first stated by German physicist Werner Heisenberg back in 1927.

This uncertainty isn't a matter of measuring well or badly,

but an inevitable result of combining particle and wave nature.

The Uncertainty Principle isn't just a practical limit on measurment.

It's a limit on what properties an object can have,

built into the fundamental structure of the universe itself.