Number System grade 10

Summary

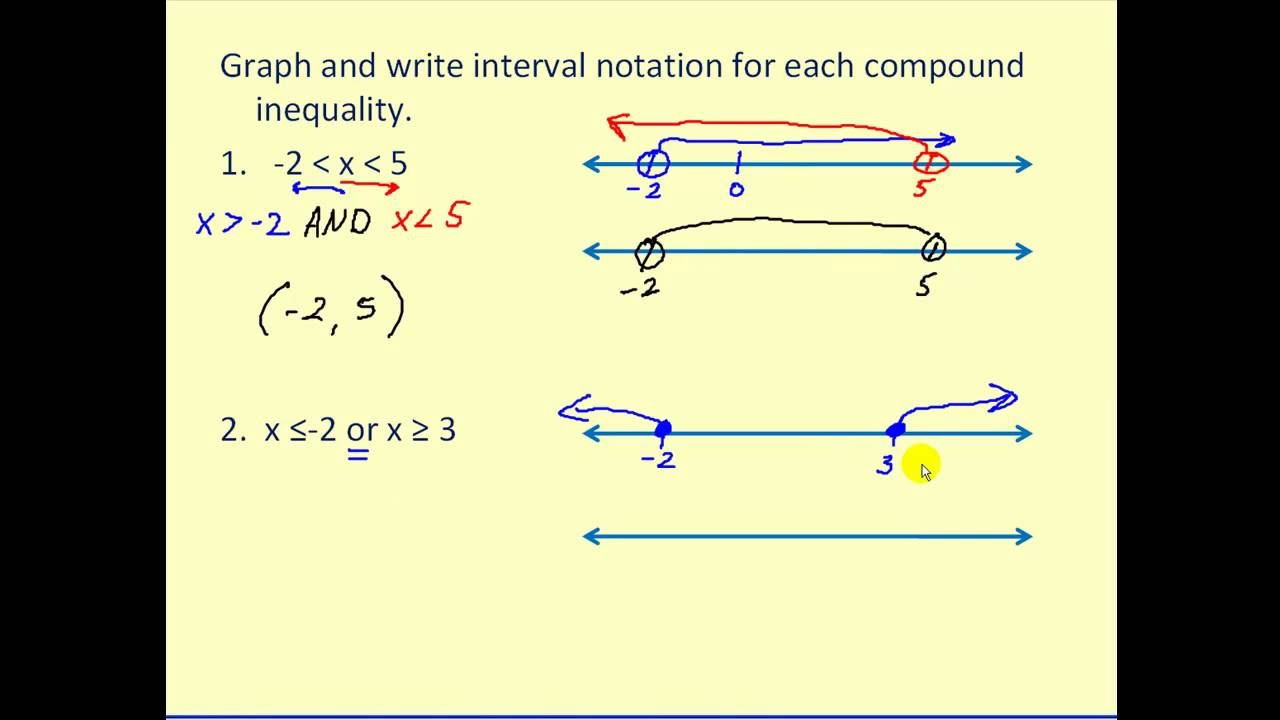
This educational video lesson focuses on teaching interval notation, set builder notation, and number lines, which are often challenging for students. The instructor uses a step-by-step approach to explain how to represent numbers greater than three and less than seven on a number line, employing open circles to denote exclusion of the endpoints. The lesson covers interval notation with parentheses to indicate non-inclusive boundaries and set builder notation using brackets to specify conditions for variables. The instructor also introduces the concept of real numbers and infinity, using creative analogies like a hungry crocodile to help students remember the direction of inequalities. The lesson aims to demystify these mathematical concepts through practice and repetition.

Takeaways

• 📚 The lesson introduces interval notation, set builder notation, and number lines, which are often challenging for students.
• 🔢 It explains how to represent numbers greater than three and less than seven, including examples like 3.1, 4.2, 5.6, and 6.54.
• 📏 The number line is used to visualize intervals, with open circles indicating numbers not included (e.g., 3 and 7).
• 📝 Interval notation is introduced as a concise way to express ranges, using round brackets to denote non-inclusive endpoints.
• 🦎 A mnemonic is used to remember the notation: a 'hungry crocodile' facing the larger number signifies 'greater than' or 'less than'.
• 📋 Set builder notation is explained, using specific brackets and the letter 'x' to define the set of numbers that meet certain conditions.
• 🌐 The concept of real numbers is briefly touched upon, indicating that 'x e r' signifies 'x is an element of the real numbers'.
• 🔄 The lesson reiterates the importance of practice to understand and apply these mathematical notations effectively.
• 🔄 The instructor provides another example with numbers greater than or equal to 7 and less than 12, demonstrating how to adjust the notation accordingly.
• ♾️ The lesson concludes with an example of numbers greater than five, introducing the concept of infinity in interval notation.

Q & A

What are the three different ways to represent numbers between 3 and 7 in mathematics?

The three different ways to represent numbers between 3 and 7 are using a number line with open circles at 3 and 7, interval notation (x e [3,7)), and set-builder notation (x ∈ ℝ | 3 < x < 7).

What does the open circle on a number line represent?

An open circle on a number line indicates that the number at that point is not included in the set.

How do you represent numbers that are not included in the set using interval notation?

In interval notation, numbers that are not included in the set are represented with round brackets, for example, (3,7).

What does 'x e' stand for in interval notation?

In interval notation, 'x e' is an abbreviation for 'x is an element of', indicating that x belongs to the set described within the brackets.

What is set-builder notation and how is it used to describe numbers between 3 and 7?

Set-builder notation is a way to describe a set of numbers using a variable, a condition, and a colon. For numbers between 3 and 7, it would be written as {x | 3 < x < 7}.

What does 'x ∈ ℝ' mean in set-builder notation?

'x ∈ ℝ' in set-builder notation means that x is an element of the set of real numbers, indicating that x can be any real number that satisfies the given condition.

How do you represent a number that is bigger than 5 but not including 5 on a number line?

On a number line, a number that is bigger than 5 but not including 5 would be represented with an open circle at 5 and a continuous arrow extending to the right.

What is the interval notation for numbers that are bigger than 5 but not including 5?

The interval notation for numbers that are bigger than 5 but not including 5 is (5, ∞).

How do you represent numbers that are bigger than 5 using set-builder notation?

In set-builder notation, numbers that are bigger than 5 would be represented as {x ∈ ℝ | x > 5}.

Why is it important to practice these different notations for representing numbers?

Practicing different notations helps to solidify understanding of how to represent sets of numbers mathematically and can be useful in various mathematical contexts and problem-solving.

What is the significance of the direction of the inequality symbols in set-builder notation?

The direction of the inequality symbols in set-builder notation indicates whether the boundary numbers are included or excluded. For example, '>' means the number is strictly greater, excluding the boundary, while '≥' would include the boundary number.