Addition of Vectors By Means of Components - Physics

Summary

This video script offers an insightful tutorial on vector addition, emphasizing the importance of both magnitude and direction. It explains the straightforward process of adding parallel vectors and the more complex task of combining vectors at various angles, including perpendicular ones. The script delves into calculating the resultant force vector's magnitude and direction through component analysis and trigonometric methods, providing clear examples and formulas to guide viewers through the process.

Takeaways

• 📚 A vector is a quantity with both magnitude and direction, such as a force vector with a specific strength and direction.
• 🔍 When adding parallel vectors, simply sum their magnitudes to get the resultant force.
• 🤔 For anti-parallel vectors, subtract the smaller magnitude from the larger to find the net force, with the direction of the larger vector.
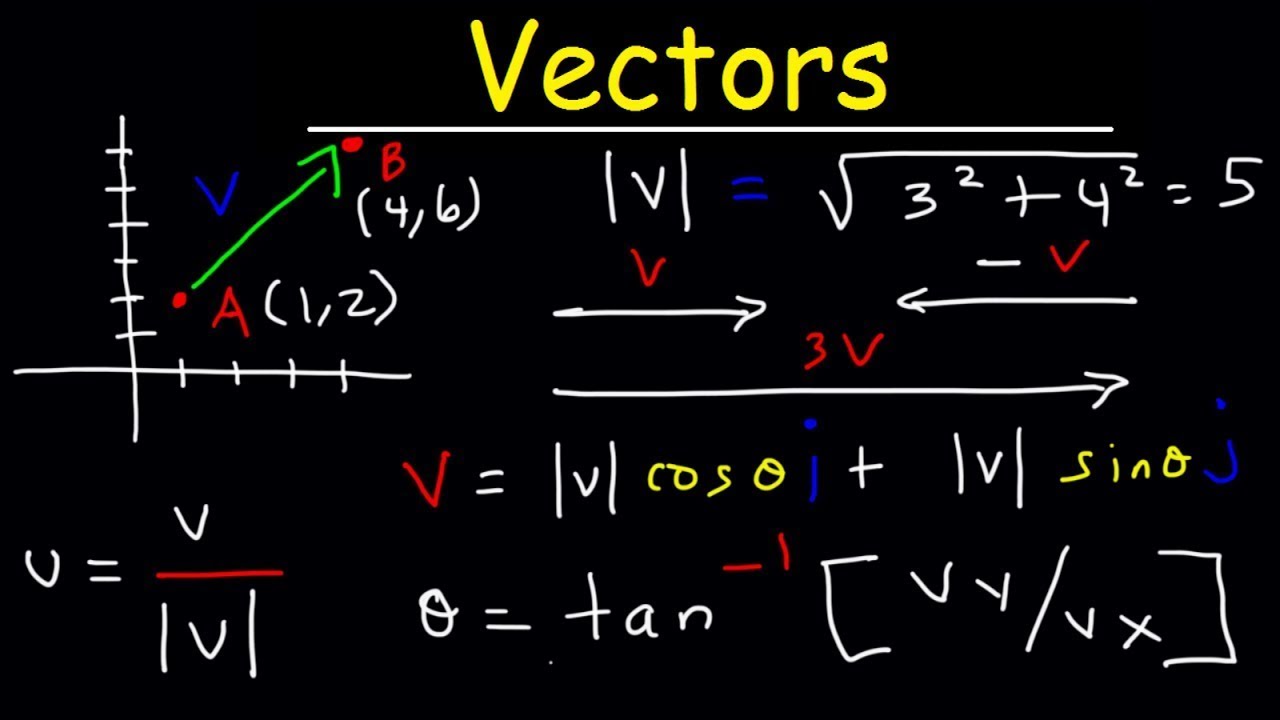
• 📐 To find the resultant of perpendicular vectors, calculate the square root of the sum of the squares of their magnitudes.
• 🧭 The direction of the resultant vector can be found using the inverse tangent function (arctan) of the y-component divided by the x-component.
• 📈 The magnitude of the resultant vector from non-parallel or non-perpendicular vectors is the square root of the sum of the squares of the individual x and y components.
• 📉 To determine the direction of the resultant vector, use the arctan of the y-component over the x-component, adjusting for the correct quadrant.
• 📝 The reference angle can be found by taking the arctan of the y-component divided by the x-component, always resulting in an acute angle.
• 📈 The component method involves breaking down vectors into their x and y components, summing these components, and then finding the resultant vector.
• 📐 The Pythagorean theorem is used to relate the magnitude of a vector to its x and y components.
• 🔢 The angle theta of a vector can be determined using trigonometric relationships, such as arctan for the direction.

Q & A

What is a vector and what are its two main components?

A vector is a quantity that has both magnitude and direction. The magnitude is the size of the vector, such as the strength of a force, while the direction indicates the orientation, like east or north.

How do you add two vectors that are parallel to each other?

When adding two parallel vectors, you simply add their magnitudes together. For example, if you have a 100 Newton force vector directed east and another 50 Newton force vector in the same direction, the resultant force is 150 Newtons.

What is the resultant vector when adding two anti-parallel vectors?

For anti-parallel vectors, you subtract their magnitudes. For instance, a 200 Newton vector directed east and a 120 Newton vector directed west would result in a net force of 80 Newtons directed east.

How do you determine the magnitude and direction of the resultant vector when adding two perpendicular vectors?

For perpendicular vectors, you find the magnitude of the resultant vector by calculating the square root of the sum of the squares of their magnitudes. The direction can be found using the inverse tangent of the y-component divided by the x-component.

What is the resultant force vector and its direction when adding a 60 Newton east vector and a 90 Newton west vector?

The resultant force vector would be 30 Newtons directed west, as the east vector is 60 Newtons and the west vector is 90 Newtons, resulting in a net force of -30 Newtons, which can be stated as 30 Newtons west.

How do you calculate the magnitude of the resultant vector when adding a 50 Newton west vector and a 120 Newton south vector?

You would calculate the magnitude by taking the square root of the sum of the squares of the two vectors, which in this case is the square root of 50 squared plus 120 squared, resulting in a magnitude of 130 Newtons.

What is the reference angle and how is it used to determine the direction of a resultant vector?

The reference angle is the acute angle formed between the resultant vector and the x-axis, found using the arctangent of the y-component divided by the x-component. It is used to adjust the direction of the resultant vector based on the quadrant it lies in.

How do you find the direction of the resultant vector when adding a 50 Newton west vector and a 120 Newton south vector?

First, find the reference angle using the arctangent of the y-component (120) divided by the x-component (50), which gives an angle of 67.4 degrees. Since this is in quadrant three, the direction of the resultant vector is 180 degrees plus the reference angle, resulting in 247.4 degrees from the positive x-axis.

What is the magnitude and direction of the resultant force vector when adding a 45 Newton east vector and a 60 Newton south vector?

The magnitude is found by taking the square root of the sum of the squares of the two vectors, which is 75 Newtons. The direction is found by calculating the angle in the fourth quadrant, which is 360 degrees minus the reference angle of 53.1 degrees, resulting in 306.9 degrees from the positive x-axis.

How do you add vectors that are not parallel or perpendicular using the component method?

You break down each vector into its x and y components based on its magnitude and angle. Then, you add the x-components together and the y-components together. Finally, you find the magnitude of the resultant vector using the Pythagorean theorem and the direction using the inverse tangent of the y-component divided by the x-component.

Can you provide an example of adding a 100 Newton east vector and a 150 Newton vector at 30 degrees above the x-axis using the component method?

First, find the x and y components of each vector. For the 100 Newton east vector, the x-component is 100 and the y-component is 0. For the 150 Newton vector at 30 degrees, the x-component is 150 times cosine(30) and the y-component is 150 times sine(30). Add the x-components to get 229.9 and the y-components to get 75. Then, find the magnitude of the resultant vector, which is the square root of 229.9 squared plus 75 squared, resulting in 241.8 Newtons. The direction is found using the inverse tangent of the y-component divided by the x-component, giving an angle of 18.1 degrees.