GENERATING PATTERNS IN SEQUENCES II GRADE 10 MATHEMATICS Q1
Summary
TLDRIn this educational video, the concept of sequences is explored, distinguishing between finite and infinite sequences. The presenter illustrates how to identify patterns and derive mathematical expressions for generating sequences, using examples such as alphabetic sequences, odd numbers, perfect squares, and arithmetic progressions. The video also explains the terminology of sequences, including terms like 'general term' and 'nth term,' and demonstrates how to apply these concepts to find specific terms within a sequence.
Takeaways
- 😀 The video defines sequences as ordered lists of numbers, distinguishing between finite and infinite sequences based on their domain.
- 🔍 It introduces the concept of terms within a sequence, explaining that each number in a sequence is called a term and how to denote them using subscript notation.
- 📝 The script walks through the process of identifying patterns in sequences to derive a mathematical rule for generating terms, using examples with alphabet letters and numbers.
- 📈 It demonstrates how to find specific terms in a sequence, such as the 19th term, by applying the general term formula.
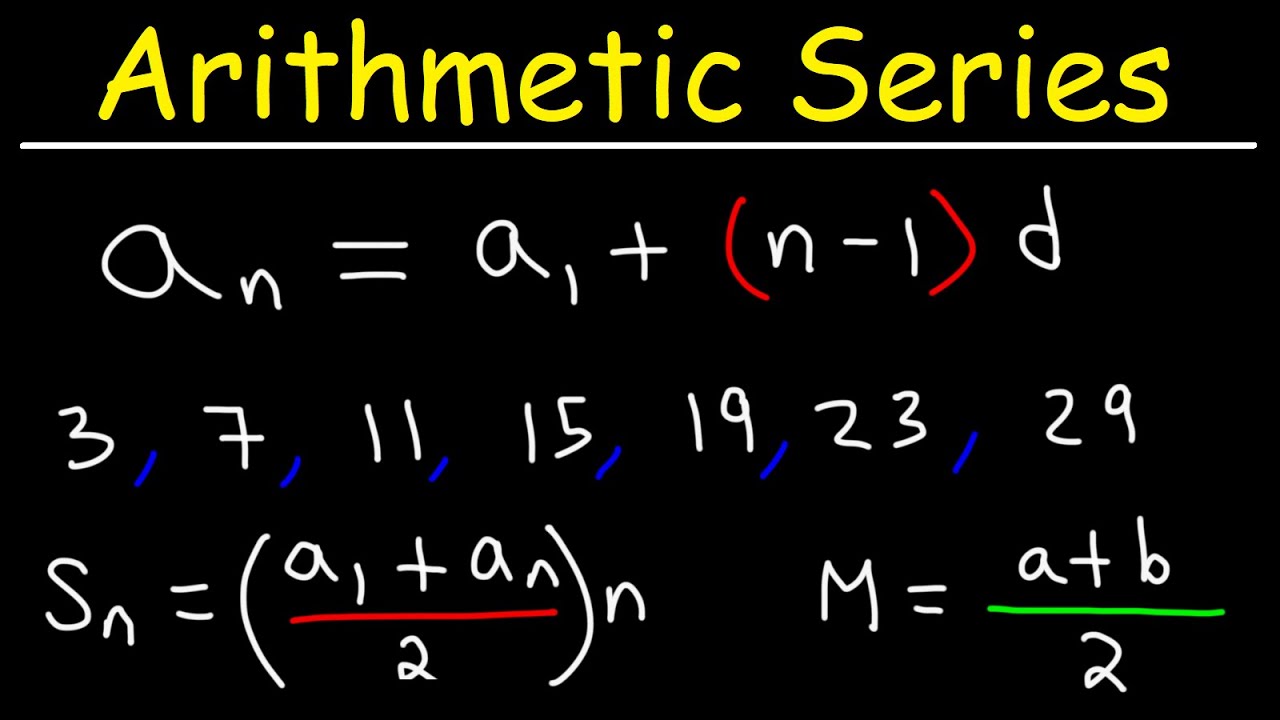
- 🔢 The video uses examples of sequences where the pattern involves adding a constant number to each term (e.g., adding 10).
- 🎓 It explains the difference between the first term and the general term of a sequence, with the first term being a specific value and the general term being a formula that can generate any term in the sequence.
- 📚 The script provides a method to find the first few terms of a sequence when given its general term formula, by substituting the first few natural numbers into the formula.
- 🤔 It challenges viewers to think about the general term of a sequence by examining given terms and deducing the underlying mathematical rule.
- 📉 The video also covers sequences where the terms alternate in sign, such as those involving (-1)^n, and explains how this affects the sequence's pattern.
- 📊 Examples of sequences with specific patterns, like perfect squares or cubes, are used to illustrate how to derive the general term formula.
- 👍 The video concludes with an encouragement to like, subscribe, and hit the bell button for more educational content.
Q & A
What is a sequence in mathematics?
-A sequence in mathematics is a function whose domain is the set of positive integers, representing an ordered list of numbers.
What is the difference between a finite and an infinite sequence?
-A finite sequence has a last term and its domain is a set of positive integers up to a certain number, often denoted by 'n'. An infinite sequence, on the other hand, does not have a last term and its domain is the entire set of positive integers.
How are the terms in a sequence referred to?
-Each number in a sequence is called a term. For example, the first term is often denoted as 'a_1', the second term as 'a_2', and so on.
What is the general term of a sequence?
-The general term of a sequence is a rule or formula that generates each term in the sequence. It is often denoted as 'a_n', where 'n' is the position of the term in the sequence.
How can you find the next term in a sequence given a pattern?
-To find the next term in a sequence, you can look for a pattern in the given terms, such as adding a constant number, multiplying by a constant, or following a mathematical operation that applies to each term.
What is the pattern in the sequence 1, 3, 5, 7, ...?
-The pattern in this sequence is that each term is an odd number, and the sequence increases by 2 each time. So, the next term after 7 would be 9, followed by 11, and so on.
How do you determine the next term in the sequence 1, 4, 9, 16, 25, ...?
-This sequence consists of perfect squares. Each term is the square of its position in the sequence (1^2, 2^2, 3^2, etc.), so the next term after 25 (which is 5^2) would be 6^2, which is 36.
What is the pattern in the sequence 5, 15, 25, 35, 45, ...?
-The pattern in this sequence is that each term increases by 10 from the previous term. So, starting from 5, you add 10 to get the next term.
How can you find the nth term of a sequence given its general term formula?
-To find the nth term of a sequence, you substitute the value of 'n' into the general term formula and calculate the result.
What is the general term for a sequence that alternates between positive and negative terms?
-A sequence that alternates between positive and negative terms often has a general term involving (-1)^n, which raises -1 to the power of the term's position, resulting in alternating signs.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
5.0 / 5 (0 votes)