KESEBANGUNAN BANGUN DATAR matematika SMP kelas VII Kurikulum Merdeka revisi 2022 Bab 5
Summary
TLDRIn this video, the concept of similarity in geometry is explained for 7th-grade students, focusing on both quadrilaterals (parallelograms) and triangles. The conditions for similarity are outlined: corresponding sides must be proportional, and corresponding angles must be equal. The video provides examples of parallelograms and right-angled triangles to illustrate these principles. A step-by-step problem-solving example is included to demonstrate how to find missing side lengths using the similarity condition. This comprehensive explanation makes it easier for students to grasp the concepts and apply them in solving geometry problems.
Takeaways
- 😀 Similarity in geometry refers to two figures having the same shape but different sizes, with corresponding sides in proportion and corresponding angles equal.
- 😀 The first condition for two figures to be similar is that corresponding sides must have equal ratios (proportions).
- 😀 The second condition for similarity is that corresponding angles must be congruent (equal).
- 😀 In parallelograms, the corresponding sides must have proportional lengths, and the angles between corresponding sides must be the same.
- 😀 For example, when comparing two parallelograms, their corresponding sides can be compared for proportionality to determine if they are similar.
- 😀 The same principles of similarity apply to triangles, where corresponding sides must have the same ratio and corresponding angles must be equal.
- 😀 An example of a triangle comparison involves calculating side ratios, such as comparing sides of 6 cm and 9 cm, and 8 cm and 12 cm, and ensuring corresponding angles match.
- 😀 In solving problems involving similar figures, proportionality of sides and equality of angles are used to find missing measurements.
- 😀 In a specific example, two similar triangles can be used to find an unknown side by setting up a proportion equation between corresponding sides.
- 😀 Understanding similarity in geometric figures helps in solving real-world problems, such as finding missing side lengths or determining whether two shapes are similar.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the concept of similarity in geometry, specifically focusing on quadrilaterals and triangles, as well as the conditions for two shapes to be considered similar.
What are the two key conditions for two shapes to be considered similar?
-The two key conditions are: 1) The corresponding sides of the shapes must be in proportion (have equal ratios), and 2) The corresponding angles must be equal.
How is the proportionality of sides verified in the script using parallelograms?
-The proportionality of sides is verified by comparing the corresponding sides of two parallelograms. For example, the side AB of the first parallelogram is compared to side EF of the second parallelogram, and the ratio of their lengths is found to be 1:2, confirming the proportionality.
How are the corresponding angles of similar shapes verified in the video script?
-The corresponding angles are verified by checking that the angles of one shape match the angles of the other. For example, angle A in the first parallelogram is equal to angle E in the second parallelogram, and this pattern follows for all corresponding angles.
What is the importance of the angle sum of 180 degrees in the script?
-The angle sum of 180 degrees is important because it helps to verify that the corresponding angles in two similar shapes are indeed equal. For example, angle A and angle B in a parallelogram add up to 180 degrees, and the same is true for their corresponding angles in the second parallelogram.
How does the script demonstrate the similarity condition in right triangles?
-In the script, the similarity of two right triangles is demonstrated by comparing the corresponding sides. For instance, the side AB of the first triangle is compared to side DE of the second triangle, and the ratios of the sides are found to be equal, confirming the similarity.
How does the script use an equation to solve for an unknown side in a similar triangle?
-The script uses an equation to solve for the unknown side by setting up a proportion based on the similarity of the triangles. For example, the equation EB/AB = EF/AC is set up, and by cross-multiplying, the unknown side (EB) is calculated.
What method is used to simplify the ratios of the corresponding sides?
-The method used to simplify the ratios involves dividing both sides of the ratio by their greatest common divisor (GCD). This simplification ensures that the ratios are in their simplest form, such as simplifying 6:12 to 1:2.
What role does the variable 'x' play in solving the example problem with triangles?
-The variable 'x' represents the unknown length of side EB in the example problem. By setting up the proportion and solving for 'x', the value of the unknown side is determined.
How is the solution for 'x' derived in the triangle example?
-The solution for 'x' is derived by cross-multiplying the proportion (EF/AC = EB/AB) and simplifying the resulting equation. After solving, the value of 'x' is found to be 6 cm.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

Kurikulum Merdeka Materi Matematika Kelas 7 Bab 5 Kesebangunan

Matematika Kelas 5 SD - Bab 4 Keliling Bangun Datar || Kurikulum Merdeka
Quadrilaterals - Trapezoids, Parallelograms, Rectangles, Squares, and Rhombuses!

Jenis -Jenis dan Sifat - Sifat Segi Empat | Matematika SMP/MTs Kelas 7
Properties of Parallelogram - @MathTeacherGon
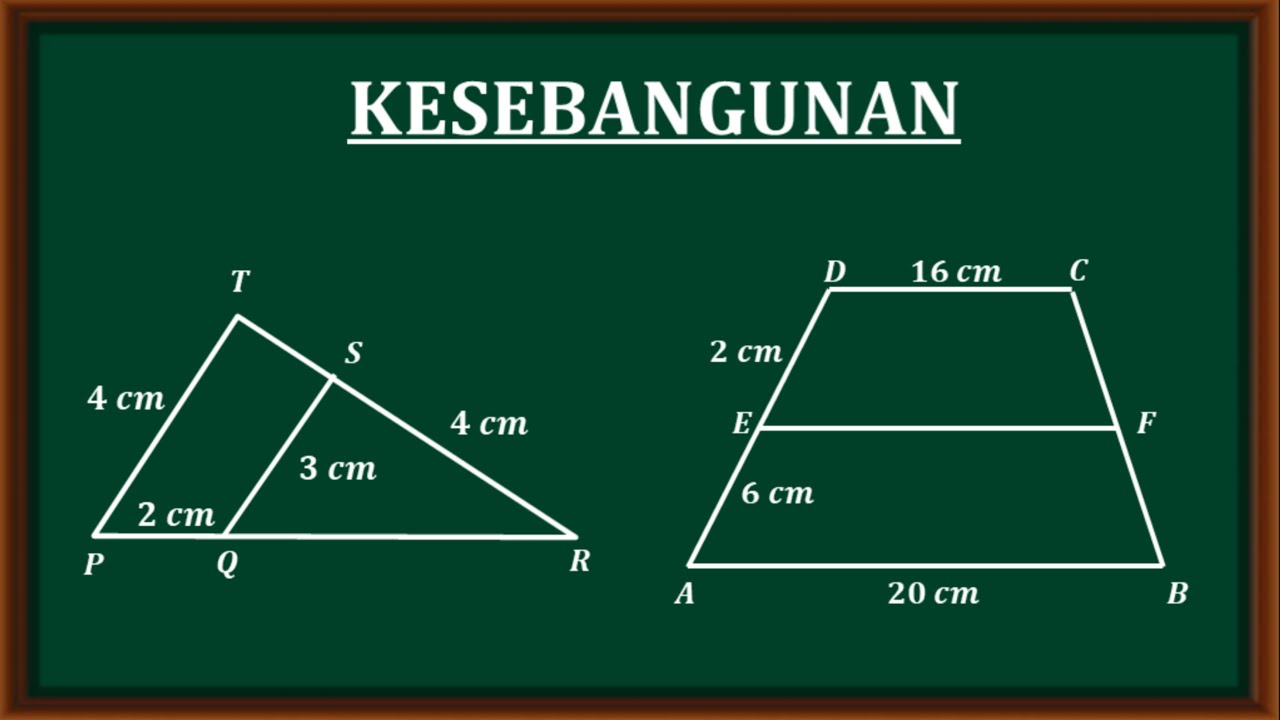
Kesebangunan - soal dan pembahasan materi kesebangunan matematika tingkat SMP kelas ix
5.0 / 5 (0 votes)
