Congruent Figures part 2

Summary

This video lesson focuses on the concept of congruent figures, specifically exploring the CPCFC theorem (Corresponding Parts of Congruent Figures are Congruent). The teacher explains how corresponding sides and angles of congruent figures are congruent and emphasizes the importance of congruence statements. A key point is the correct order in which the vertices of the figures must be listed. The teacher also discusses different ways to write congruence statements and clarifies the misconception that there's only one way to do so. This content is essential for understanding future proofs in geometry.

Takeaways

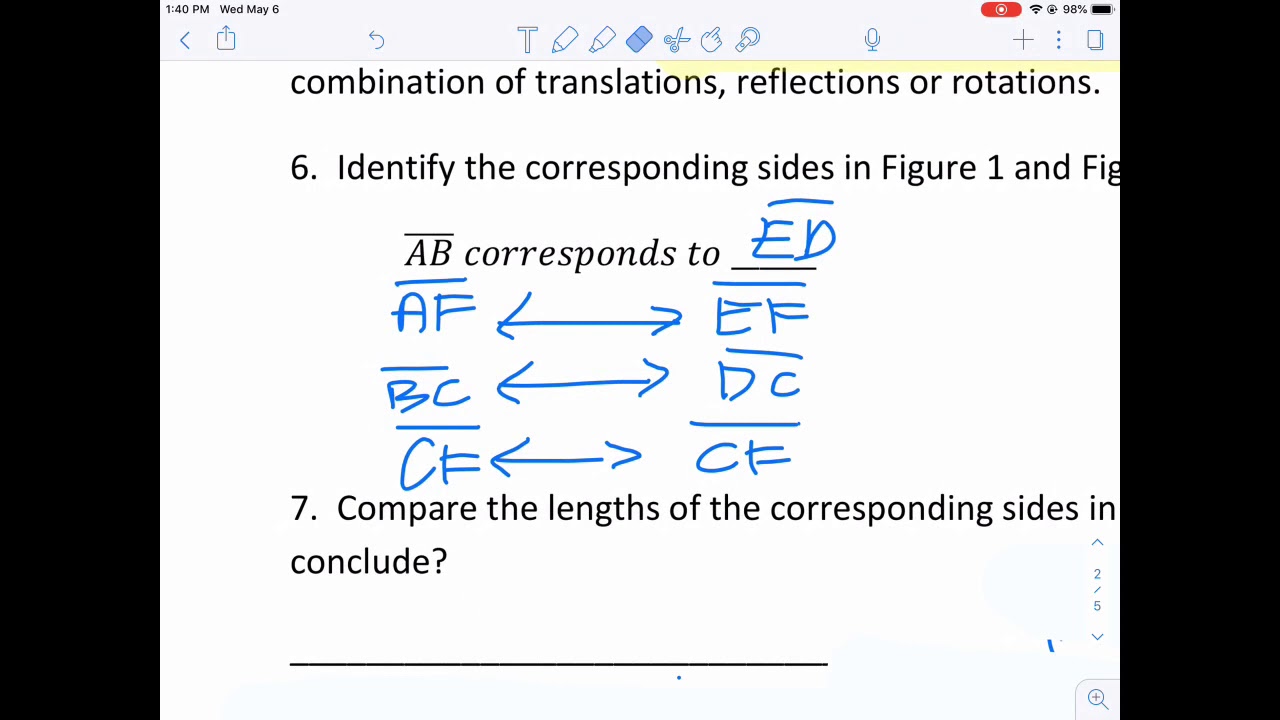
• 😀 Corresponding sides of congruent figures are congruent.
• 😀 Corresponding angles of congruent figures are congruent.
• 😀 Corresponding sets of parallel sides in congruent figures are also congruent.
• 😀 The CPC FC theorem stands for 'Corresponding Parts of Congruent Figures are Congruent'.
• 😀 The CPC FC theorem is an important concept for future geometric proofs.
• 😀 A congruence statement must list corresponding vertices in the same order.
• 😀 The order of vertices in a congruence statement must match the order in which the figures are presented.
• 😀 The only correct congruence statement maintains a consistent clockwise or counterclockwise order of vertices.
• 😀 Multiple ways can be used to write congruence statements for figures if the order of vertices is maintained.
• 😀 The leading vertex in a congruence statement can be chosen freely, allowing for different ways to write it.
• 😀 The understanding of congruence statements and the CPC FC theorem is foundational for solving future geometric proofs.

Q & A

What does CPC FC stand for in the context of this lesson?

CPC FC stands for 'Corresponding Parts of Congruent Figures are Congruent'. This theorem asserts that corresponding sides and angles of congruent figures are congruent.

What are the key points of the CPC FC theorem that should be noted?

The key points of the CPC FC theorem are that corresponding sides are congruent, corresponding angles are congruent, and corresponding sets of parallel sides are congruent.

Why is the congruence of corresponding sides and angles important in geometry?

The congruence of corresponding sides and angles is crucial because it allows for the establishment of relationships between figures, enabling us to prove other geometric properties and solve problems.

What does it mean for sides or angles to be congruent?

For sides or angles to be congruent, they must be equal in measurement. This means that corresponding sides or angles in congruent figures have the same length or angle size.

What was the mistake in the incorrect congruence statement shown in the script?

The mistake in the incorrect congruence statement was the order of the vertices. The vertices in the congruence statement must be written in corresponding order, and one statement had the vertices written in counterclockwise order, which was not correct.

What makes a congruence statement valid?

A congruence statement is valid if the corresponding vertices of two figures are written in the same order. The vertices must correspond in a consistent and logical manner.

How can congruence statements be written for a figure?

Congruence statements can be written in multiple ways as long as the corresponding vertices are listed in the same order. The leading vertex can be any point, and the statement can start with different vertices as long as the sequence remains consistent.

Why is it important to write congruence statements in a specific order?

It is important to write congruence statements in a specific order to ensure that the corresponding sides and angles are matched correctly between two figures. This order is crucial for maintaining the integrity of geometric proofs.

What was the debate between Sally and Johnny about congruence statements?

Sally believed that there were many ways to write congruence statements, as long as the corresponding vertices were in order. Johnny disagreed, thinking there was only one way to write them. Sally was correct, as there are multiple valid ways to start a congruence statement, depending on the leading vertex.

What is the role of congruence statements in geometric proofs?

Congruence statements play a crucial role in geometric proofs as they help establish the relationships between different parts of geometric figures. They are used to show that specific sides or angles of figures are equal, which is essential for proving further geometric properties and theorems.