Kalkulus | Barisan dan Deret Tak Hingga (Part 14) - Uji Deret Positif (Uji Integral)

Summary

This video explains the integral test for positive series. It outlines the necessary conditions for applying this test: the function must be continuous, monotonically decreasing, and positive on the interval from 1 to infinity. The process involves evaluating an improper integral to determine if the series converges or diverges. If the integral converges, the series converges; if the integral diverges, the series also diverges. The relationship between the area under the curve and the behavior of the series is highlighted, emphasizing how the integral’s area approaching a finite value signals convergence, while growing unbounded indicates divergence.

Takeaways

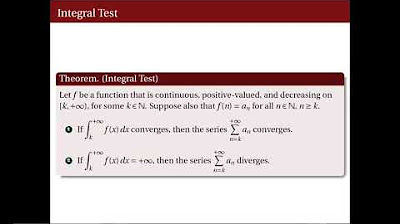
• 😀 The first positive series test discussed is the integral test.
• 😀 The integral test involves a function 'f' that must be continuous, monotonically decreasing, and positive over the interval from 1 to infinity.
• 😀 If the improper integral from 1 to infinity of f(x)g(x) converges, the series also converges.
• 😀 If the improper integral from 1 to infinity of f(x)g(x) diverges, the series diverges as well.
• 😀 The integral test has three key conditions: continuity, monotonicity (non-increasing), and positivity over the interval [1, ∞).
• 😀 The test applies to series where the function must be continuous, monotonically decreasing, and positive over the interval from 1 to infinity.
• 😀 To apply the integral test, one calculates the improper integral of the function from 1 to infinity.
• 😀 If the result of the improper integral is finite (convergent), then the series is also convergent.
• 😀 If the improper integral diverges (goes to infinity), the series is divergent.
• 😀 The relationship between the improper integral and the series can be understood through the concept of area—if the area under the curve keeps increasing without bound, the integral and series diverge, but if it approaches a fixed value, both converge.

Q & A

What is the main purpose of the Integral Test in series convergence?

The main purpose of the Integral Test is to determine whether a series converges or diverges by evaluating the corresponding improper integral. If the integral converges, the series converges; if the integral diverges, the series also diverges.

What are the three conditions required to apply the Integral Test?

The three conditions are: (1) the function must be continuous on the interval [1, ∞), (2) the function must be monotonically decreasing, and (3) the function must be positive on the interval [1, ∞).

Why does the function need to be continuous for the Integral Test?

The function needs to be continuous to ensure that the improper integral can be properly evaluated without any discontinuities that could interfere with determining the convergence or divergence of the series.

What does it mean for a function to be monotonically decreasing in the context of the Integral Test?

A function is monotonically decreasing if, as x increases, the function either decreases or stays constant. This ensures that the area under the curve behaves predictably, which is necessary for the test to be valid.

What does it mean for a function to be positive in the Integral Test?

The function must be positive on the interval [1, ∞) so that the area under the curve is meaningful and non-negative. This ensures that the series' terms are contributing positively to the sum.

What happens if the improper integral is convergent?

If the improper integral is convergent, it means the series corresponding to the function also converges.

What does it mean if the improper integral is divergent?

If the improper integral is divergent, it means the area under the curve increases without bound, leading to a conclusion that the series corresponding to the function also diverges.

How does the area under the curve relate to the convergence of the series?

The area under the curve represents the sum of the terms of the series. If the area keeps growing as x increases, the series diverges. If the area approaches a finite value, the series converges.

What is the role of the improper integral in determining series convergence?

The improper integral helps evaluate whether the series behaves similarly to the integral. By checking the convergence of the integral, we can conclude whether the series itself converges or diverges.

Can the Integral Test be applied to any series?

No, the Integral Test can only be applied to series where the terms of the series correspond to a function that meets the required conditions: continuity, monotonicity, and positivity on the interval [1, ∞).