Lecture 32 : Improper Integral – Part 2
Summary
TLDRThe video explores the comparison test in calculus, a method to determine the convergence or divergence of integrals. It explains how to apply the test by comparing a given function with a larger or smaller function whose integral behavior is known. The video covers different scenarios: when the larger integral converges, the smaller one does too, and vice versa for divergence. It also discusses the limit comparison test, showing how the relationship between two functions affects their integrals' behavior. Practical examples demonstrate the application of these concepts, offering a clear understanding of integral convergence.
Takeaways
- 😀 Comparison Test 1 helps in determining convergence by comparing an integrand to a known simpler function that is either larger or smaller.
- 😀 If the larger function’s integral converges, then the integral of the smaller function also converges (if both are non-negative).
- 😀 If the integral of the smaller function diverges, then the integral of the larger function will also diverge.
- 😀 The first comparison test provides an easy way to prove convergence or divergence by relating the given integral to known results.
- 😀 Comparison Test 2 applies when two functions are related by an inequality, and their ratio approaches a constant as x goes to infinity.
- 😀 If the ratio of the functions’ behavior approaches a constant that is not zero, then both integrals will behave similarly (either both converge or both diverge).
- 😀 If the ratio of the functions tends to zero and the larger function converges, then the smaller function will also converge.
- 😀 If the ratio of the functions tends to infinity and the larger function diverges, then the smaller function will also diverge.
- 😀 The goal of these tests is to simplify the process of evaluating improper integrals by comparing them to simpler functions.
- 😀 The comparison tests are especially useful when direct evaluation of an integral is difficult or impossible.
- 😀 These tests help to draw conclusions about integrals without fully solving them, based on the known behavior of related functions.
Please replace the link and try again.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

KALKULUS INTEGRAL - INTEGRAL TAK WAJAR
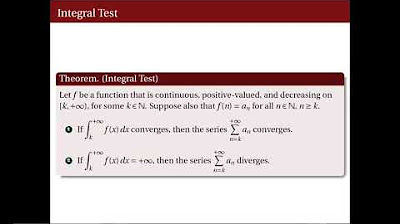
[Math 22] Lec 08 Integral Test and Comparison Test (Part 1 of 2)

3. Числовой ряд. Признак сравнения рядов. Предельный признак сравнения рядов.

Convergence and Divergence: The Return of Sequences and Series
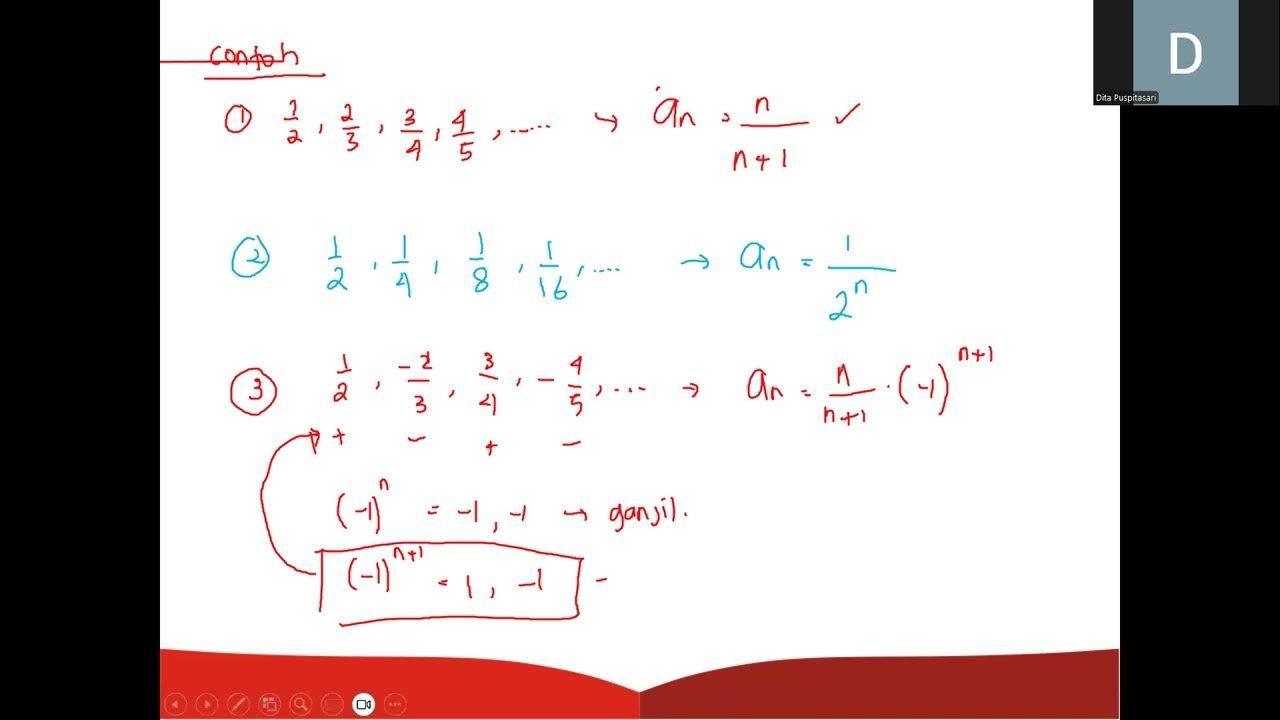
Kalkulus 1 - Barisan dan Deret (Part 1)

Sequences and Its Examples
5.0 / 5 (0 votes)