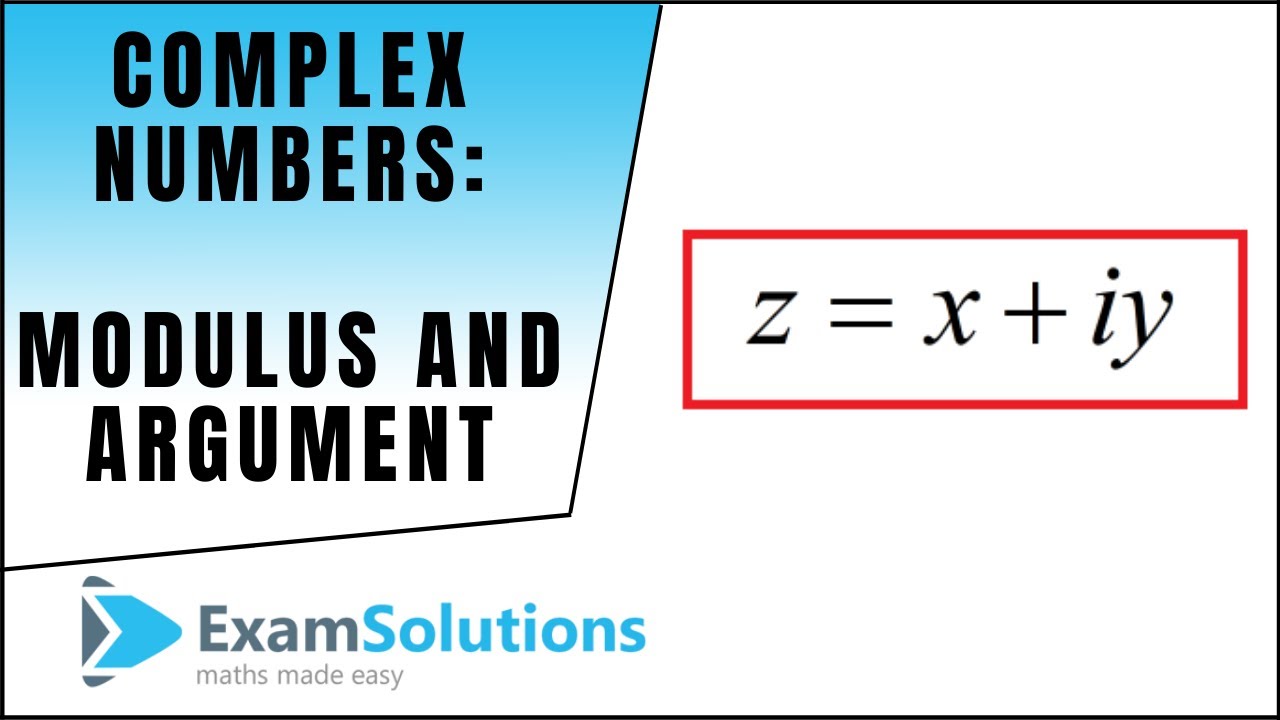
Bilangan Kompleks • Part 15: Modulus dan Argumen Bilangan Kompleks
Summary
TLDRIn this video, the concept of modulus and argument in complex numbers is explained. The modulus represents the length of the vector formed by a complex number on the Argand diagram, and is calculated using the Pythagorean theorem. The argument indicates the angle formed between the positive real axis and the vector, measured counterclockwise. The video explores how to determine the argument in different quadrants and how it repeats in cycles. The main argument is defined within a range from 0 to 2π. The video concludes by summarizing key points, such as the argument of real and imaginary numbers.
Takeaways
- 😀 The modulus of a complex number represents its magnitude or length in the complex plane, calculated as the square root of the sum of the squares of the real and imaginary parts.
- 😀 The argument of a complex number is the angle formed by the vector representing the complex number with the positive real axis, measured in a counterclockwise direction.
- 😀 Complex numbers can be visualized as vectors in the complex plane, with the real part corresponding to the x-axis and the imaginary part corresponding to the y-axis.
- 😀 The modulus of a complex number is denoted as |Z|, and it is the length of the vector from the origin to the point representing the complex number.
- 😀 The argument of a complex number is calculated using the inverse tangent function (arctan), expressed as θ = tan^(-1)(y/x).
- 😀 The argument of a complex number depends on the quadrant in which the point lies: quadrant I gives an argument between 0 and π/2, quadrant II gives between π/2 and π, and so on.
- 😀 The argument of a complex number is not unique because the angle repeats every 2π radians, meaning the argument can take multiple values depending on the integer k in the formula θ + 2kπ.
- 😀 The main argument of a complex number is the unique value of the argument between 0 and 2π, typically represented as Arg(Z).
- 😀 Special cases of arguments include: for real positive numbers, the argument is 0; for real negative numbers, the argument is π; and for pure imaginary numbers, the argument is either π/2 or 3π/2 depending on the sign of the imaginary part.
- 😀 To express the general argument, use the formula θ + 2kπ, where k is an integer, reflecting the periodic nature of trigonometric functions.
Q & A
What is the modulus of a complex number?
-The modulus of a complex number is the length or magnitude of the vector representing the complex number in the complex plane. It is the distance from the origin (0,0) to the point (x,y), and is calculated using the Pythagorean theorem as √(x² + y²).
How is the modulus of a complex number represented?
-The modulus of a complex number Z is represented as |Z| or |z|, which is equivalent to the length of the vector OP where O is the origin and P is the point corresponding to the complex number in the complex plane.
What does the argument of a complex number represent?
-The argument of a complex number represents the direction or angle of the vector in the complex plane. It is the angle θ between the positive real axis (x-axis) and the vector pointing to the complex number.
How is the argument of a complex number calculated?
-The argument θ of a complex number can be calculated using the formula θ = arctan(y/x), where x is the real part and y is the imaginary part of the complex number.
What is the principal argument of a complex number?
-The principal argument of a complex number is the unique value of the argument that lies between 0 and 2π (or 0° to 360°). It is the specific angle that describes the direction of the complex number within one full revolution in the complex plane.
What is the difference between argument and principal argument?
-The argument of a complex number can have multiple values, expressed as θ + k * 2π, where k is any integer, due to the periodic nature of trigonometric functions. The principal argument, however, is a single value between 0 and 2π (or 0° to 360°), representing the smallest angle in the standard range.
How are angles measured for complex numbers in different quadrants?
-Angles are measured from the positive real axis (x-axis) in a counterclockwise direction. In quadrant I (x > 0, y > 0), the argument is between 0 and π/2. In quadrant II (x < 0, y > 0), the argument is between π/2 and π. In quadrant III (x < 0, y < 0), the argument is between π and 3π/2. In quadrant IV (x > 0, y < 0), the argument is between 3π/2 and 2π.
What is the argument of a purely real positive number?
-The argument of a purely real positive number is 0, because the direction of the vector representing the number is aligned with the positive real axis.
What is the argument of a purely real negative number?
-The argument of a purely real negative number is π, since the vector points in the opposite direction to the positive real axis, along the negative real axis.
What is the argument of a purely imaginary number?
-For a purely positive imaginary number (e.g., 2i), the argument is π/2 because it points upward along the positive imaginary axis. For a purely negative imaginary number (e.g., -2i), the argument is 3π/2 because it points downward along the negative imaginary axis.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
Complex Numbers : Modulus and Argument | ExamSolutions

Mencari akar-akar dari Bilangan Kompleks

Grafik dan Nilai Mutlak Bilangan Kompleks

Bilangan Kompleks • Part 3: Definisi dan Menyatakan Bentuk Bilangan Kompleks
Data Representation - Mantissa And Exponents Part 3 - (A Level Computer Science Made Easy (A2) )

Convert Image into Matrix - Like a Pro!
5.0 / 5 (0 votes)
