Writing Quadratic Functions in Intercept Form
Summary
TLDRThis video tutorial focuses on writing quadratic functions in intercept form, defined as f(x) = a(x - p)(x - q), where p and q are the x-intercepts. The presenter explains how to determine the function using given intercepts and an additional point on the graph. Two examples illustrate the process of substituting values to find the constant 'a' and formulating the complete quadratic function. The video encourages viewers to practice and check their work with graphing tools, reinforcing the importance of mastering quadratic functions.
Takeaways
- 😀 The intercept form of a quadratic function is expressed as f(x) = a(x - p)(x - q), where p and q are the x-intercepts.
- 📈 To write a quadratic function, you need the x-intercepts and at least one additional point on the graph.
- 📝 It is helpful to label the intercepts (p and q) and the additional point (x, y) clearly before starting calculations.
- 🔍 When substituting values into the function, ensure to maintain clarity to avoid confusion with multiplication signs.
- ⚖️ Simplifying the expressions within the parentheses is key before solving for the coefficient a.
- 🧮 After finding the value of a, replace it in the function to express the complete quadratic equation.
- 🖥️ Verifying the resulting function by graphing is a useful step to confirm accuracy.
- 📊 The negative value of a indicates that the parabola opens downward, which can be inferred from the graph.
- 👩🏫 When given only the intercepts and a point, the same method of substitution and solving can be used to find the quadratic function.
- 🌟 Practice with different sets of intercepts and points can help solidify understanding of writing quadratic functions in intercept form.
Q & A
What is intercept form of a quadratic function?
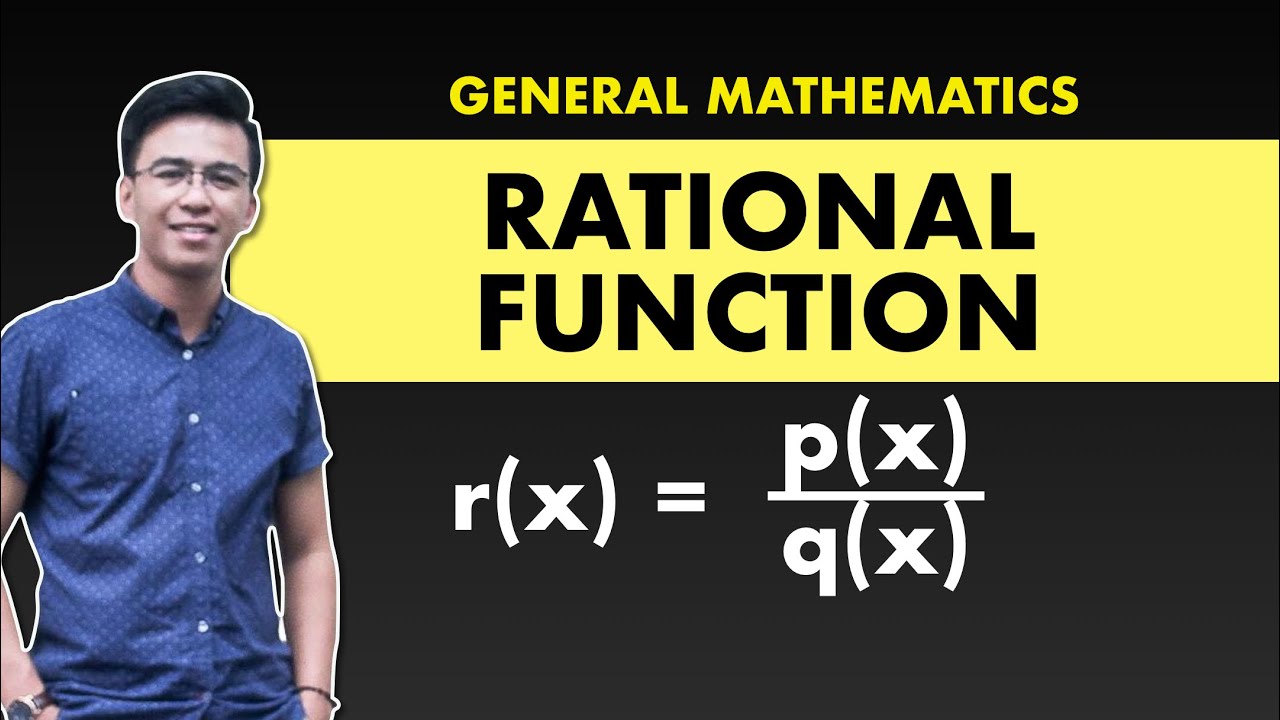
-The intercept form of a quadratic function is represented as f(x) = a(x - p)(x - q), where p and q are the x-intercepts.
What are the key components needed to write a quadratic function in intercept form?
-To write a quadratic function in intercept form, you need the x-intercepts (p and q) and another point on the graph.
In the example provided, what are the x-intercepts?
-In the example, the x-intercepts are (3, 0) and (5, 0).
What point was used to determine the value of 'a' in the first example?
-The point used to determine 'a' in the first example was (2, -3).
How is the variable 'a' calculated in the quadratic function?
-The variable 'a' is calculated by substituting the x-intercept values and the additional point into the equation, simplifying, and solving for 'a'.
What is the significance of the sign of 'a' in the quadratic function?
-The sign of 'a' indicates the direction the parabola opens: if 'a' is negative, the parabola opens downwards, and if 'a' is positive, it opens upwards.
What happens if you switch the values of p and q?
-Switching the values of p and q does not change the intercept form of the quadratic function; it remains valid as long as p and q are correctly identified as x-intercepts.
Why is it important to keep multiplication clear when writing the function?
-It is important to keep multiplication clear to avoid confusion, such as misinterpreting it as addition or subtraction, which can lead to errors in calculation.
What is the final quadratic function derived from the first example?
-The final quadratic function derived from the first example is f(x) = -1(x - 3)(x - 5).
What method can be used to verify the derived quadratic function?
-You can verify the derived quadratic function by graphing it using graphing tools like calculators or software (e.g., Desmos, GeoGebra) and checking if it matches the original graph.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
5.0 / 5 (0 votes)