INVERS MATRIKS ORDO 2x2
Summary
TLDRIn this video, viewers learn about the inverse of 2x2 matrices, building on previously covered determinants. The presenter explains the general formula for calculating the inverse, including how to determine the adjoint and the determinant. Through clear examples involving matrices A, B, and C, the video demonstrates step-by-step calculations of inverses, reinforcing key concepts like swapping elements and changing signs. The lesson concludes with an invitation to like, share, and subscribe for future content, promising a follow-up on 3x3 matrix inverses.
Takeaways
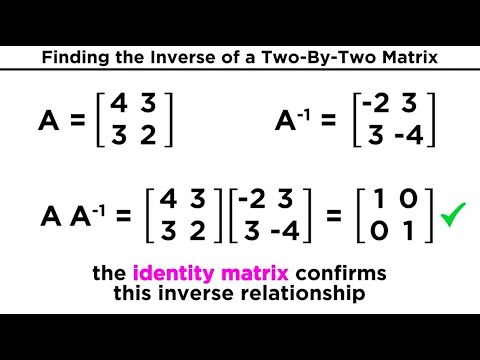
- 😀 Inverse of a matrix is denoted as A^{-1} and signifies a matrix that, when multiplied by A, yields the identity matrix.
- 📏 A 2x2 matrix consists of two rows and two columns, represented as A = [[a, b], [c, d]].
- 🔢 The determinant of a 2x2 matrix is calculated using the formula: det(A) = ad - bc.
- ✍️ A matrix has an inverse only if its determinant is non-zero.
- 🔄 The adjoint (or adjugate) of a matrix is formed by swapping the elements of the main diagonal and changing the signs of the off-diagonal elements.
- 📐 The formula for calculating the inverse of a 2x2 matrix is: A^{-1} = (1/det(A)) * adj(A).
- 🧮 To find the inverse, first calculate the determinant and then find the adjoint of the matrix.
- 📊 The example problem demonstrates the step-by-step calculation of the inverse for the matrix A = [[2, 3], [5, 7]].
- ➖ The calculated determinant for the example matrix A was -1, indicating it has an inverse.
- ✅ The final result of the inverse for the example matrix A was A^{-1} = [[-7, 3], [5, -2]].
Q & A
What is the main topic of the video?
-The main topic of the video is learning about the inverse of 2x2 matrices.
What was discussed in the previous video?
-The previous video covered the determinants of matrices, specifically for 2x2 and 3x3 orders.
How is the inverse of a 2x2 matrix represented mathematically?
-The inverse of a matrix A is denoted as A⁻¹, which indicates the inverse operation.
What formula is used to calculate the inverse of a 2x2 matrix?
-The formula for the inverse of a 2x2 matrix A is given by: A⁻¹ = 1/det(A) * adj(A), where det(A) is the determinant of A and adj(A) is the adjoint of A.
What is the determinant of a 2x2 matrix defined as?
-The determinant of a 2x2 matrix with elements a, b, c, and d is defined as: det(A) = ad - bc.
How does one find the adjoint of a 2x2 matrix?
-To find the adjoint of a 2x2 matrix, swap the positions of a and d, and negate the positions of b and c.
What is the example matrix A used to explain the inverse calculation?
-The example matrix A used is [[2, 3], [5, 7]].
What is the final result of the inverse of matrix A?
-The inverse of matrix A is calculated to be [[-7, 3], [-5, -2]].
What is the formula used for the inverse of matrix B in the second example?
-For matrix B, the inverse is calculated using the same formula: B⁻¹ = 1/det(B) * adj(B).
What values were assigned to the elements of matrix C in the video?
-In the example for matrix C, the values are [-3, 2], [7, -5].
What is the final result of the inverse of matrix C?
-The inverse of matrix C is found to be [[-5, -2], [-7, -3]].
What invitation is given to viewers at the end of the video?
-Viewers are encouraged to like, share, and subscribe to the channel for future videos, along with turning on notifications.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

MATRIZ INVERSA | Cálculo da Matriz Inversa

Cara Menentukan Invers Matriks

Matematika SMA - Matriks (5) - Determinan Matriks 2x2, Invers Matriks 2x2 (A)
Inverse Matrices and Their Properties

Matriks Matematika Wajib Kelas 11 Bagian 3 - Determinan Matriks Ordo 2x2 dan 3x3 dengan cara Sarrus

Matriks Matematika Kelas 11 • Part 23: Menyelesaikan SPLTV dengan Metode Determinan Matriks
5.0 / 5 (0 votes)
