BE3002 Transport Phenomena in Biosystem_Module 6_Segment 2
Summary
TLDRThis segment explores the application of equations of change in isothermal systems to solve flow problems involving Newtonian fluids. It outlines key equations like continuity, motion, and viscosity, emphasizing their role in determining pressure and velocity distributions. The discussion focuses on simplifying complex problems by formulating postulates about flow behavior and applying boundary conditions. Examples, such as flow through circular tubes and falling films, illustrate these principles in action. The segment sets the stage for further exploration of dimensional analysis in fluid dynamics.
Takeaways
- 😀 The segment focuses on applying equations of change to solve flow problems in isothermal systems involving Newtonian fluids.
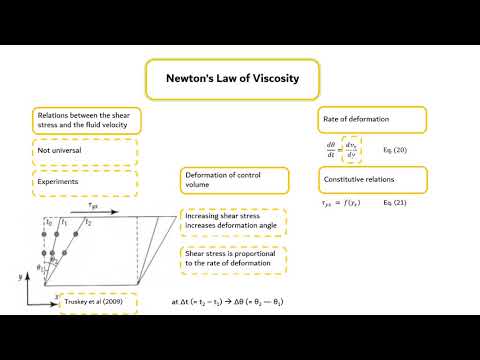
- 📚 Key equations include the equation of continuity, the equation of motion, shear stress components, the equation of state, and viscosity equations.
- 🔍 Understanding flow requires using these equations alongside boundary and initial conditions to determine pressure, density, and velocity distributions.
- ✂️ For fluids with constant density and viscosity, the continuity equation and Navier-Stokes equations can simplify the analysis of flow problems.
- 📝 Familiarity with important tables in the textbook is essential for students to set up and solve flow equations effectively.
- 🧩 Making postulates about pressure and velocity relationships helps to eliminate unnecessary terms from the equations, simplifying problem-solving.
- 🚀 The segment discusses problem-solving techniques for stable flows, including examples like flow through circular tubes and falling films.
- 📈 Integration of relevant equations leads to general solutions for pressure and velocity distributions, refined using boundary conditions.
- 💡 The next segment will delve into dimensional analysis, further enhancing understanding of the equations of change.
- ⚖️ Assumptions made during analysis can significantly impact the simplicity and solvability of fluid dynamics problems.
Q & A
What is the main focus of this segment on transport phenomena in biosystems?
-The segment focuses on applying the equations of change to solve flow problems in isothermal systems, specifically for Newtonian fluids at constant temperature.
Which key equations are necessary for describing fluid flow in this context?
-The key equations discussed include the equation of continuity, the equation of motion, the components of viscosity (tau), and the equations of state.
What role do initial and boundary conditions play in fluid dynamics?
-Initial and boundary conditions are crucial for determining the pressure, density, and velocity distributions within a fluid.
Why should beginning students not worry about the derivation of equations?
-Beginning students should focus on familiarizing themselves with the tables of equations provided in the textbook rather than the derivation process, as they need to understand how to set up and apply these equations to solve flow problems.
What assumptions are made when setting up equations for fluid flow problems?
-Assumptions include postulating the forms of pressure and velocity distributions based on the specific problem, allowing the elimination of unnecessary terms from the equations.
How does the lecturer suggest approaching the flow through a circular tube?
-The lecturer suggests postulating that the velocity is a function of radial and axial positions, which leads to simplified equations for pressure and velocity distributions.
What is the significance of the analytical solutions discussed in the segment?
-The analytical solutions serve as a preparation for tackling more complex fluid dynamics problems, including numerical solutions and dimensional analysis.
In the context of falling film flow, what postulates are made regarding the flow?
-In falling film flow, it is postulated that there is steady-state flow with constant density and that the x and y components of the velocity are zero, while vz is a function of x.
What is the expected outcome of integrating the equations discussed?
-Integrating the equations leads to expressions for pressure and velocity distributions that can be determined using boundary conditions.
What will be discussed in the next segment of the module?
-The next segment will cover dimensional analysis of the equations of change, expanding on the concepts introduced in this segment.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

The million dollar equation (Navier-Stokes equations)
BE3002 Transport Phenomena in Biosystem Module 2_Segment 4

Thermodynamics 12 - Steady Flow Process

DIFFERENCE BETWEEN NEWTONIAN AND NON-NEWTONIAN FLUIDS

Rheology Part 3 - Flow Profiles - A Video Tutorial by samMorell.com

LECTURE NOTES: AIRCRAFT AERODYNAMICS I, CHAPTER I, PART 3
5.0 / 5 (0 votes)
