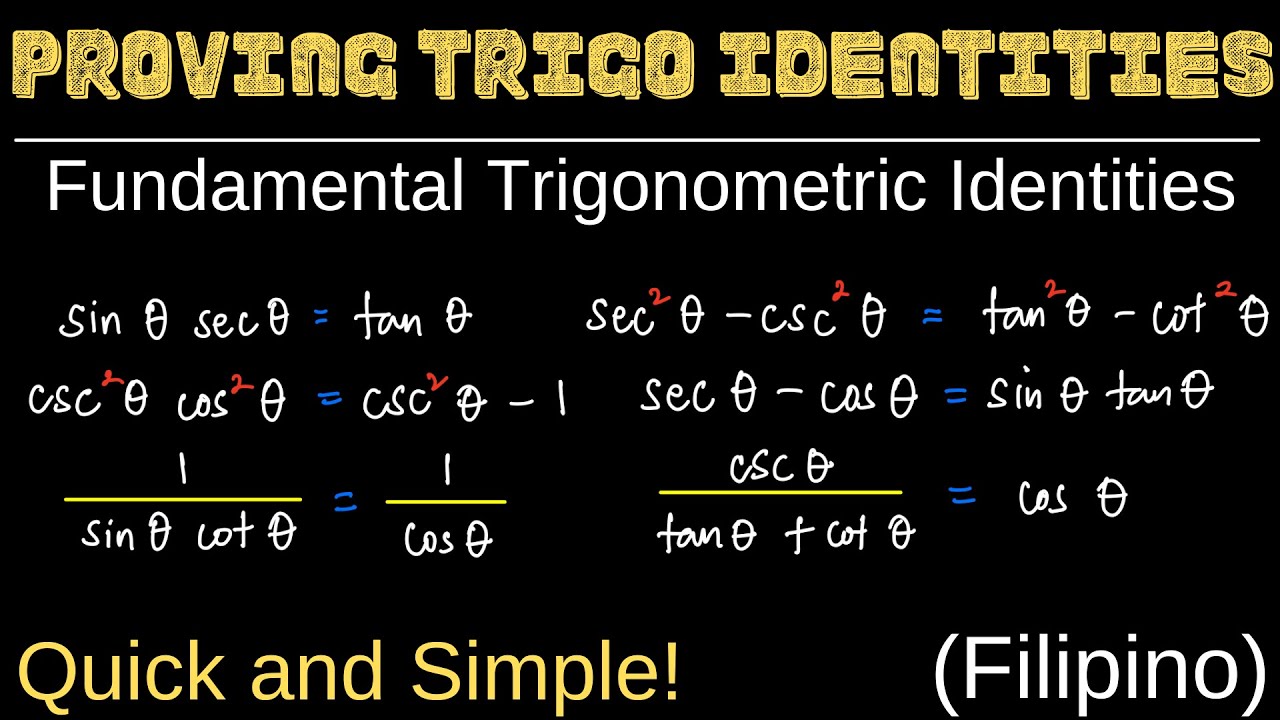
Soal-soal Identitas Trigonometri
Summary
TLDRIn this video, the Math channel discusses trigonometric identities and how to prove them through various examples. The presenter explains step-by-step solutions to problems involving identities like cos²X(1 + tan²X) = 1, and demonstrates the process of simplifying trigonometric expressions to make both sides of the equation identical. The video emphasizes understanding fundamental trigonometric transformations, offering clear explanations to help viewers grasp the concepts. The host encourages interaction through likes, shares, and comments, and hopes the content will serve as a beneficial learning resource.
Takeaways
- 📘 The video is a tutorial on solving trigonometric identity problems.
- 💡 The first problem involves proving that cos²(X) * (1 + tan²(X)) = 1 by manipulating the left-hand side.
- 🔧 The approach involves substituting tan²(X) with sin²(X) / cos²(X) and simplifying the expression step by step.
- ✅ After simplification, the left-hand side equals 1, proving the identity.
- 📚 The second example focuses on proving that (1 + tan²(X))(1 - cos²(X)) = tan²(X), again by manipulating the left side.
- 🔄 Similar to the first problem, trigonometric identities are used, and terms are simplified until both sides are equal.
- 📏 The third problem is to prove that cos²(X) / (1 - sin(X)) = 1 + sin(X), requiring factorization and cancellation.
- ✔️ The fourth problem demonstrates proving (1 - tan²(X)) / (1 + tan²(X)) = cos²(X) - sin²(X), using algebraic manipulation and trigonometric identities.
- 🔗 The speaker emphasizes using trigonometric identities such as sin²(X) + cos²(X) = 1 to simplify equations.
- 🙏 The video concludes with the hope that viewers find the content beneficial and reminds them to like, share, and subscribe.
Q & A
What is the main topic discussed in the video?
-The video primarily discusses solving problems related to trigonometric identities, focusing on proving various trigonometric equations.
What is the first trigonometric identity problem presented?
-The first problem asks to prove that cos²(X) * (1 + tan²(X)) = 1.
How is the expression tan²(X) simplified in the first problem?
-The expression tan²(X) is simplified to sin²(X) / cos²(X), based on trigonometric identities.
What is the approach used to solve the first problem?
-The approach involves simplifying the left-hand side by distributing cos²(X) to both terms inside the parentheses and then using the identity sin²(X) + cos²(X) = 1.
What is the second trigonometric problem presented in the video?
-The second problem is to prove that (1 + tan²(X))(1 - cos²(X)) = tan²(X).
How is cos²(X) treated in the second problem?
-Cos²(X) is left unchanged on the right-hand side, while the left-hand side is simplified by expanding and then applying trigonometric identities.
What trigonometric identity is used in the second problem to simplify the expression?
-The identity sin²(X) + cos²(X) = 1 is used to simplify parts of the expression during the proof.
What is the third trigonometric identity problem mentioned?
-The third problem asks to prove that cos²(X) / (1 - sin(X)) = 1 + sin(X).
How is the third problem approached in terms of solving?
-The approach involves factoring and canceling terms in the numerator and denominator, using the identity cos²(X) = 1 - sin²(X).
What is the fourth and final trigonometric identity problem in the video?
-The fourth problem asks to prove that (1 - tan²(X)) / (1 + tan²(X)) = cos²(X) - sin²(X).
What mathematical operation is applied in the fourth problem to simplify the expressions?
-In the fourth problem, division of fractions is simplified by multiplying by the reciprocal, and then common terms are canceled out.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
5.0 / 5 (0 votes)