Representing Real-Life Situations Using RATIONAL FUNCTIONS (Senior High School General Mathematics)
Summary
TLDRIn this general math tutorial, TeacherNet introduces rational functions, which are composed of polynomials with non-negative, integral exponents. The video explains that a rational function is a ratio of two polynomials, with the denominator not equaling zero. Practical applications are demonstrated, such as dividing a fixed family budget among members and calculating the rate of planting trees. The script uses real-life examples to illustrate how rational functions are relevant and ubiquitous in daily life, aiming to help viewers understand and appreciate their importance.
Takeaways
- 📚 A polynomial function is defined as a set of terms with non-negative, integral exponents.
- 🔢 Examples of polynomial functions include f(x) = 2x^5 + 1, f(x) = (1/5)x^2 - 8x + 4, and h(x) = -15, where the exponents are positive integers or zero.
- ❌ Functions with fractional or negative exponents, like f(x) = 3x^(1/4) + 6 or g(x) = x^(-4) - 3, are not polynomial functions.
- 🔍 A rational function is expressed as r(x) = t(x) / q(x), where both t(x) and q(x) are polynomial functions, and q(x) ≠ 0.
- ⚠️ Rational functions have restrictions on the domain to avoid division by zero in the denominator.
- 💡 Real-life applications of rational functions include scenarios where a fixed amount is distributed among a variable number of recipients.
- 👨👩👧👦 An example of a rational function in real life is the division of a family's monthly food budget among varying numbers of family members.
- 🌳 Another example is the rate of planting trees, where the rate of work is inversely proportional to the amount of work done.
- 📉 As the number of family members (x) increases, the amount of money (f(x)) each member gets for food decreases, following the function f(x) = 15000 / x.
- 📈 Conversely, as the amount of work (w) in minutes increases, the rate of work (r(w)) decreases, following the function r(w) = w / 30.
- 🌐 Rational functions are ubiquitous in everyday life, often unnoticed, but essential for understanding various proportional relationships.
Q & A
What is a rational function according to the script?
-A rational function is a function in the form r(x) = t(x) / q(x), where both t(x) and q(x) are polynomial functions and q(x) should not equal zero.
What is a polynomial function and what are the requirements for the exponents in such a function?
-A polynomial function is a term or set of terms with non-negative, integral exponents. The exponents must be positive integers or zero.
Why is the function f(x) = 3x^(1/4) + 6 not considered a polynomial function?
-The function f(x) = 3x^(1/4) + 6 is not a polynomial function because the exponent 1/4 is not an integer.
What is the restriction on the variable x for the rational function r(x) = (3x^2 + 2x - 1) / (x + 1)?
-The restriction is that x should not equal -1 because if x is -1, the denominator (x + 1) becomes zero, which is undefined in real numbers.
How does the script describe the relationship between the number of family members and the amount of money spent on food per member?
-The script describes it as a rational function where f(x) = 15,000 / x, with x being the number of family members and f(x) being the amount of money spent on food per member.
What happens to the value of f(x) as the number of family members (x) increases?
-As the number of family members (x) increases, the value of f(x), which represents the amount of money spent on food per member, decreases.
What is the rate of work r(w) as a function of the amount of work done w in minutes, according to the script?
-The rate of work r(w) is expressed as a function of the amount of work done w in minutes as r(w) = w / 30.
How does the script illustrate the concept of a rational function using the example of planting trees?
-The script uses the example of planting trees to illustrate that as the rate of work (r(w)) increases, the amount of work done (w) increases, and vice versa, forming a rational function relationship.
What is the rate of work (r(w)) when two trees are planted, according to the script's example?
-When two trees are planted, the rate of work (r(w)) is 1/15, as it takes 30 minutes to plant one tree, so 2 trees would take 15 minutes on average.
How does the script emphasize the practical application of rational functions in everyday life?
-The script emphasizes the practical application of rational functions by providing real-world examples such as family budgeting for food and the rate of work in planting trees.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

What are rational expressions

Polinomial (Bagian 1) - Pengertian dan Operasi Aljabar Polinomial Matematika Peminatan Kelas XI

Materi Polinomial kelas XI MIPA Matematika Peminatan Pertemuan 1- PPL 2 PPG Prajabatan Gel 1
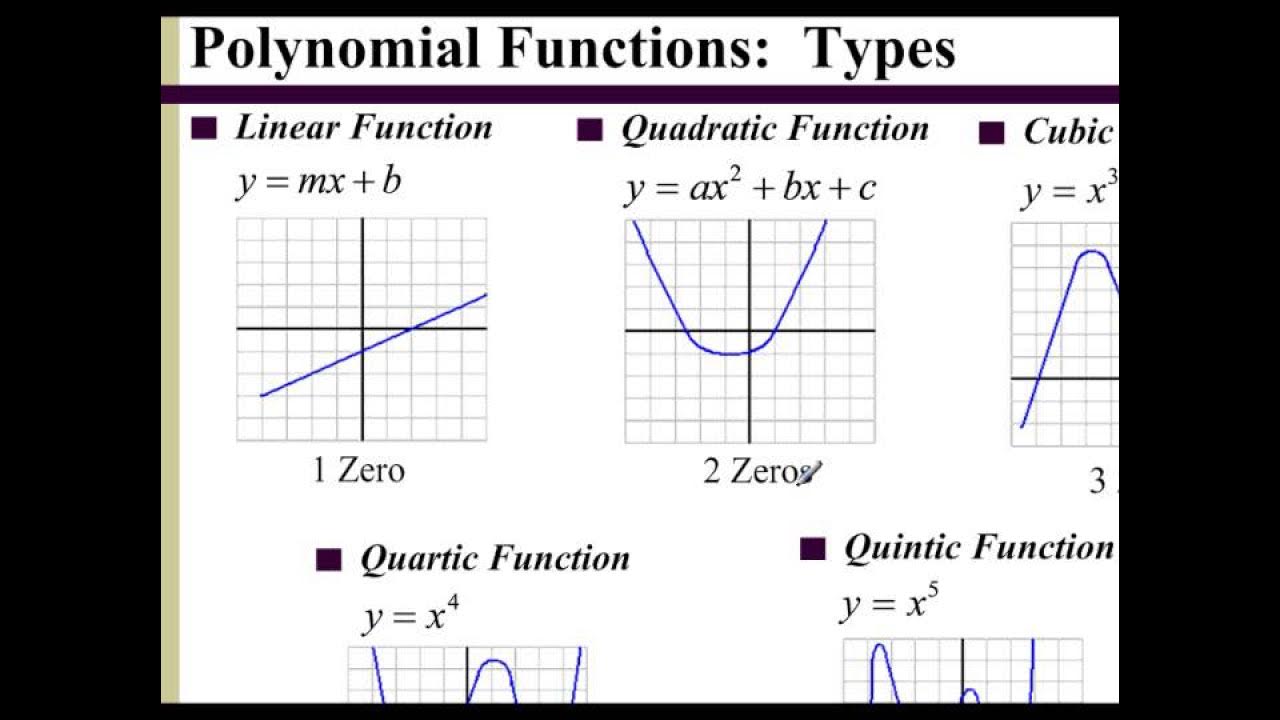
Lesson 6.3 - Identifying a Polynomial Function from the Graph

Ganzrationale Funktionen (Polynomfunktionen) - Einführung / Grundlagen
Rational Functions | General Mathematics
5.0 / 5 (0 votes)
