Media Pembelajaran Matematika Materi Peluang

Summary

In this educational video, Ibu Geisha Sonarita introduces the concept of probability, explaining its significance in predicting future events. She covers the definition of probability, sample space, and experiments, providing relatable examples such as rolling dice and tossing coins. The lesson walks through methods to list sample spaces, including using lists, tree diagrams, and tables. The video also explains how to calculate the probability of events using a formula. Real-life examples, like weather forecasts and board games, help reinforce the concepts. The video concludes with a brief recap and interactive exercises for viewers.

Takeaways

• 😀 Probability is the measure of how likely an event is to occur, with values ranging from 0 (impossible) to 1 (certain).
• 😀 In everyday life, probability is applied in things like weather forecasting, where past events help predict future ones.
• 😀 An **experiment** is a process that produces results, such as tossing a coin or rolling a die.
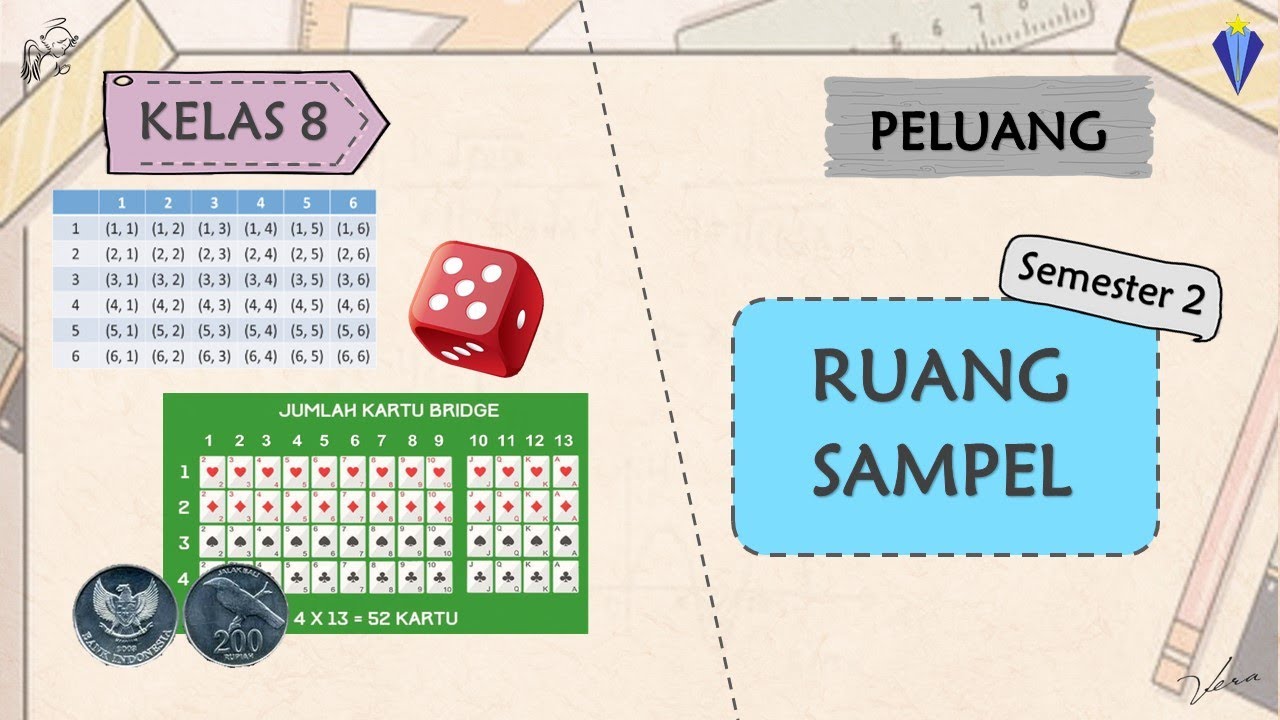
• 😀 The **sample space** refers to all possible outcomes of an experiment, and its size is denoted by 'NS'.
• 😀 For example, rolling a single die has a sample space of {1, 2, 3, 4, 5, 6}, so NS = 6.
• 😀 To determine a sample space, we can list all outcomes, use a tree diagram, or create a table, depending on the number of outcomes.
• 😀 A **tree diagram** helps visualize outcomes in experiments with multiple steps, like tossing a coin and rolling a die.
• 😀 When there are many possible outcomes, a **table** can be a helpful way to organize and represent them.
• 😀 The **probability formula** is P(A) = n(A) / n(S), where P(A) is the probability of an event, n(A) is the number of favorable outcomes, and n(S) is the total outcomes in the sample space.
• 😀 A probability of 0 indicates an impossible event (e.g., humans flying without technology), while a probability of 1 means the event will certainly occur (e.g., death).

Q & A

What is the definition of probability in mathematics?

In mathematics, probability refers to the method used to estimate the likelihood of an event occurring. It is a way to predict the chances of something happening.

How is probability applied in real life?

An example of probability in real life is weather forecasting. Organizations like BMKG predict the weather by analyzing past events and their causes to estimate the probability of similar events occurring again.

What are the key concepts to understand when studying probability?

The key concepts in probability include experiments and sample spaces. An experiment is an activity conducted to obtain a specific result, like tossing a coin or rolling a die. A sample space is the set of all possible outcomes of an experiment.

What is a sample space, and can you provide an example?

A sample space is the set of all possible outcomes from an experiment. For example, when rolling a die, the sample space consists of the numbers 1, 2, 3, 4, 5, and 6.

What are the three methods used to list the elements of a sample space?

The three methods to list the elements of a sample space are: 1) listing them directly, 2) using a tree diagram, and 3) using a table.

How does a tree diagram help in determining a sample space?

A tree diagram helps when the sample space is relatively large. For example, when tossing a coin and rolling a die, it visually maps all possible combinations of outcomes, such as 'Heads-1', 'Heads-2', 'Tails-1', and so on.

Why is a table used to list the sample space?

A table is used when the sample space is very large. It helps organize outcomes in a systematic manner, like when rolling two dice, where the sample space can be represented in a 6x6 grid.

What does a probability value of 0 mean?

A probability value of 0 means the event is impossible. For example, a person flying unaided is impossible with current technology, so its probability is 0.

What does a probability value of 1 mean?

A probability value of 1 means the event is certain to happen. An example is that every human will eventually die, so the probability of this event occurring is 1.

How is the probability of an event calculated?

The probability of an event is calculated using the formula P(A) = n(A) / NS, where P(A) is the probability of event A, n(A) is the number of favorable outcomes, and NS is the total number of outcomes in the sample space.