Dao động điều hòa: Chu kì. Tần số. Tần số góc. Vận tốc và gia tốc của vật dao động điều hòa
Summary
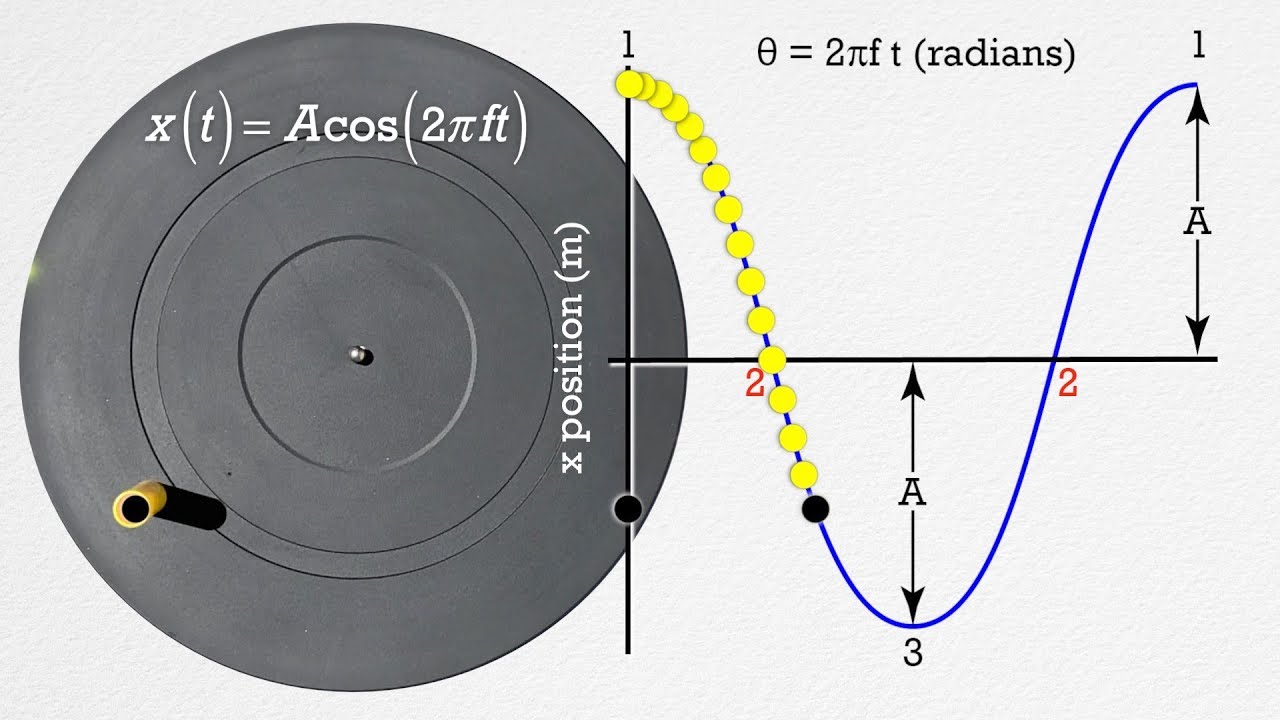
TLDRIn this lesson, students explore the relationship between simple harmonic motion and uniform circular motion. The teacher uses a model of circular motion and its shadow to introduce key concepts such as amplitude, frequency, period, velocity, and acceleration. The lesson covers how the period is the time for a complete oscillation, the inverse relationship between period and frequency, and how angular velocity (omega) relates to harmonic motion. Students learn the formulas for velocity and acceleration in oscillatory motion, recognizing that acceleration always points toward equilibrium, even as velocity changes direction during the motion.
Takeaways
- 📚 The lesson explores the connection between harmonic oscillation and uniform circular motion.
- 🔄 A full oscillation is completed when the shadow of a uniformly moving object returns to its original position.
- ⏱️ The period of oscillation is defined as the time taken for one complete oscillation or one full rotation in circular motion.
- 🔢 The frequency is the inverse of the period, indicating the number of oscillations in a unit of time.
- 🔧 Frequency is measured in hertz (Hz), representing oscillations per second.
- 🌀 Angular velocity (omega) is the rate of change of angular displacement during uniform circular motion.
- 🧮 In harmonic motion, angular velocity is known as angular frequency and is involved in the oscillation equation.
- 🚀 Velocity changes with position: it's zero at the extremities and maximum at the equilibrium position.
- ⚖️ Acceleration is always directed toward the equilibrium position and is proportional to the displacement.
- 📊 The relationship between acceleration and displacement is represented by a = -ω²x, showing acceleration as a restoring force.
Q & A
What is the relationship between uniform circular motion and harmonic oscillation as introduced in the lesson?
-The relationship between uniform circular motion and harmonic oscillation is demonstrated through the model where the shadow of an object in uniform circular motion creates a harmonic oscillation. The key quantities involved include amplitude and initial phase.
What is the definition of the period (T) in harmonic oscillation?
-The period (T) is defined as the time taken for an object to complete one full oscillation and return to its initial position and direction. In terms of uniform circular motion, it is the time taken for the object to complete one full revolution.
How is the period of oscillation calculated if the object completes 'n' full oscillations in a given time?
-The period (T) is calculated by dividing the time (Δt) by the number of full oscillations (n): T = Δt / n.
What is the frequency (f) of oscillation, and how is it related to the period?
-The frequency (f) of oscillation represents the number of oscillations per unit of time and is calculated as the inverse of the period: f = 1/T. It is measured in Hertz (Hz), which is equivalent to oscillations per second.
What is angular velocity (ω) in uniform circular motion, and how is it connected to harmonic oscillation?
-Angular velocity (ω) is the rate of change of the angle swept by an object in uniform circular motion and is given by ω = 2π/T. In harmonic oscillation, ω is referred to as angular frequency and plays a key role in the oscillation equation X = A cos(ωt + φ).
How is the velocity of an object in harmonic oscillation related to its displacement?
-The velocity of the object in harmonic oscillation is the time derivative of displacement (x). At the extremes (amplitude), velocity is zero, and at the equilibrium position, velocity is at its maximum (v = ωA).
How does acceleration behave in harmonic oscillation?
-The acceleration of the object in harmonic oscillation is the second derivative of displacement and is proportional to displacement but in the opposite direction. The equation is a = -ω²x, meaning acceleration always points towards the equilibrium position.
How do velocity and acceleration vectors behave in harmonic oscillation?
-In harmonic oscillation, the velocity vector is always directed along the direction of motion. In contrast, the acceleration vector always points towards the equilibrium position, even when the object is moving away from it.
What happens to the velocity and acceleration when the object moves from the amplitude position towards the equilibrium?
-As the object moves from the amplitude (extreme) position towards the equilibrium, its velocity increases, and both velocity and acceleration point towards the equilibrium position.
What changes occur in the velocity and acceleration when the object moves from the equilibrium to the amplitude position?
-When the object moves from the equilibrium to the amplitude position, the velocity decreases, and while the velocity vector points towards the amplitude, the acceleration vector still points towards the equilibrium position.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة

Comparing Simple Harmonic Motion(SHM) to Circular Motion - Demonstration

Pengantar Materi Getaran dan Gelombang pada Mata Kuliah Fisika Dasar ITK 2020/2021

Dạng 1 Vận tốc của vật dao động điều hòa

Simple Harmonic Motion(SHM) - Graphs of Position, Velocity, and Acceleration

MATERI KINEMATIK kelas 11 bag 8 GERAK MELINGKAR BERATURAN GMB K Merdeka
Simple Harmonic Motion(SHM) - Position Equation Derivation
5.0 / 5 (0 votes)
