Piecewise Function Domain Range Quadratic Linear Constant
Summary
TLDRIn this video, Anil Kumar explores the domain and range of a piecewise function with three different parts. He sketches the graph of the function to better understand its behavior, discussing how to plot each segment. Kumar explains the domain and range using interval notation and set notation, highlighting discontinuities at specific points. He encourages viewers to practice writing domain and range in different notations and explains the process of identifying key points through tables and graphing techniques. The video emphasizes understanding piecewise functions, their domain, and range.
Takeaways
- 📊 The function H(x) is a piecewise function consisting of three parts.
- 🧮 First piece: H(x) = 2x + 2 for x < -2.
- 🧮 Second piece: H(x) = x² for -1 ≤ x ≤ 2.
- 🧮 Third piece: H(x) = 3 for x > 2.
- 📈 A graph of the function helps in visualizing and understanding the domain and range.
- 🔵 The domain of the function is from -∞ to ∞, with specific ranges for each piece.
- 🔢 The range of the function is from -∞ to 4, with 4 being the highest value in the second piece.
- ⚠️ There are two discontinuities in the function: between x = -2 and x = -1, and at x = 2.
- 📚 Set notation and interval notation can be used to describe the domain and range.
- 📝 As an exercise, the domain and range can be written in different notations, like set notation.
Q & A
What is the given piecewise function in the video?
-The piecewise function is H(x), which consists of three parts: H(x) = 2x + 2 when x < -2, H(x) = x² for -1 ≤ x ≤ 2, and H(x) = 3 for x > 2.
What is the first step suggested in understanding the piecewise function?
-The first step is to sketch the function by plotting the different pieces on a graph to visualize how they are connected.
How is the function H(x) = 2x + 2 represented for x < -2?
-For x < -2, the function H(x) = 2x + 2 is a straight line. A table of values is made for points such as x = -2, -3, and -4. The function is plotted with an open circle at x = -2 since the value is not included in this piece.
How is the second piece of the function (x²) plotted for -1 ≤ x ≤ 2?
-The second piece, H(x) = x², is plotted as a parabola between x = -1 and x = 2. A table of values is made for points like -1, 0, 1, and 2, and the points are connected with filled circles because the endpoints are included.
What does the third piece of the function (H(x) = 3 for x > 2) represent?
-The third piece is a constant function where H(x) = 3 for all x greater than 2. It is represented as a horizontal line starting from x = 2 (open circle) and extending to the right.
How is the domain of the piecewise function written in interval notation?
-The domain is written as the union of three intervals: (-∞, -2) ∪ [-1, 2] ∪ (2, ∞). This indicates that the function is defined for all real numbers except for the gaps between the intervals.
How is the range of the piecewise function written in interval notation?
-The range is written as (-∞, 4], meaning the output values of the function extend from negative infinity to 4, with 4 being the maximum value.
Where are the discontinuities in the piecewise function?
-There are two discontinuities in the function: one at x = -2 (between the first and second pieces) and a jump discontinuity at x = 2 (between the second and third pieces).
What type of discontinuity is observed at x = 2?
-At x = 2, there is a jump discontinuity because the function drops from H(x) = 4 in the second piece to H(x) = 3 in the third piece.
Why is using a table of values helpful when sketching piecewise functions?
-Using a table of values helps plot specific points for each piece of the function accurately, especially when dealing with different types of functions, such as straight lines and parabolas.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة
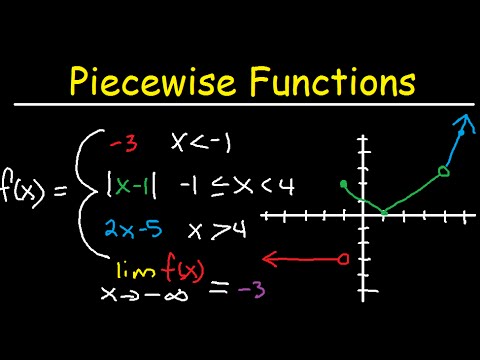
Graphing Piecewise Functions, Domain & Range - Limits, Continuity, & Absolute Value ,

Relasi dan Fungsi Matematika Kelas 10 • Part 12: Fungsi Sepotong-Sepotong / Piecewise Function

SHS General Mathematics Q1 Ep1: Function
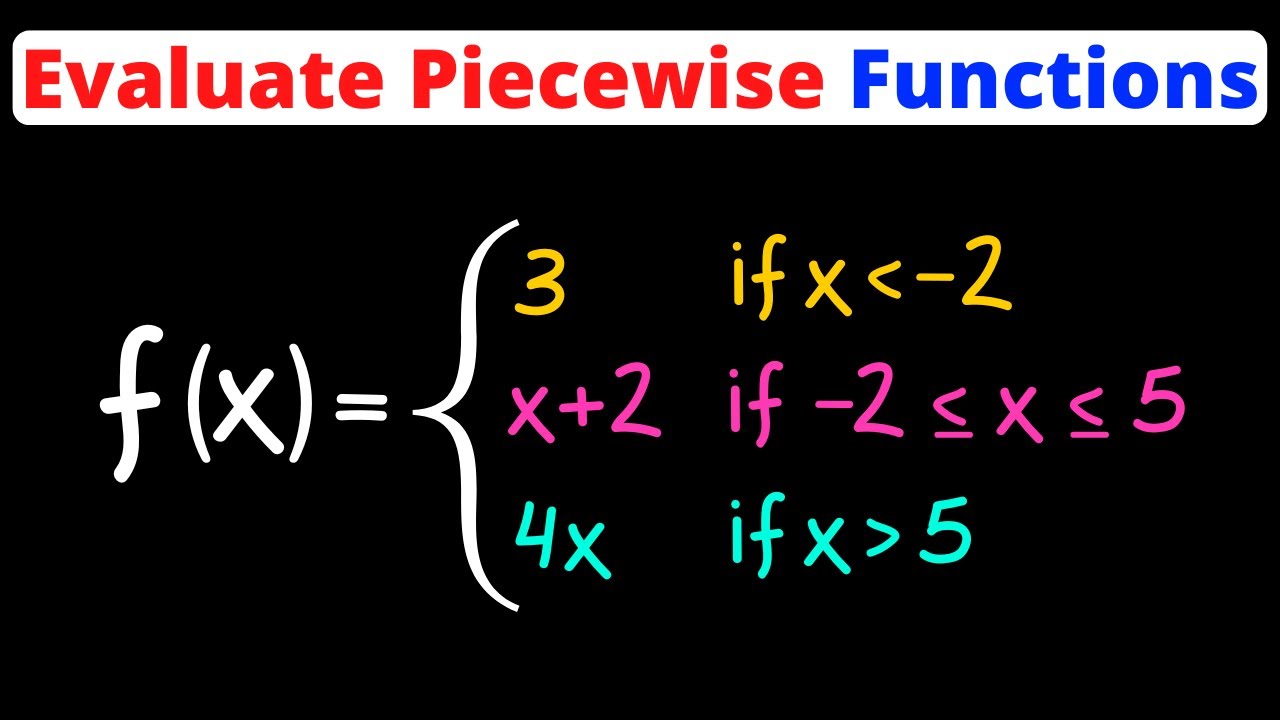
Evaluate a Piecewise Function | Eat Pi

Apa sih yang Dimaksud dengan Fungsi dalam Matematika? | Kalkulus 1

Functions and Graphs | Precalculus
5.0 / 5 (0 votes)
