Combined Gas Law

Summary

This educational video script introduces the Combined Gas Law, which unifies Boyle's Law, Charles's Law, and Gay-Lussac's Law to describe the relationship between pressure, volume, and temperature of a gas. The script walks through an example problem where a gas's initial conditions and changes in pressure and temperature are given, and the new volume is calculated using the Combined Gas Law. The process involves converting temperatures to Kelvin, setting up the equation, and solving for the unknown volume, resulting in a new volume of 580.58 liters.

Takeaways

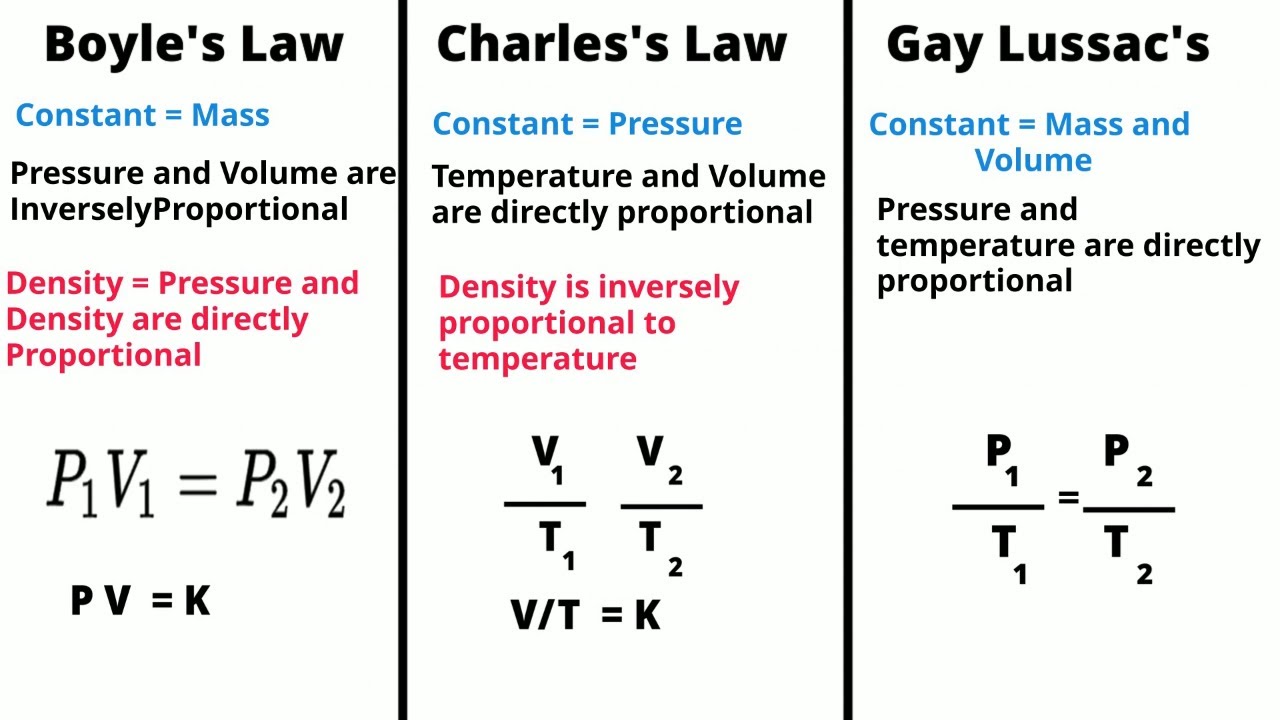
• 📚 The lesson introduces three fundamental gas laws: Boyle's Law, Charles's Law, and Gay-Lussac's Law, which describe the relationships between pressure, volume, and temperature of a gas.
• 🔍 Boyle's Law relates pressure and volume, stating that at a constant temperature, the pressure of a gas is inversely proportional to its volume.
• 🌡️ Charles's Law connects volume and temperature, indicating that at a constant pressure, the volume of a gas is directly proportional to its temperature in Kelvin.
• 🔄 Gay-Lussac's Law shows the relationship between pressure and temperature, asserting that at a constant volume, the pressure of a gas is directly proportional to its temperature in Kelvin.
• 🔗 The Combined Gas Law is formed by combining Boyle's, Charles's, and Gay-Lussac's Laws, accounting for changes in pressure, volume, and temperature simultaneously.
• 🧩 The formula for the Combined Gas Law is \( \frac{P_1V_1}{T_1} = \frac{P_2V_2}{T_2} \), where \( P \) stands for pressure, \( V \) for volume, and \( T \) for temperature in Kelvin.
• 📘 An example problem is presented involving a gas at 110 kilopascals and 30°C with an initial volume of 2 liters, which is then heated to 80°C and the pressure increased to 440 kilopascals.
• ⚖️ The problem requires converting Celsius temperatures to Kelvin by adding 273, which is done for both the initial and final temperatures.
• 🔢 The solution process involves substituting the given values into the Combined Gas Law equation and solving for the unknown variable, which in this case is the new volume (\( V_2 \)) of the gas.
• 📝 The calculation involves cross-multiplying and isolating \( V_2 \) to find the new volume, which is determined to be 0.58 liters.
• 📚 The lesson concludes with a demonstration of how to apply the Combined Gas Law to practical problems involving changes in the state of a gas.

Q & A

What are the three gas laws mentioned in the script?

The three gas laws mentioned are Boyle's Law, Charles's Law, and Gay-Lussac's Law.

What does Boyle's Law relate to?

Boyle's Law relates the pressure and volume of a particular gas, stating that at a constant temperature, the pressure of a gas is inversely proportional to its volume.

What is the relationship described by Charles's Law?

Charles's Law relates the volume and temperature of a particular gas, stating that at a constant pressure, the volume of a gas is directly proportional to its temperature in Kelvin.

How does Gay-Lussac's Law connect pressure and temperature?

Gay-Lussac's Law states that at a constant volume, the pressure of a gas is directly proportional to its temperature in Kelvin.

What is the Combined Gas Law and why is it used?

The Combined Gas Law is a single law that combines Boyle's Law, Charles's Law, and Gay-Lussac's Law to describe the relationship between pressure, volume, and temperature of a gas when all three variables are changing.

What formula represents the Combined Gas Law?

The formula for the Combined Gas Law is P1V1/T1 = P2V2/T2, where P1 and P2 are the initial and final pressures, V1 and V2 are the initial and final volumes, and T1 and T2 are the initial and final temperatures in Kelvin.

What is the initial condition of the gas in the example problem?

The initial condition of the gas in the example is 110 kilopascals pressure, 30°C temperature, and 2 liters volume.

How is the temperature converted from Celsius to Kelvin?

The temperature is converted from Celsius to Kelvin by adding 273 to the Celsius temperature.

What are the final conditions of the gas in the example problem after the changes?

The final conditions are 440 kilopascals pressure and 80°C temperature, with the volume to be determined.

How is the new volume calculated in the example problem?

The new volume is calculated by using the Combined Gas Law formula and cross-multiplying to isolate V2, the unknown variable.

What is the final answer for the new volume of the gas in the example problem?

The final answer for the new volume of the gas is 0.58 liters.