TRIPEL PYTHAGORAS - TEOREMA PYTHAGORAS (3) - MATEMATIKA SMP KELAS 8

Summary

In this educational video, Wahyudi presents an in-depth explanation of the Pythagorean Theorem and its application to Pythagorean triples. The video covers the definition of the theorem, explores how to determine Pythagorean triples using specific formulas, and provides examples to illustrate the concept. The speaker emphasizes understanding the relationships between the sides of right triangles, particularly focusing on integer solutions to the equation a² + b² = c². By walking through examples and formulas, Wahyudi makes learning about Pythagorean triples both accessible and engaging, encouraging viewers to explore this mathematical concept further.

Takeaways

• 😀 The lesson covers Pythagorean Triples, focusing on their definition and how to find them.
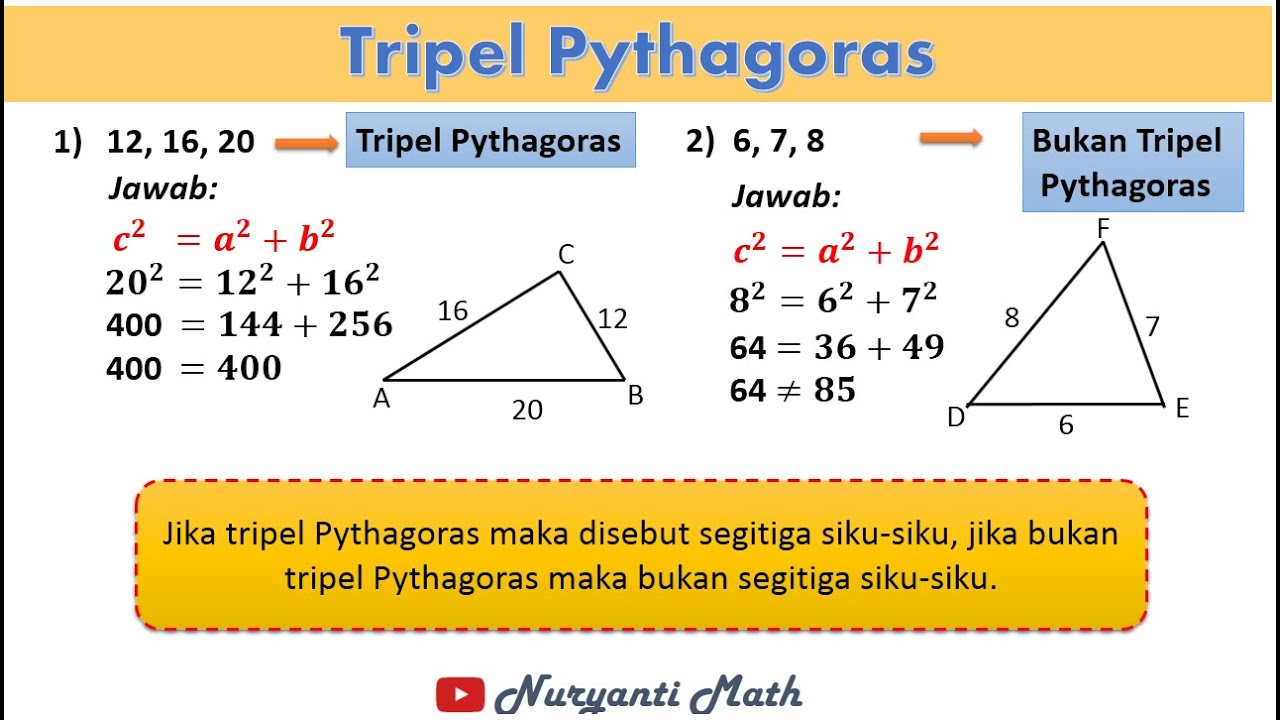
• 😀 Pythagorean Triples are sets of three positive integers (a, b, c) that satisfy the equation a² + b² = c², with c being the largest value.
• 😀 The Pythagorean Theorem is reviewed, where for a right triangle with sides a, b, and hypotenuse c, the relationship c² = a² + b² holds true.
• 😀 The script explains how to manipulate the Pythagorean Theorem equation to find the values of a, b, and c in a triangle.
• 😀 A key formula derived from the Pythagorean Theorem is a² = c² - b², which helps solve for a given c and b.
• 😀 The concept of finding Pythagorean Triples through various methods is discussed, with examples provided for better understanding.
• 😀 The video emphasizes that Pythagorean Triples can be scaled, meaning multiplying a valid Triple by a constant results in another valid Triple.
• 😀 A method to derive Pythagorean Triples by using odd numbers (starting from 3) is shown, where the first example gives the Triple (3, 4, 5).
• 😀 The video demonstrates how to calculate other Pythagorean Triples such as (5, 12, 13) and (7, 24, 25) using a systematic approach.
• 😀 The script encourages viewers to continue exploring Pythagorean Triples, noting that they are infinite and can be derived from simple formulas.

Q & A

What is the Pythagorean Theorem?

The Pythagorean Theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. Mathematically, this is expressed as C² = A² + B².

What is a Pythagorean Triple?

A Pythagorean Triple consists of three positive integers, A, B, and C, where A² + B² = C². These are sets of whole numbers that satisfy the Pythagorean Theorem, with C being the largest of the three numbers.

What is the importance of the condition a < b < c in a Pythagorean Triple?

In a Pythagorean Triple, the condition a < b < c ensures that the numbers are ordered in increasing size, where 'c' is the hypotenuse (the longest side of the triangle), and 'a' and 'b' are the legs (the other two sides). This helps in clearly identifying the largest number as the hypotenuse.

How do you find Pythagorean Triples?

Pythagorean Triples can be found using the formula where a = 2m, b = m² - 1, and c = m² + 1, where m is a positive integer greater than 1. This formula generates primitive Pythagorean Triples when m is chosen accordingly.

What is a primitive Pythagorean Triple?

A primitive Pythagorean Triple is a set of Pythagorean triples where the greatest common divisor (GCD) of the three numbers (A, B, C) is 1, meaning that they are relatively prime to each other.

Can you give an example of a Pythagorean Triple?

One well-known example of a Pythagorean Triple is (3, 4, 5), as 3² + 4² = 9 + 16 = 25, which equals 5².

How can you derive other Pythagorean Triples from a basic triple?

You can derive other Pythagorean Triples by multiplying each number in the original triple by the same factor. For example, multiplying the (3, 4, 5) triple by 2 gives (6, 8, 10), and multiplying it by 3 gives (9, 12, 15).

What happens if C - B = 1 in a Pythagorean Triple?

If C - B = 1, it leads to a formula where a² = 2B + 1. This relationship helps identify whether the resulting values for 'a' will be odd, as the square of a number close to a power of 2 often results in odd numbers.

Why is it important to choose odd values for B and C when solving for Pythagorean Triples?

Choosing odd values for B and C ensures that the resulting values for 'A' in the Pythagorean Triple formula are integers and that the triplet remains a valid solution under the Pythagorean Theorem.

What role does the variable 'm' play in generating Pythagorean Triples?

The variable 'm' is used to generate a general form for the Pythagorean Triples. By substituting different values for 'm', you can generate various Pythagorean Triples. The relations a = 2m, b = m² - 1, and c = m² + 1 hold for all valid Pythagorean Triples.