TRIGONOMETRI (5): PEMBAHASAN SOAL KONTEKSTUAL PERBANDINGAN TRIGONOMETRI ATAU APLIKASI SEHARI-HARI
Summary
TLDRIn this video, the presenter discusses trigonometry concepts, focusing on contextual problems involving elevation and depression angles. Several real-life scenarios are explored, such as calculating the height of a tree from a distance using a clinometer, determining the height of a building based on a pilot's view, and using trigonometric functions to find distances between ships and a lighthouse. Through these practical examples, viewers learn to apply trigonometric ratios like tangent to solve problems involving right triangles in various contexts.
Takeaways
- 😀 Raihan stands 20 meters away from a tree, and using a clinometer, the elevation angle is 55°. The problem involves calculating the height of the tree.
- 😀 The concept of elevation angle is explained as the angle between the horizontal line and the line pointing upwards to the top of an object.
- 😀 In the first example, the height of the tree is found by first calculating the horizontal distance from Raihan to the tree, and then adding the height of Raihan's eyes to the final tree height.
- 😀 The solution involves using trigonometric functions such as tangent, where tan(55°) is used to find the length of side BC in a right triangle.
- 😀 In the second example, a plane above a building observes the building top at a depression angle of 42°. The goal is to determine the height of the building.
- 😀 Depression angle is explained as the angle between the horizontal and the line going downward from the observer's point of view.
- 😀 To calculate the building height, the first step is to find the length of line CD, and then subtract it from the height of the plane above the building.
- 😀 The tangent function is again used for this calculation, with tan(42°) = CD/300 meters, and the building height is found by subtracting the value of CD from the total height.
- 😀 In a third example, the height of a tower is calculated using trigonometric principles from a known distance and angle of elevation from point B.
- 😀 The script also includes how to use tangent ratios to determine various unknown distances and angles in real-world geometry problems involving elevation and depression.
Q & A
What is the concept of 'elevation angle' in trigonometry?
-The elevation angle refers to the angle formed by a horizontal line and a line of sight directed upwards towards an object. It is used to measure the angle from the observer's eye level to the object above.
How do we calculate the height of a tree using trigonometry in the given scenario?
-To calculate the height of the tree, we first use the tangent of the elevation angle (55°) to find the height of the tree's top above the observer's eye level. Afterward, we add the height of the observer's eyes (1.5 meters) to get the total height of the tree.
What trigonometric ratio is used to calculate the height of the tree?
-The tangent ratio is used because the known side is the horizontal distance (adjacent side), and the unknown side is the vertical height (opposite side).
How do we calculate the height of a building when a pilot observes its peak with a depression angle of 42°?
-We use the tangent of the depression angle (42°) to calculate the distance from the building’s top to the pilot’s position. The height of the building can then be found by subtracting this distance from the total height.
What is the difference between an elevation angle and a depression angle in trigonometric problems?
-An elevation angle measures the angle from the horizontal line upward towards an object, while a depression angle measures the angle downward from the horizontal line towards an object.
How do you calculate the distance between two ships seen from a lighthouse?
-First, calculate the distance from the lighthouse to each ship using the depression angle and the tangent function. Then subtract the two distances to find the distance between the ships.
In the problem with the lighthouse and ships, why is the tangent ratio used?
-The tangent ratio is used because it relates the height of the lighthouse (opposite side) to the distance from the lighthouse to the ship (adjacent side).
What are the necessary steps to find the height of a structure using the tangent function?
-To find the height of a structure, you first need to know the horizontal distance to the structure and the elevation or depression angle. Use the tangent of the angle, multiply it by the horizontal distance, and if needed, add or subtract the observer's height.
Why is it important to know the height of the observer’s eyes in trigonometric height problems?
-The observer's eye height is important because it represents the starting point for measurements of height in problems where the observer is not standing at ground level.
In the trigonometric problems involving angles and distances, how is the tangent function applied?
-The tangent function is applied by relating the angle of elevation or depression to the ratio of the opposite side (height of an object) over the adjacent side (distance). This ratio is used to find unknown distances or heights in the problem.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

Trigonometri #14 - Sudut Elevasi dan Sudut Depresi
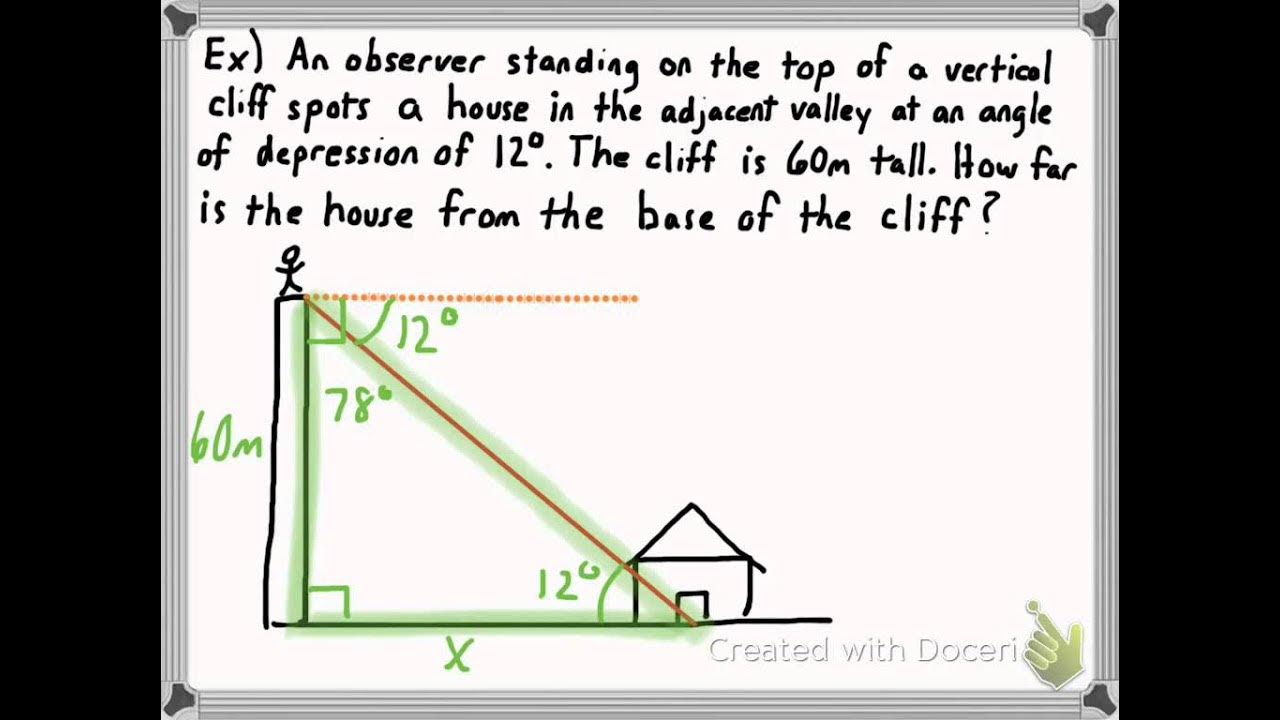
Angle of Elevation/Angle of Depression Problems
Matematika SMA - Trigonometri (2) - Aplikasi Perbandingan Trigonometri, Sudut Depresi & Elevasi (A)

Perbandingan Trigonometri Part 2- Mengukur Tinggi Benda dengan Clinometer

Perbandingan Trigonometri: Pembahasan Soal Cerita
Matematika SMA - Trigonometri (1) - Pengenalan Trigonometri, Perbandingan Trigonometri (A)
5.0 / 5 (0 votes)
