Normal stress due to axial and bending moment - Tegangan normal akibat gaya dalam momen lentur - 7

Summary

In this video, Nurazizah Andhika discusses the combination of axial and bending stresses in columns, focusing on the effect of eccentric loads. The video uses the example of a rectangular column to explain the distribution of stresses due to vertical loads and bending moments. The concept of the neutral axis is introduced, where parts of the column experience compression or tension. The video delves into calculating these stresses and the position of the neutral axis, providing valuable insights for structural design, particularly in the context of steel and concrete structures.

Takeaways

• 😀 Columns can have different cross-sectional shapes, such as circular or rectangular, which affect how they respond to axial and bending stresses.
• 😀 Eccentric loading (where the load is not applied at the center of the column's cross-section) introduces an additional bending moment, which needs to be considered along with axial loads.
• 😀 The bending moment (MW) created by eccentric loading causes one side of the column to be in compression and the other side in tension.
• 😀 The location of the neutral axis in a column is where the stress is zero, dividing the region under tension from the region under compression.
• 😀 The stress at any point on the column due to both axial load and bending moment can be calculated using the formula for normal stress, incorporating both axial and bending components.
• 😀 The 'plus-minus' sign convention is used to differentiate between compressive and tensile stresses in the column.
• 😀 The neutral axis is determined by setting the stress equal to zero and finding the balance between the tension and compression forces in the column.
• 😀 For the neutral axis, solving for the point where the stress is zero helps identify the transition between the compressive and tensile regions.
• 😀 The example discussed involves a column with a rectangular cross-section, which has dimensions of 300 mm by 150 mm, with an eccentric load applied at a distance from the centroid.
• 😀 Understanding axial and bending stress combinations is crucial for designing safe and stable structural elements like columns in both concrete and steel structures.

Q & A

What is the primary focus of this lecture?

The primary focus of this lecture is the combined effects of bending stress (tegangan lentur) and axial stress (tegangan aksial) in columns, particularly in a structural framework like a portal frame.

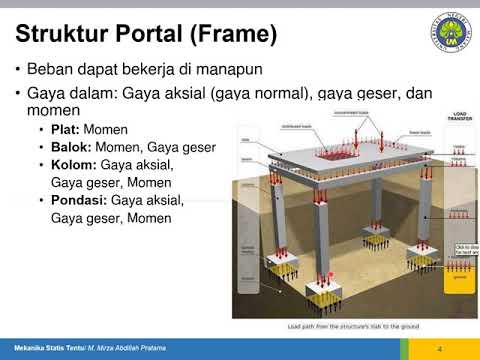
What is the structure discussed in the lecture?

The lecture discusses a portal frame structure, which consists of horizontal beams (balok) and vertical columns (kolom). The columns can have circular or rectangular cross-sections.

How is the axial and bending stress combination represented in the example?

The lecture uses a rectangular column as an example, where the axial force and bending moment work together to affect the stress distribution across the column. The bending moment causes compression on one side and tension on the opposite side.

What role does eccentricity play in the calculation of stress?

Eccentricity refers to the offset of the applied load from the centroid of the cross-section, creating a bending moment (MW). This moment affects the stress distribution in the column and is critical in determining the neutral axis.

What is the significance of the neutral axis in this context?

The neutral axis is the location in the column where there is no axial stress (neither tension nor compression). It helps determine the regions of the column that are under tension or compression due to the applied loads.

How is the location of the neutral axis determined?

The neutral axis is determined by finding the point where the combined stress (axial and bending) equals zero. This involves solving the equations for stress at different points across the cross-section and identifying where the stress changes sign.

What formula is used to calculate the stress distribution in the column?

The stress distribution is calculated using the formula that accounts for both the axial force and the bending moment, expressed as sigma = P/A ± M/W, where P is the axial load, A is the cross-sectional area, M is the bending moment, and W is the section modulus.

What is the impact of the bending moment on the column?

The bending moment causes part of the column to experience compression, while the opposite side experiences tension. The magnitude and distribution of these stresses depend on the bending moment and the column’s geometry.

How does the load position affect the stress in the column?

The position of the applied load, especially if it is eccentric (not at the centroid), creates a bending moment. This results in a non-uniform stress distribution, with some areas of the column being compressed and others under tension.

What are the practical applications of these calculations?

These calculations are critical in structural engineering, particularly in the design of steel and concrete structures. Understanding how bending and axial stresses interact allows engineers to design columns that can safely bear loads without failing.