Konversi Koordinat Cartesius ke Koordinat Kutub/ Polar dan Sebaliknya
Summary
TLDRIn this educational video, Ema Rosina Dewi explains how to read and convert coordinates between Cartesian and polar (or kutub) systems. She introduces the concept of polar coordinates using a practical example, showing how to calculate the radius (R) and angle (α) from Cartesian coordinates. The video also demonstrates how to convert between these two coordinate systems with clear, step-by-step explanations. Viewers are encouraged to learn and practice these concepts for better understanding, making this video an insightful resource for learning mathematical conversions of coordinates.
Takeaways
- 😀 The video introduces the concept of Cartesian coordinates and polar coordinates.
- 😀 Cartesian coordinates are based on the x and y axes, while polar coordinates use a radius (r) and angle (α).
- 😀 The video explains how to convert from Cartesian coordinates to polar coordinates and vice versa.
- 😀 To convert from Cartesian to polar coordinates, the radius r is calculated as √(x² + y²), and the angle α is determined using the tangent function.
- 😀 The angle α can be calculated as tan(α) = y/x, and the value of α depends on the quadrant in which the point lies.
- 😀 The polar coordinates for a point (x=2, y=2) are 2√2, 45°.
- 😀 The conversion from polar to Cartesian coordinates uses the formulas x = r * cos(α) and y = r * sin(α).
- 😀 For a point given in polar coordinates (r=2√2, α=45°), the Cartesian coordinates are (x=2, y=2).
- 😀 The video uses the example of a red point on a coordinate grid to explain these concepts clearly.
- 😀 The importance of understanding the relationship between Cartesian and polar coordinates is emphasized for solving mathematical problems.
Q & A
What is the main topic of the video?
-The main topic of the video is about understanding and converting between Cartesian coordinates and polar coordinates, specifically how to read and convert coordinates from one system to the other.
How are Cartesian coordinates represented in the video?
-Cartesian coordinates are represented as a pair of numbers (x, y), where 'x' is the horizontal distance from the origin, and 'y' is the vertical distance from the origin.
What is the purpose of converting Cartesian coordinates to polar coordinates?
-The purpose is to represent a point in terms of its distance from the origin (r) and the angle (Alpha) from the x-axis, providing a different way to describe the location of the point.
What are the elements used in polar coordinates?
-Polar coordinates are represented by two elements: 'r', the radial distance from the origin, and 'Alpha', the angle formed with the x-axis.
How is the distance 'r' calculated in polar coordinates?
-The distance 'r' is calculated using the Pythagorean theorem: r = √(x² + y²), where x and y are the Cartesian coordinates of the point.
How is the angle 'Alpha' determined in polar coordinates?
-The angle 'Alpha' is determined using the tangent function: tan(Alpha) = y/x, where x and y are the Cartesian coordinates. The angle is then calculated using the inverse tangent function.
What is the formula for converting Cartesian coordinates (x, y) to polar coordinates (r, Alpha)?
-To convert Cartesian coordinates to polar coordinates, first calculate r = √(x² + y²), then calculate Alpha = tan⁻¹(y/x), adjusting for the correct quadrant of the point.
What is the reverse process of converting polar coordinates to Cartesian coordinates?
-To convert polar coordinates to Cartesian coordinates, use the formulas x = r * cos(Alpha) and y = r * sin(Alpha), where r is the radial distance and Alpha is the angle.
What is the Cartesian coordinate for the point given as (2√2, 45°) in polar coordinates?
-The Cartesian coordinates for the point (2√2, 45°) are (2, 2), as calculated by using the formulas x = r * cos(Alpha) and y = r * sin(Alpha).
Why is it important to understand both Cartesian and polar coordinates?
-Understanding both systems is important because they provide different ways of representing and analyzing the position of points, making it easier to solve problems in various mathematical and real-world applications.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
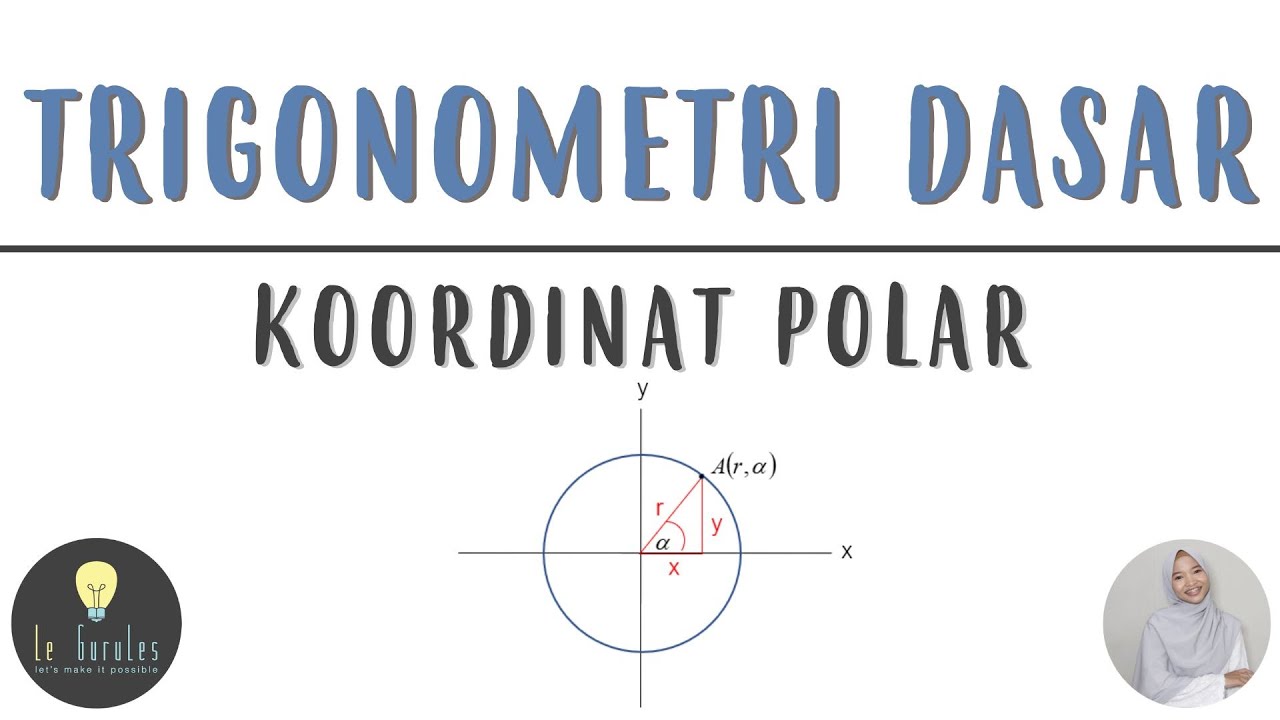
Matematika SMA - Trigonometri (6) - Koordinat Polar, Koordinat Kutub dan Kartesius (A)
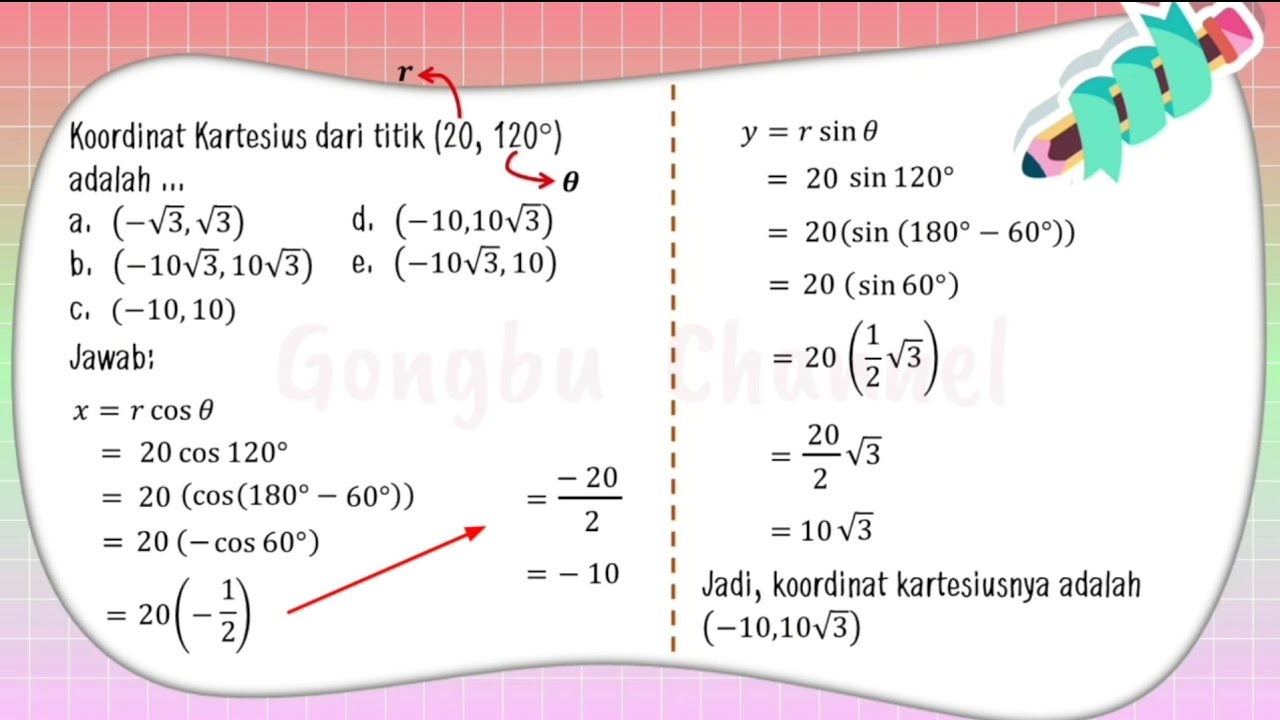
TRIGONOMETRI (Konversi Koordinat Kutub Ke Koordinat Kartesius)

Polar Coordinates Basic Introduction, Conversion to Rectangular, How to Plot Points, Negative R Valu

Coordenadas Polares ¿Qué son? EXPLICACIÓN COMPLETA

Vector Algebra (LEC -33) Polar coordinate system || Polar coordinate system || in Hindi ||
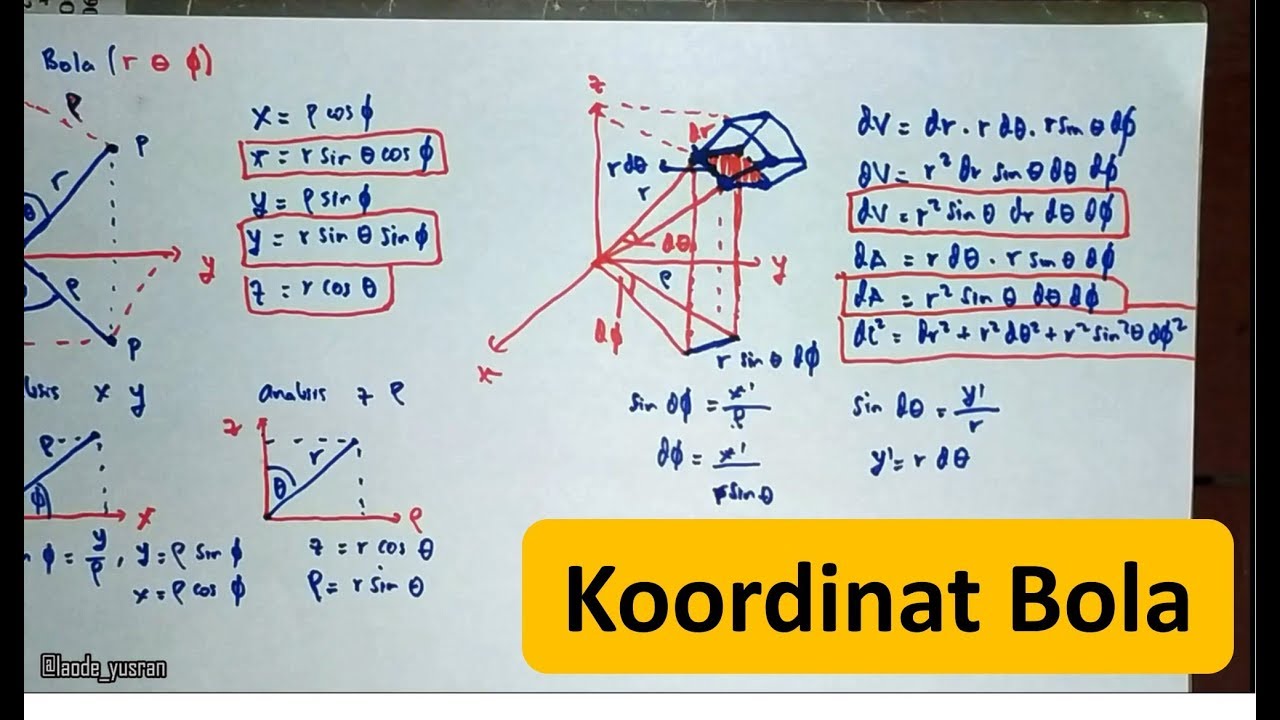
Fisika Matematika : Sistem Koordinat Bola
5.0 / 5 (0 votes)
