De qué depende el periodo para una masa en un resorte | Física | Khan Academy en Español

Summary

This video script explains the principles behind the oscillation of a mass on a spring, focusing on the relationship between amplitude, period, mass, and the spring constant. It highlights that while the amplitude affects the distance the mass moves, it does not influence the period. The period, on the other hand, is affected by the mass and the spring constant (K). A larger mass increases the period due to greater inertia, while a higher spring constant results in a faster oscillation and a shorter period. Additionally, the period remains unaffected by gravitational acceleration, making this concept applicable to both horizontal and vertical setups.

Takeaways

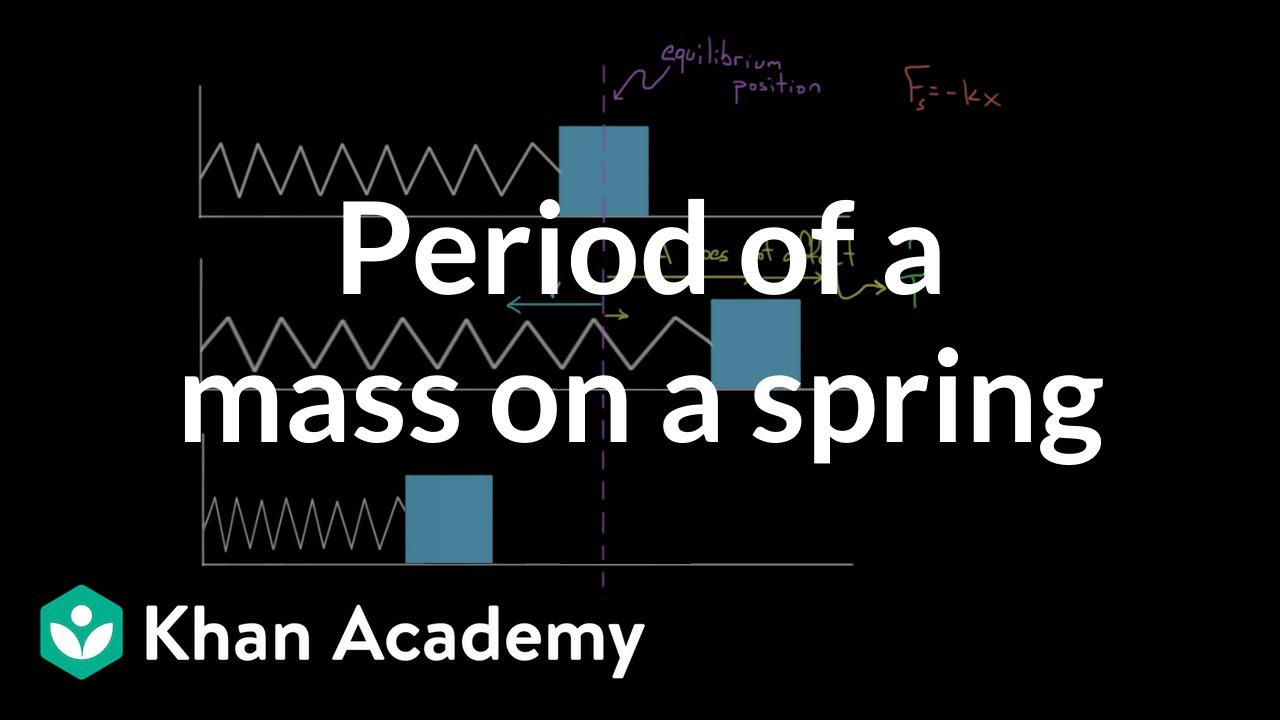
• 😀 The amplitude of oscillation is the maximum displacement of the mass from its equilibrium position.
• 😀 The period of oscillation is the time it takes for the mass to complete one full cycle.
• 😀 Amplitude does not affect the period of oscillation in a spring system.
• 😀 Although increasing the amplitude increases the distance the mass travels, it also increases the speed of the mass, balancing out the effect on the period.
• 😀 The period of oscillation is determined by the mass of the object and the spring constant, not by the amplitude.
• 😀 The formula for the period of a mass on a spring is: T = 2π√(m/K), where T is the period, m is the mass, and K is the spring constant.
• 😀 A larger mass increases the period because it has more inertia, resisting changes in motion.
• 😀 A larger spring constant (K) decreases the period by making the spring stiffer, resulting in faster oscillations.
• 😀 The period is independent of gravitational acceleration (g) and will remain the same whether the system is on Earth, Mars, or the Moon.
• 😀 This formula for the period applies to both horizontal and vertical oscillations of the mass on the spring.
• 😀 Changing the amplitude of oscillation does not affect the time it takes for the mass to complete a cycle, regardless of whether the system is horizontal or vertical.

Q & A

What is the relationship between amplitude and the period of oscillation?

The amplitude does not affect the period of oscillation. While a larger amplitude means a greater distance for the mass to travel, it also results in a higher speed due to a stronger force from the spring. These two factors balance out, and the period remains the same.

How does the mass of the object affect the period of oscillation?

The period increases with the mass. A larger mass has greater inertia, making it more resistant to changes in motion. This causes it to take more time to complete a full cycle, increasing the period.

What effect does the spring constant (k) have on the period?

The period decreases with a higher spring constant (k). A stiffer spring exerts more force on the mass, causing it to move faster, and therefore the period of the oscillation becomes shorter.

Why does the period not depend on the amplitude of the oscillation?

The period is independent of the amplitude because the increased distance to travel with a larger amplitude is compensated by the increased speed of the mass due to the greater force exerted by the spring. These two factors balance, leaving the period unchanged.

What is the formula for the period of oscillation in a mass-spring system?

The formula for the period of a mass-spring system is: T = 2π√(m/k), where 'T' is the period, 'm' is the mass, and 'k' is the spring constant.

How does gravitational acceleration affect the period of a mass-spring system?

Gravitational acceleration (g) does not affect the period of oscillation. The formula for the period works the same regardless of whether the system is on Earth, Mars, or the Moon.

What would happen to the period if the mass were increased?

If the mass is increased, the period will increase. A larger mass has more inertia, which makes it harder to change its motion, leading to a longer time to complete an oscillation.

How would changing the spring constant (k) affect the period?

Increasing the spring constant (k) would make the spring stiffer, increasing the force exerted on the mass. This would cause the mass to move more quickly, reducing the period of the oscillation.

Does the period change when the amplitude is varied?

No, the period does not change with variations in amplitude. The oscillation will still take the same amount of time to complete a full cycle regardless of how far the mass is displaced from the equilibrium position.

Can the period of oscillation be the same for horizontal and vertical mass-spring systems?

Yes, the period of oscillation is the same for both horizontal and vertical systems as long as the mass and spring constant remain unchanged. The formula for the period applies to both setups, and gravitational acceleration does not influence the period.