[MEKANIKA BAHAN] PERTEMUAN-17 : "DEFORMASI METODE DOUBLE INTEGRAL PART-1" OLEH Dr. JANUARTI J. E.

Summary

This video focuses on the topic of deformation in structures, specifically bending deformation in beams. The speaker introduces methods for calculating changes in shape due to applied loads, explaining how a beam may bend either upward or downward. Key concepts include the elastic curve, curvature radius, and the relationship between moment, strain, and deformation. The speaker also elaborates on the mathematical relationships and formulas used to determine strain, stress, and curvature in structural beams, highlighting the importance of these calculations in structural analysis.

Takeaways

• 😀 The script covers the deformation of beams under loading, focusing on how beams bend and change shape.
• 😀 The Elastic Curve method is introduced to calculate the deformation in beams, which shows how the shape changes under applied forces.
• 😀 Curvature (ρ) is discussed as a key concept in beam deformation, and the radius of curvature is essential for understanding beam bending.
• 😀 The relationship between the initial length of the beam and the deformed length is explained, with an example of how the beam elongates or contracts.
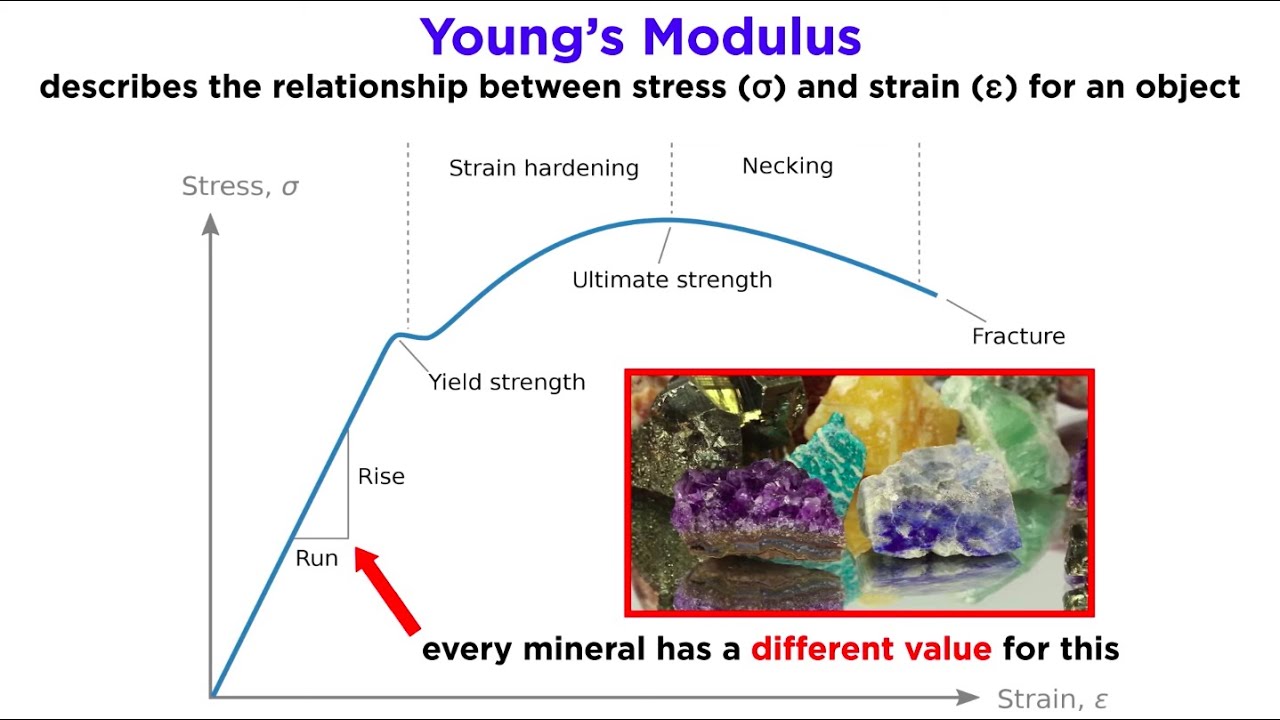
• 😀 Key equations are derived to express the relationship between elongation (ΔL), modulus of elasticity, and the applied bending moment.
• 😀 The lecturer explains how strain is calculated as the ratio of elongation to the original length of the beam.
• 😀 The concept of stress and strain is tied to the modulus of elasticity and bending moment, with a focus on how these factors influence beam deformation.
• 😀 A practical example of a uniformly loaded beam is presented to show how deformation calculations are applied in real-world situations.
• 😀 The script explains how small sections of the beam are analyzed to understand the overall deformation, with a focus on small changes in angle (Δθ).
• 😀 Mathematical derivations are provided to find the vertical displacement of a beam as a function of its position and applied moments, with integration being a key method for solving these equations.

Q & A

What is the main topic of the lecture?

The lecture focuses on deformation in beams under load, specifically addressing bending, shear forces, torsion, and the methods to calculate these deformations, such as the elastic curve method.

What is the elastic curve method used for in this context?

The elastic curve method is used to determine the deformation of a beam when subjected to various loads. It helps calculate the deflection and curvature of the beam by analyzing the bending and torsion effects.

How does the bending of a beam occur under load?

When a beam is subjected to a load, it deforms, typically bending in one direction. This can result in a curve either bending upwards or downwards, depending on the type and direction of the load applied.

What is the significance of the term 'radius of curvature' in beam deformation?

The radius of curvature refers to the radius of the curve formed by the beam when it deforms. It is an important parameter in calculating the extent of bending or deformation in the beam.

What role do shear forces and torsion play in beam deformation?

Shear forces and torsion contribute to the internal stresses within the beam, leading to deformation. Shear forces cause bending and twisting, while torsion specifically induces rotational deformation along the beam’s axis.

What is the relationship between strain and stress in beam deformation?

Strain is the measure of deformation, calculated as the change in length relative to the original length. Stress is the internal force per unit area within the material, which causes deformation. The relationship is given by the formula strain = stress / modulus of elasticity.

How is the elongation of the beam calculated during deformation?

Elongation is calculated by observing the change in length at different points along the beam. This can be determined through geometric relationships and using the concept of strain, which relates the change in length to the radius of curvature and applied forces.

What is the formula for calculating the bending moment in the beam?

The bending moment is typically calculated using the formula M = σ * y, where M is the moment, σ is the stress, and y is the distance from the neutral axis to the point where the stress is applied. This helps determine how the beam bends under load.

What does the lecture suggest about calculating the curvature of a beam?

The curvature of a beam is calculated using the relationship between the bending moment and the radius of curvature. The formula involves the second derivative of the displacement function and the beam’s moment of inertia.

What is the connection between the deflection and the moment in a beam?

The deflection of a beam is related to the bending moment through the equation that links the moment to the curvature of the beam. The larger the bending moment, the greater the deflection. This is often calculated using integration methods to derive the beam’s displacement function.