Ideia por trás da inclinação de uma reta
Summary
TLDRIn this educational video, the presenter explains how to derive the equation of a line given its slope and a point on the line. Starting from the basic definition of slope, the video illustrates how to create the equation using the coordinates of a known point and an arbitrary point on the line. The fundamental equation of the line is derived and simplified, showcasing its practical application with a specific example. The presenter also demonstrates how to convert this equation into slope-intercept form, making it easier to understand the relationship between slope and y-intercept.
Takeaways
- 📏 The slope (m) of a line can be determined using points on the line, specifically through a known point (a, b).
- 📊 The equation of a line can be constructed by relating the slope between any point (x, y) and the known point (a, b).
- 🔼 The change in y (Δy) is calculated as the difference between the arbitrary y-value and the y-value of the known point.
- 🔽 Similarly, the change in x (Δx) is determined by subtracting the x-value of the known point from the arbitrary x-value.
- ✍️ The relationship established by the slope can be represented in an equation form: (y - b) = m(x - a).
- 📐 This equation is referred to as the fundamental equation of the line and is a foundational concept in linear algebra.
- 🔗 The fundamental equation can be rewritten using specific values for the slope and a point to express the equation in standard form.
- 🧮 An example demonstrates how to use the slope and a point to derive the line's equation effectively.
- 🛠️ The equation can be further transformed into slope-intercept form (y = mx + b), which is often easier to analyze.
- 📈 Understanding both forms of the line's equation allows for better interpretation of linear relationships in mathematical contexts.
Q & A
What is the significance of the slope 'm' in the context of the line described in the transcript?
-'m' represents the slope of the line, indicating its steepness and direction. A higher value of 'm' means a steeper incline.
How is the slope between two points on a line calculated?
-The slope is calculated as the change in 'y' (vertical change) over the change in 'x' (horizontal change), expressed mathematically as (y - b) / (x - a) for points (a, b) and (x, y).
What geometric concept does the term 'delta' represent in the context of the transcript?
-'Delta' refers to the difference or change in values, represented as 'Δy' for change in y and 'Δx' for change in x.
What is the fundamental equation of a line as derived in the transcript?
-The fundamental equation of the line is y - b = m(x - a), which relates any point (x, y) on the line to the known point (a, b) and the slope 'm'.
How can the fundamental equation of the line be simplified?
-To simplify, one can multiply both sides by (x - a) to eliminate the denominator, resulting in the equation y - b = m(x - a).
Why is the equation y - b = m(x - a) referred to as the fundamental equation of the line?
-It is called the fundamental equation because it provides a straightforward way to analyze and determine if a point lies on the line based on its slope and a point on the line.
How do you derive the slope-intercept form from the fundamental equation?
-To derive the slope-intercept form, you distribute 'm' and then isolate 'y', resulting in the form y = mx + c, where 'c' is the y-intercept.
What example is provided in the transcript to illustrate the use of the fundamental equation?
-The example involves a line with a slope of 2 passing through the point (-7, 5), which is used to create the equation y - 5 = 2(x + 7).
What is the final slope-intercept form derived from the given example?
-The final slope-intercept form derived from the example is y = 2x + 19, indicating the slope and the y-intercept.
What different forms can the equation of a line take according to the transcript?
-The equation of a line can be expressed in various forms, including the fundamental equation y - b = m(x - a) and the slope-intercept form y = mx + c.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

Introduction to point-slope form | Algebra I | Khan Academy
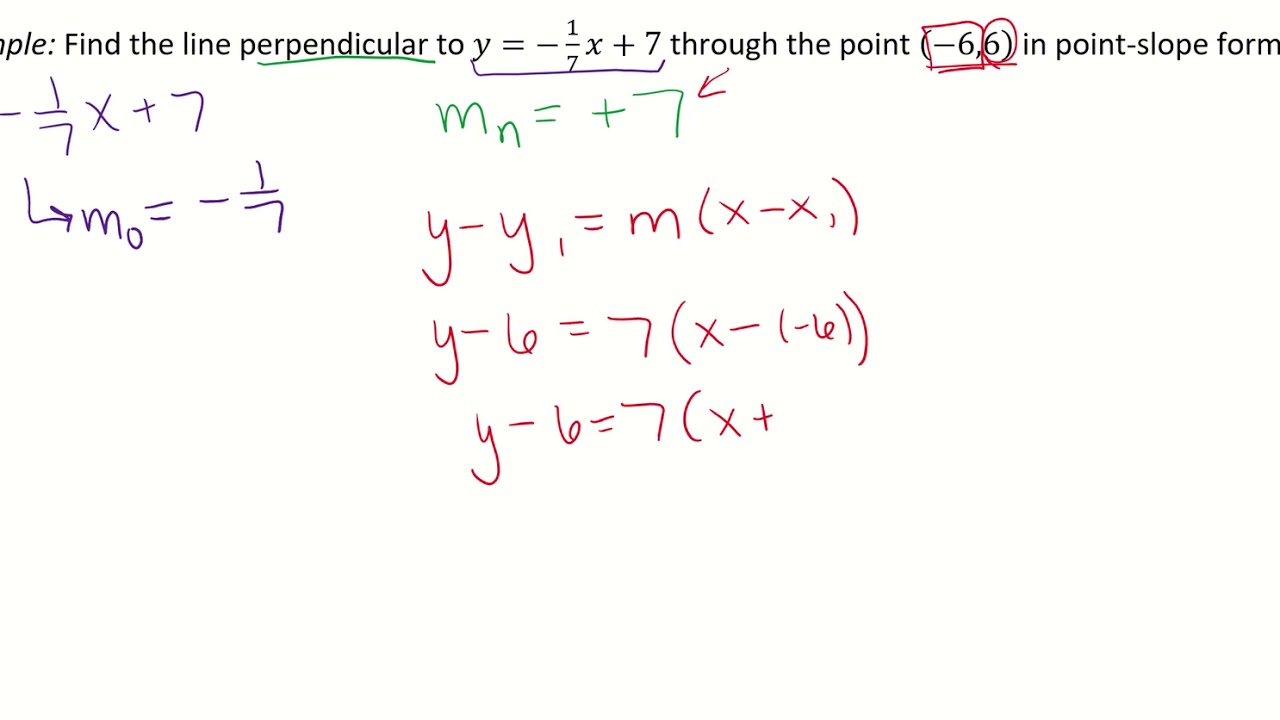
Lesson 4-1, Video 5; Perpendicular Line 2

Linear Functions

Pengertian Kemiringan Hal 235-238 Bab 5 Persamaan Garis Lurus Kelas 8 Kurikulum Merdeka Belajar

Persamaan Garis Lurus (2) | Gradien Garis | Matematika Kelas 8

Geometria analítica - Inclinação e coeficiente angular Equação da reta sabendo ponto e a declividade
5.0 / 5 (0 votes)
