Proving Trigonometric Identities (Tagalog/Flipino Math)
Summary
TLDRThis video script delves into trigonometric identities, explaining how they are equations true for all variable values. It covers reciprocal, Pythagorean, and quotient identities, illustrating how they relate to trigonometric functions. The script provides examples of expressing trigonometric functions in terms of others, like sine and cosine, and demonstrates proving identities by simplifying expressions using fundamental trigonometric relationships. It's a comprehensive tutorial aimed at enhancing understanding of trigonometric identities.
Takeaways
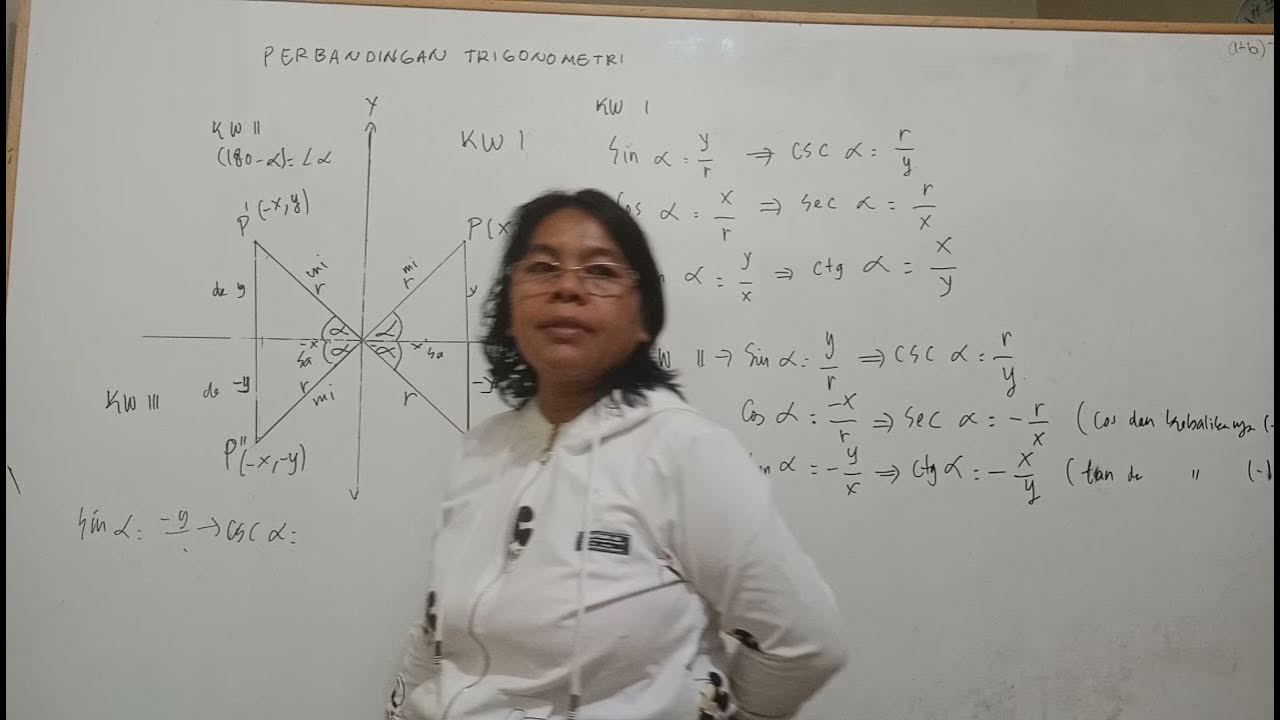
- 📚 Trigonometric identities are equations true for all variable values with finite definitions.
- 🔄 Reciprocal trigonometric functions include sine, cosine, tangent, and their counterparts (cosecant, secant, and cotangent).
- 📐 Pythagorean identities are key in trigonometry, such as sin²θ + cos²θ = 1 and 1 + tan²θ = sec²θ.
- 🔗 Ratio identities define relationships between trigonometric functions, like tan(θ) = sin(θ)/cos(θ) and cot(θ) = cos(θ)/sin(θ).
- 🔄 Expressions can be rewritten using fundamental identities to simplify complex trigonometric equations.
- 💡 To prove trigonometric identities, substitute proper definitions and reduce expressions to simpler forms.
- ✔️ It is useful to reduce the more complex side of an identity to match the simpler side for easier verification.
- ⚠️ Avoid introducing radical expressions unless necessary when proving identities.
- 📝 Simplifying complex trigonometric expressions is often done by converting them into sine and cosine functions.
- 📊 Use fundamental identities like reciprocal, Pythagorean, and ratio identities to prove and simplify trigonometric equations.
Q & A
What is a trigonometric identity?
-A trigonometric identity is an equation that holds true for all values of the variables for which both sides of the equation have defined finite values. It expresses a relationship between trigonometric functions.
What are the reciprocal trigonometric identities?
-The reciprocal trigonometric identities are: sine θ = 1/cosecant θ, cosine θ = 1/secant θ, and tangent θ = 1/cotangent θ. These identities relate basic trigonometric functions to their reciprocals.
What are the basic Pythagorean trigonometric identities?
-The basic Pythagorean identities are: sine²θ + cosine²θ = 1, 1 + tangent²θ = secant²θ, and 1 + cotangent²θ = cosecant²θ. These are derived using the Pythagorean Theorem.
How can sine θ be expressed in terms of cosine θ?
-Using the Pythagorean identity, sine²θ + cosine²θ = 1, we can rearrange it to sine²θ = 1 - cosine²θ. Taking the square root gives sine θ = ±√(1 - cosine²θ).
How can tangent θ be expressed in terms of sine θ and cosine θ?
-Tangent θ can be expressed as sine θ / cosine θ. If sine θ is written in terms of cosine θ, the expression for tangent θ becomes ±√(1 - cosine²θ) / cosine θ.
What is secant θ in terms of cosine θ?
-Secant θ is the reciprocal of cosine θ, so secant θ = 1 / cosine θ.
What is cotangent θ in terms of secant θ?
-Using the identity 1 + tangent²θ = secant²θ, we can express cotangent θ as 1 / tangent θ, which is equal to 1 / ±√(secant²θ - 1).
How can cosine θ be expressed in terms of cosecant θ?
-Cosine θ can be expressed in terms of sine θ using the identity cosine²θ = 1 - sine²θ. Since sine θ = 1 / cosecant θ, cosine θ becomes ±√(1 - (1 / cosecant²θ)).
What is a general approach to proving trigonometric identities?
-A general approach to proving trigonometric identities is to substitute known trigonometric definitions or relations, simplify both sides of the equation, and reduce complex expressions into simpler forms.
How can secant²θ + tangent²θ be simplified?
-Secant²θ + tangent²θ can be simplified using the Pythagorean identity secant²θ = 1 + tangent²θ. This allows for further manipulation when simplifying expressions.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级5.0 / 5 (0 votes)