ap13.1 Describing oscillations
Summary
TLDRThis video covers key concepts of periodic motion in AP Physics, focusing on oscillations. It explains that oscillations occur due to a restoring force and defines critical terms like amplitude, period, frequency, and angular frequency. The lesson introduces Hooke’s Law to describe the net force on an oscillating body and demonstrates the relationship between displacement, velocity, and acceleration. The video also explains how angular frequency is calculated and its relationship with frequency and period, using examples such as an ultrasonic transducer. The session concludes with a summary of these foundational principles of oscillatory motion.
Takeaways
- 📍 All oscillations have a stable equilibrium position.
- 🔄 Oscillation can only occur when there is a restoring force acting on the body.
- 📊 Key terms used to describe oscillations include amplitude, period, frequency, and angular frequency.
- 🕰 Periodic motion refers to any motion that repeats itself over time, such as a pendulum or an object oscillating on a spring.
- 📏 The displacement of an object during oscillation is always measured relative to the equilibrium position.
- ⚖ The net force in oscillations follows Hooke's Law: F = -kx, where force and displacement are always in opposite directions.
- 📈 Amplitude is the maximum displacement from the equilibrium position, measured in meters.
- ⏱ The period of an oscillation is the time it takes for one complete cycle, measured in seconds.
- 🔄 Frequency is the number of cycles per unit of time, measured in Hertz (Hz), and is the inverse of the period.
- ⏳ Angular frequency (ω) is related to the frequency by the formula ω = 2πf, measured in radians per second.
Q & A
What is the definition of periodic motion?
-Periodic motion is any kind of motion that repeats itself over and over.
What are two examples of periodic motion mentioned in the video?
-The two examples of periodic motion mentioned are a pendulum and an object oscillating on a spring.
What is a stable equilibrium position in the context of oscillations?
-A stable equilibrium position is a point where a body tends to return after being displaced, due to a restoring force.
What is the role of a restoring force in oscillations?
-A restoring force is what pulls a body back to its equilibrium position whenever it is displaced, enabling oscillation.
How is displacement described in an oscillating system?
-Displacement is described relative to the equilibrium position, where the origin (x = 0) represents the stable equilibrium.
What does Hooke's Law state about the force on an oscillating object?
-According to Hooke's Law, the net force (F) on an oscillating object is proportional to its displacement (x), with F = -kx, where k is the spring constant.
How are force and acceleration related to displacement in oscillations?
-Force and acceleration are always in the opposite direction to displacement in oscillations.
What is amplitude in the context of oscillations?
-Amplitude is the maximum magnitude of displacement from the equilibrium position, typically measured in meters.
What is the relationship between period and frequency in oscillations?
-The period (T) is the time for one complete cycle, and frequency (F) is the number of cycles per unit time. They are inversely related: F = 1/T.
What is angular frequency and how is it related to frequency?
-Angular frequency (ω) is defined as 2π times the frequency (F), with ω = 2πF. It is measured in radians per second.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
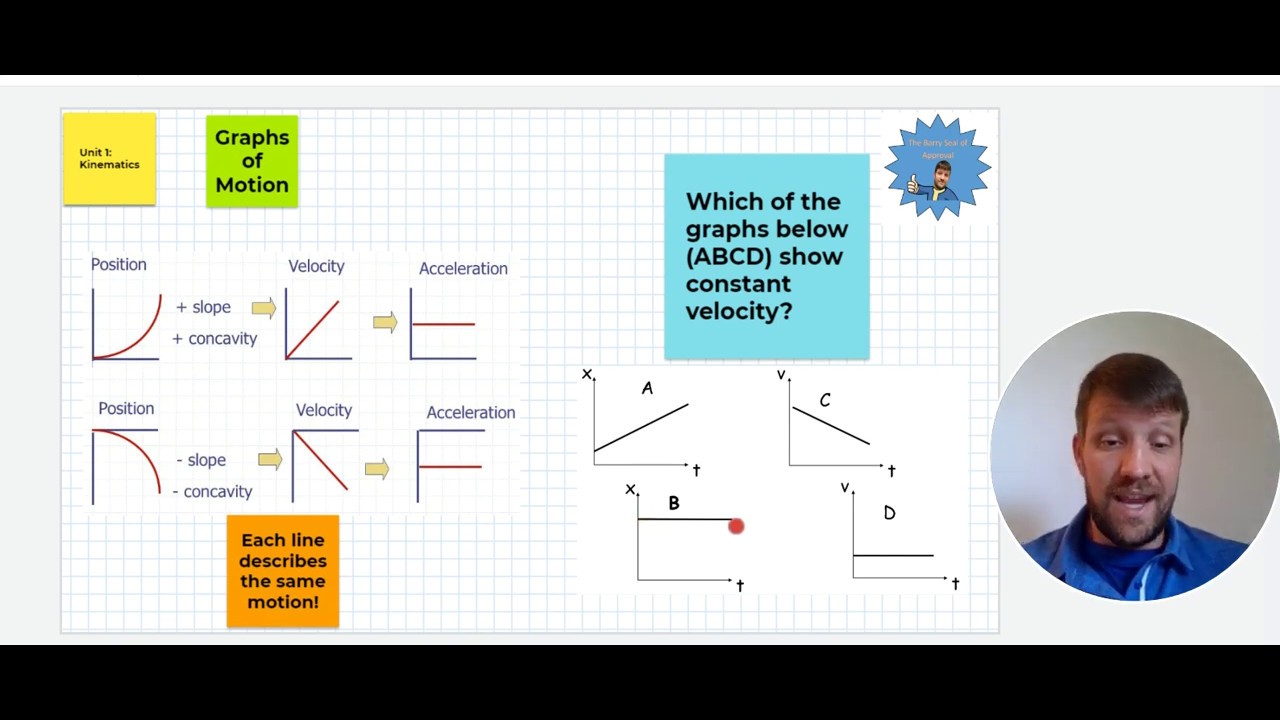
AP Physics 1 - Unit 1 Summary - Kinematics

(New) AP Physics 1 - Unit 1 Review - Kinematics - Exam Prep
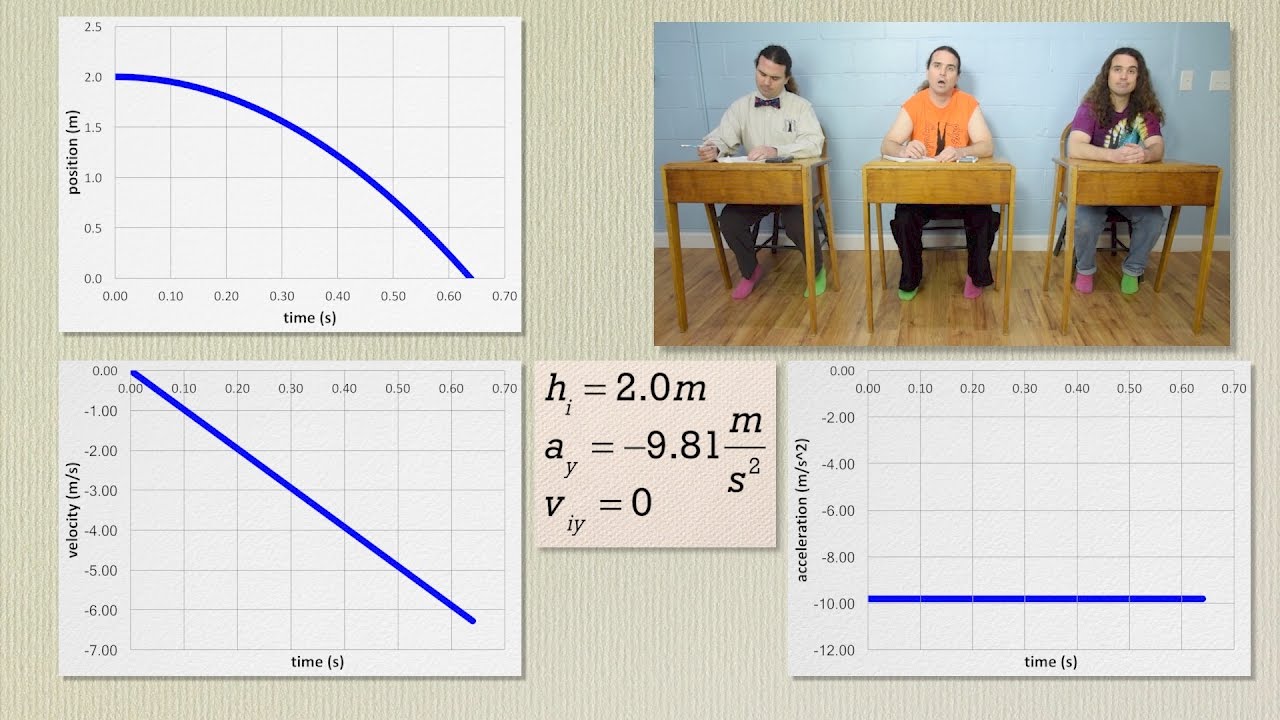
AP Physics C: Kinematics Review (Mechanics)

Gerak Harmonik Sederhana • Part 1: Konsep & Persamaan Simpangan Getaran Harmonis

Ayunan sederhana yang digantungkan pada langit-langit lift yang diam memiliki periode ayunan T.

Me Salva! CIN11 - MCU
5.0 / 5 (0 votes)
