Teorema Pythagoras Kelas 8 Semester 2
Summary
TLDRThis educational video script discusses the Pythagorean theorem for 8th-grade students. It explains the theorem's application in determining the type of triangle (acute, right, or obtuse) using side lengths. The script also covers the proof of the theorem using geometric illustrations and introduces the concept of Pythagorean triples. Practical problems, such as calculating the length of a ladder and solving for unknowns in triangles, are solved using the theorem.
Takeaways
- 📐 **Pythagorean Theorem**: The video discusses the Pythagorean Theorem, a fundamental principle in mathematics that describes the relationship between the sides of a right triangle.
- 🔢 **Triangle Classification**: It explains how to classify triangles based on the lengths of their sides, into obtuse, right, or acute triangles.
- 📚 **Concept of Triangles**: The script introduces the concept of triangles based on their angles, dividing them into three categories: acute, right, and obtuse.
- 📐 **Application of Pythagorean Theorem**: The video demonstrates how to apply the Pythagorean Theorem to determine the type of triangle given the lengths of its sides.
- 📐 **Example Calculation**: It provides an example of calculating whether a triangle with sides 2, 3, and 4 is obtuse, right, or acute.
- 📐 **Proof of Pythagorean Theorem**: The script includes a visual proof of the Pythagorean Theorem using a square divided into four right triangles and rearranged to form two squares.
- 🔢 **Triple Pythagoras**: It introduces the concept of Triple Pythagoras, which are sets of three numbers that satisfy the Pythagorean Theorem.
- 📚 **Verification of Triple Pythagoras**: The video shows how to verify a set of numbers as a Triple Pythagoras by checking if they satisfy the theorem.
- 📐 **Practical Application**: It demonstrates the practical application of the Pythagorean Theorem to solve real-world problems, such as finding the length of a ladder.
- 🔢 **Problem Solving**: The script includes problem-solving exercises that apply the Pythagorean Theorem to find unknown side lengths in triangles.
- 📚 **Final Problem**: The video concludes with a complex problem that uses the Pythagorean Theorem to find the value of 'Q' in a geometric setup.
Q & A
What is the main topic discussed in the video?
-The main topic discussed in the video is the Pythagorean Theorem, specifically for 8th-grade middle school mathematics.
What are the three types of triangles based on the size of their angles?
-The three types of triangles based on the size of their angles are acute, right, and obtuse triangles.
What is the condition for a triangle to be an acute triangle?
-A triangle is an acute triangle if the square of its largest angle (C) is less than the sum of the squares of the other two sides (a and b).
How is a right triangle defined according to the Pythagorean Theorem?
-A right triangle is defined as having the square of the length of its hypotenuse (C) equal to the sum of the squares of the lengths of the other two sides (a and b).
What is the condition for a triangle to be an obtuse triangle?
-An obtuse triangle is one where the square of its largest angle (C) is greater than the sum of the squares of the other two sides (a and b).
What is the Pythagorean Theorem?
-The Pythagorean Theorem is a principle that states, in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
How is the Pythagorean Theorem proven in the video?
-The Pythagorean Theorem is proven in the video by illustrating how four identical right triangles can be arranged to form a square, with the area of the square being equal to the sum of the areas of the individual triangles.
What is a Pythagorean Triple?
-A Pythagorean Triple consists of three positive integers that satisfy the Pythagorean Theorem, meaning that the sum of the squares of the two smaller integers is equal to the square of the largest integer.
How is the Pythagorean Theorem applied in the second exercise of the video?
-In the second exercise, the Pythagorean Theorem is applied to calculate the length of a ladder leaning against a wall, given the height of the wall and the distance from the wall to the foot of the ladder.
What is the solution to the third problem in the video involving the value of 'a'?
-The solution to the third problem involves rearranging the Pythagorean Theorem to solve for 'a', which results in 'a' being equal to 12 after simplifying the equation.
How is the value of 'Q' determined in the final problem of the video?
-The value of 'Q' in the final problem is determined by applying the Pythagorean Theorem to a series of triangles and solving the resulting equation, which leads to 'Q' being equal to 12 cm.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

teorema pythagoras kelas 8 "bagian 4" (mudah): menentukan perbandingan sisi-sisi segitiga siku-siku
Grade 10 Math: Drawing geometric figures on a Cartesian plane

COMO HALLAR O CALCULAR LA GENERATRIZ DE UN CONO TRUNCADO
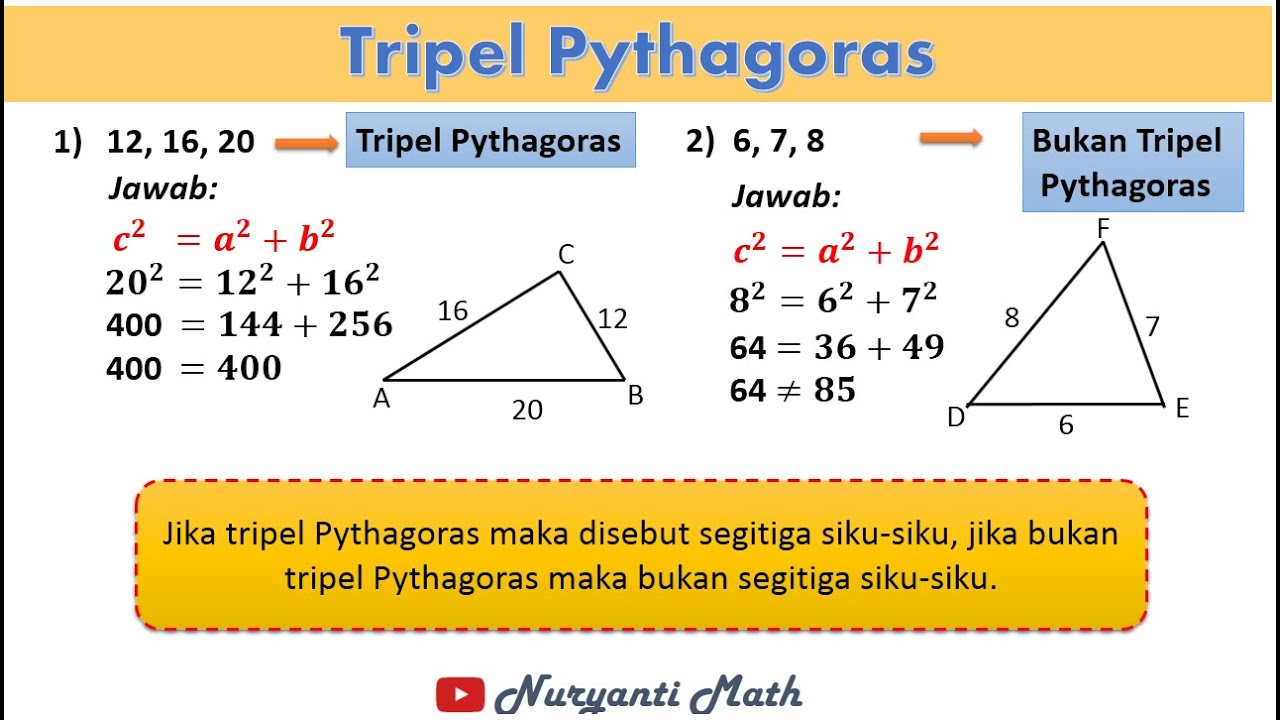
Tripel Pythagoras, Menentukan Jenis Segitiga - Matematika Kelas 8 SMP/MTs

TEOREMA PYTHAGORAS : MATEMATIKA KELAS 8 SMP

TRIPEL PYTHAGORAS - TEOREMA PYTHAGORAS (3) - MATEMATIKA SMP KELAS 8
5.0 / 5 (0 votes)
